How is energy conserved in electrical circuits without considering the energy stored in the magnetic field of the wires in the circuit?

Question

It is a well known fact that an inductor or a solenoid stores energy in the magnetic field around it. Suppose that the solenoid is unwound into a straight current carrying conductor. Firstly, is energy stored in the magnetic field around the straight wire? Secondly, if it does store energy, can the energy density be given by ,?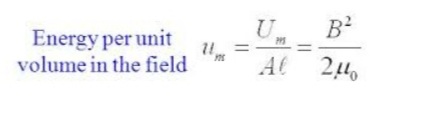

---

Considering a simple cicuit consisting of a resitor with resistance R and a battery with emf ε , then under ideal conditions, power delivered by the battery is equal to the energy dissipated as heat by resistor. $$H = i^2Rt$$ If some energy is also stored in the magnetic field around the wire, how is the above equation valid supposing that the energy stored in the magnetic field is from the energy supplied by the battery? more specifically , How is energy conserved in this case? why wouldn't be $$E_{\text{delivered by battery}} = i^2Rt + \text{Energy stored in the magnetic field} ?$$

---

Please point me out and explain where I had got the thing wrong. (By the way , this is my first question on Physics Stack Exchange)

Answer 1

Considering a simple cicuit consisting of a resitor with resistance R and a battery with emf ε , then under ideal conditions, power delivered by the battery is equal to the energy dissipated as heat by resistor. ... If some energy is also stored in the magnetic field around the wire, how is the above equation valid

This is a DC circuit. After it is hooked up and allowed to come to steady state, the current remains constant (in the ideal case) until the end of time.

That means that the energy stored in the magnetic field remains constant. The battery doesn't need to constantly supply energy to keep the magnetic field "charged".

Therefore the magnetic field and its stored energy can be ignored when analyzing the DC steady-state solution to this circuit. You would likely need to consider the magnetic energy if you wanted to know how the circuit behaves in the short time period (maybe a few or a few hundred nanoseconds) after the battery is connected.

why wouldn't be $$E_{\text{delivered by battery}} = i^2Rt + \text{Energy stored in the magnetic field} ?$$

This is mixing up two things: Energy consumed over time by the resistor, which must be continuously supplied by the battery to maintain the current; and energy stored in the magnetic field, which remains constant after the circuit is initially set up, and doesn't need to be supplied to maintain the current after the initial start up.

You should also make some guesses about the dimensions of this circuit and calculate how much magnetic energy is involved. You will find that for reasonable assumptions about the length of the wire and the area of the loop it forms with the return path, the magnetic stored energy is quite small compared to the energy consumed by the resistor in a time period over a microsecond or so.

Answer 2

Leave out the resistor, just connect a loop of wire to the battery (assumed to be perfectly conductive). That loop has an inductance. Even though there's no resistance, there isn't infinite current. Instead, the current rises according to $\frac{dI}{dt} =E/L$, where $E$ is the battery voltage and $L$ is the inductance. The battery thus transfers energy to the magnetic field, without limit in this idealization.

If you put in a resistor, the steady state of the current is given by Ohm's law, but when you first connect the battery, the initial rise of the current reflects energy storage in the magnetic field. The steady state reflects energy dissipated in the resistor.