2
$\begingroup$

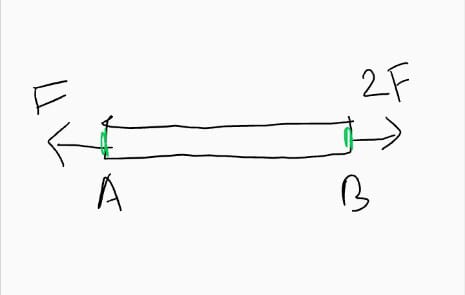
Consider an elastic rod (mass m, length l) being acted by external forces as shownenter image description here

What would be the tension at the end A and B(marked as green)? And why?

My teacher took the tensions at A to be F and at B to be 2F, but isn't the element at the end A accelerated in the forward direction so it should have tension more than F. Similarly, tension at B should be less than 2F ?

Improve this question
$\endgroup$
11
  • 1
    $\begingroup$ What affect do you acceleration has on tension? The tension is the cause of acceleration, not vice versa. $\endgroup$
    – Darth Vader
    Commented Dec 23, 2023 at 5:47
  • $\begingroup$ Also since the rod is elastic(rigid), all the particles on it will have same acceleration. $\endgroup$
    – Darth Vader
    Commented Dec 23, 2023 at 5:49
  • $\begingroup$ i agree, for the element at the end to accelerate, it must have some net forward force, so tension should be different than the forces applied $\endgroup$
    – PinkAura
    Commented Dec 23, 2023 at 5:59
  • 1
    $\begingroup$ So what should the tensions be at the ends? Have you drawn some free body diagrams to figure it out? What have you tried? $\endgroup$
    – Puk
    Commented Dec 23, 2023 at 6:50
  • 1
    $\begingroup$ @PinkAura The tension at end B is $2F$. What you're talking about is: If I take a teeny tiny element at end B of mass $dm$ and length $dx$ (like a slice from a loaf of bread), then the tension towards the right will be $2F-adm$, and towards the left it'll be$2F$. $\endgroup$
    – Darth Vader
    Commented Dec 23, 2023 at 8:06

2 Answers 2

Reset to default
2
$\begingroup$

Your teacher is correct. Because the rod has non-zero mass, tension will vary along the rod from $F$ at end A to $2F$ at end B.

If we consider a small element of the rod with length $\delta x$ and mass $\delta m$ then the difference $\delta T$ between the tensions acting to the right and to the left on that element $\delta m$ must be sufficient to accelerate it with an acceleration of $\frac F m$ which is the acceleration of the whole rod. So

$\displaystyle \delta T = \frac F m \delta m$

If we assume that the rod has the same cross sectional area $A$ and the same density $\rho$ along its whole length then

$\delta m = \rho A \delta x \\ \displaystyle \Rightarrow \delta T = \frac F m \rho A \delta x$

But the whole mass of the rod $m$ is equal to $\rho A l$, so

$\displaystyle \delta T = \frac F l \delta x$

In the limit as $\delta T$ and $\delta x$ become small we have

$\displaystyle \frac {dT}{dx} = \frac F l$

and since $\frac F l$ is a constant, we can see that $T$ must vary linearly along the rod. We know that $T=F$ at end A and $T=2F$ at end B, so at a distance $x$ from end A we have

$\displaystyle T(x) = F + \frac F l x = F \left( \frac {l+x} l \right)$

Improve this answer
$\endgroup$
6
  • $\begingroup$ Just a thought,- shouldn't we calculate integral of $\int_x^{x+k} \frac {F}{l} dl$ to get tension between $x$ and $x+k$ ? In this case answer would be $T(x,k) = F \ln \left(\frac {x+k}{x} \right)$, i.e., thus it's not linear for every practical purpose, because we can't poke physically at $x$ in rod, just at some $dx$ range ? $\endgroup$
    – Agnius Vasiliauskas
    Commented Dec 23, 2023 at 12:32
  • 1
    $\begingroup$ @AgniusVasiliauskas That's my fault for bad notation - I was using $\delta l$ for the length of a small element and using $l$ for the fixed length of the whole rod. I have edited my answer, replacing $\delta l$ with $\delta x$. $\endgroup$
    – gandalf61
    Commented Dec 23, 2023 at 12:56
  • $\begingroup$ @gandalf61 "If we consider a small element of the rod with length 𝛿𝑥 and mass 𝛿𝑚 then the difference 𝛿𝑇 between the tensions acting to the right and to the left on that element 𝛿𝑚 must be sufficient to accelerate it with an acceleration of 𝐹𝑚 which is the acceleration of the whole rod" Is it because the difference is very small in comparison to F and 2F so we neglected it at the two ends while taking the limits? $\endgroup$
    – PinkAura
    Commented Dec 24, 2023 at 12:49
  • $\begingroup$ @PinkAura No, you can still apply this argument to the ends of the rod. The leftmost element at end A of the rod experience a force $F$ to the left and a force $F+\delta T$ to the right, and the difference between these forces, $\delta T$, must be sufficient to accelerate the element with an acceleration of $\frac F m$. $\endgroup$
    – gandalf61
    Commented Dec 24, 2023 at 12:59
  • 1
    $\begingroup$ @PinkAura Yes, you are right if the element size greater than zero. But in the limit, as $\delta x \rightarrow 0$ then $\delta T \rightarrow 0$ as well and the tensions at the very ends of the rod are $F$ and $2F$. $\endgroup$
    – gandalf61
    Commented Dec 24, 2023 at 14:32
-1
$\begingroup$

image1

Let's consider end A and end B are two objects with same mass.

  1. 2F-F=2ma (both end A and end B have the same mass and system moving to the right)

  2. T-F=ma (force act on end A when the system moving to the right)

then we can obtain F=2(T-F)

F=2T-2F

3F=2T

T=3F/2

so the tension is more than F but less than 2F F<T<2F

correct me if I am wrong, hope this help!

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Your error is that the mass of the rod is distributed along its whole length, not concentrated at its two ends. You have calculated the average tension along the rod, but you have not considered how the tension varies along the rod. You should consider many small masses $\delta m$ which (we assume) are evenly distributed along the length of the rod. $\endgroup$
    – gandalf61
    Commented Dec 23, 2023 at 11:13

Not the answer you're looking for? Browse other questions tagged or ask your own question.