How accurate is radioactive decay rate formulae and is there room for error?

Question

So, lets ay I have 4 different samples of 1 mole of a radioactive element, and i check them after time of 1 half life has passed

before checking them can I be absolutely sure that

1. all the 4 experiments will have exactly the same amount of atoms left?
2. they all will have exactly(to a single atom) 0.5 moles left?

(assuming the conditions are same for all the samples)

Answer 1

Actually your experiment is a prime example of the binomial distribution.

the binomial distribution with parameters $n$ and $p$ is the discrete probability distribution of the number of successes in a sequence of $n$ independent experiments, each asking a yes–no question, and each with its own Boolean-valued outcome: success (with probability $p$) or failure (with probability $q=1-p$).

In your case you do the yes-no question "Has the atom decayed after one half-time?" for each atom. So $n=6.02\cdot 10^{23}$ is the initial number of atoms, and $p=\frac{1}{2}$ is the probability that an individual atom has decayed.

According to Binomial distribution - Expected value and variance the mean value of decayed atoms is $$\mu=np = 3.01\cdot 10^{23}=0.5\text{ mol}$$ and its standard deviation is $$\sigma=\sqrt{np(1-p)}=3.88\cdot 10^{11}=6.4\cdot 10^{-13}\text{ mol}$$

In most cases ($\approx 68$%) the actual number of decayed atoms will be in the range $\mu\pm\sigma$. Of course this tiny relative deviation is far beyond measurable.

But for smaller numbers of decayed atoms (let us say with $\mu=100$) it is easy to verify the predicted $\sigma$ in experiment.

Answer 2

The answer to both questions is no. The decay of an individual atom in a fixed time interval is probabilistic in nature, i.e. there is a probability (that depends on the length of the time interval) for deacy that is not equal to 0 or 1.

When large ensembles of atoms are together, one can use laws of probability do deduce how one expects the number of decays to change with time, but there is still room for randomness since the number of atoms is finite.

Answer 3

The answer to your question can be illustrated with a simple example.
Tossing a coin and comparing the number of heads with the total number of throws.
Just to give you an idea of the scale that you are asking consider starting with a given number of radioactive nuclei (number of coin tosses) with a probability of decay (a head) of $0.5$ in a given time (half-life) and in that time exactly one half of them decay (exactly half are heads).

This is an example of a binomial distribution with probability $=0.5$.
A good calculator for the computations is provide by WolframAlpha.

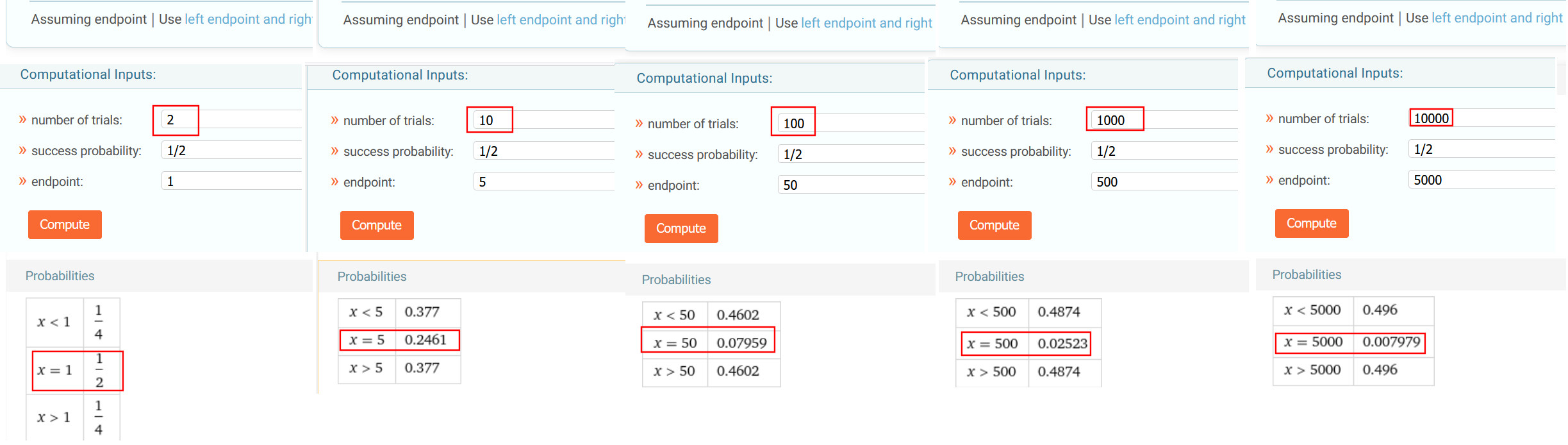
You will note that as the number of radioactive atoms (coin tosses) increases the probability of exactly half of the decaying (coin showing a head) decreases.
So for $0.5$ of a mole of radioactive atoms the probability of exactly half of them decaying will be rather small.

This is equivalent to there being exactly 0.25 mole of heads after 0.5 mole of tosses!

However the probability of this happening, although very small, is not as small as I expected, $\approx 10^{-12}$.
The value can be obtained by assuming that the binomial approximates to a normal distribution for large values of $n$, the number of atoms.
With a probability of $0.5$ the mean of the normal distribution is $3\times 10^{23}/2=1.5\times 10^{23}$ with a standard deviation of $\sqrt{3\times 10^{23}*0.5*0.5} \approx 2.74\times 10^{11}$. With a deviation of 0.5 from the mean, $z=0.5/2.74\times 10^{11}= 1.83 \times 10^{-12}$, leading to a probability of the order of one in a trillion.