In the book "Introductory Nuclear Physics" by Kenneth S Krane, it is written that "there are four ways to couple $s_n$, $s_p$, and $l$
to get a total $I$=1" for Total Angular Momentum $I=s_n+s_p+l$ of Deuteron. But I can't understand how they got these 4 possible ways, specially (c). Can anyone please explain this to me? Thank you.
$\begingroup$
$\endgroup$
2
-
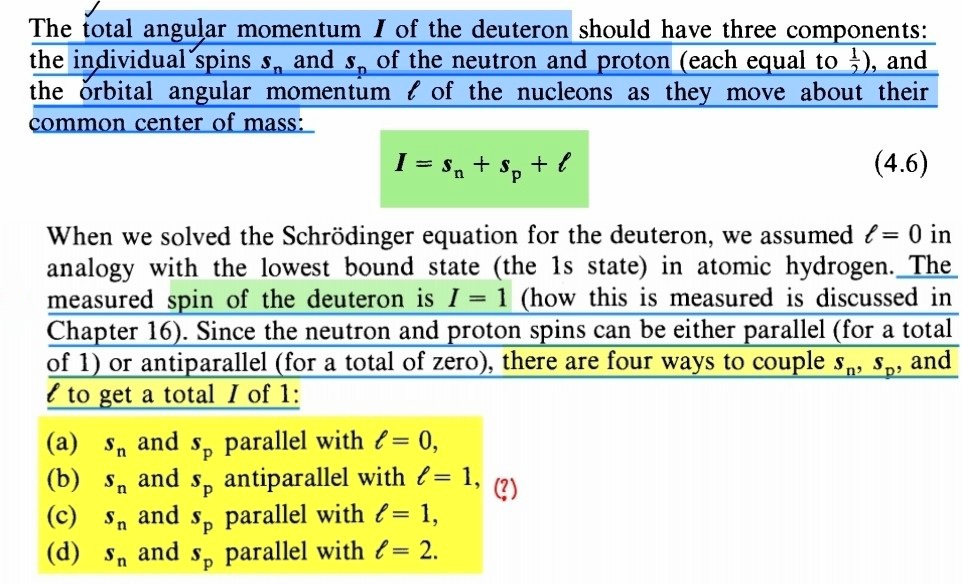
1$\begingroup$ Can you convince the reader you have mastered the addition of two angular momenta? $\endgroup$– Cosmas ZachosCommented Dec 16, 2023 at 14:29
-
$\begingroup$ @CosmasZachos Sir yes. $\endgroup$– LusypherCommented Dec 17, 2023 at 6:36
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
One of the important results in quantum mechanics is the composition rule for total angular momenta.
For any sum J of two momenta (that could be both spins, angular momenta, or one of each) which I'll call S and L (with their quantum numbers s and l) the eigenvalues of $|J|^2$ are $j(j+1)$ where $j$ goes from $|l-s|$ to $l+s$ in integer steps.
In this case, S is the sum of the two spins and L is the angular momentum. The spins are both $1/2$ in absolute value. The 4 cases are as follows:
a) s = 1 and l = 0. j is necessarily 1
b) s = 0 and l = 1. j is necessarily 1
c) s = 1 and l = 1. j goes from 0 to 2 (includes 1)
d) s = 1 and l = 2. j goes from 1 to 3 (includes 1)
Therefore you have 4 possible ways of obtaining j = 1. An S = 1 triplet and an S = 0 singlet.
Harish Chandra Rajpoot
2,50920 gold badges24 silver badges41 bronze badges
answered Dec 16, 2023 at 13:03
$\begingroup$
$\endgroup$
1
Denote by $s$ a spin $s$ irreducible representation of angular momentum (where $s= 1/2, 1, 3/2, \dots $).
Using the "angular momentum addition" rules we have (the first two factors are the spins and the third is the orbital angular momentum) $$ \tag{1} 1/2 \otimes 1/2 \otimes l = (1 \oplus 0 ) \otimes l = (1\otimes l ) \oplus (0 \otimes l) $$ and $$ 0 \otimes l = l $$ as well as (for $l\neq0$) $$ \tag{2} 1 \otimes l = (l+1) \oplus l \oplus (l-1). $$ and $1\otimes 0 = 1 $ (in the case $l=0$).
Now we can choose from either summand on the right hand side of Equation (1) to get the total angular momentum of 1. If we choose from the second summand (spin singlet, "anti parallel") we must have $l=1$ to satisfy $I=1$.
If we choose from the first summand (spin triplet, "parallel"), then there are multiple options. In Equation (2) we can see that the possibilites to get $I=1$ are $l=1,l=2,l=0$. Note that the numbers on the right hand side of Equation 2 are the values of $I$ associated to that representation.
-
1$\begingroup$ When writing direct products, it's customary to use the multiplicity, (1) would read ${\bf 2} \otimes {\bf 2} \otimes ({\bf 2l+1}) = ({\bf 3}_S \oplus {\bf 1}_A) \otimes ({\bf 2l+1})$, where I have included subscribes for representations with definite (anti)symmetry. $\endgroup$– JEBCommented Dec 16, 2023 at 18:08