Corresponding Measurement in Quantum Mechanics

Question

I recently started with R.Shankar Principles of Quantum Mechanics. There he discuss the part of measurement in Quantum mechanics which I didn't understood. For example I want to know the the momentum of a particle in state $|\psi\rangle$ the it will take one of the eigenstates $|p\rangle$(to each corresponding eigenvalue $p$).

• My first question is: Is the system now described by $|p\rangle$?

• Now If you measure the position of the particle, what will you get?
  

According to the book if we have measured the state of the particle to be $|p\rangle$ and the we measure the position, then:$$|p\rangle=\int{|x\rangle\langle x|p\rangle dx}$$ and that it will force the system into some eigenstate $x$. I didn't understand this part at all. How does the above integral describe the fact the measurement will force the system into some eigenstate $|x\rangle$?(In the book it is page number 122-124).Your response will be really valuable.

Answer 1

Actually, a measurement acts on the wavefunction of the system as a projection onto an eigenstate of the measured observable. Consequently, in your first question, the state $|\psi\rangle$ is sent to $|p\rangle\langle p|\psi\rangle = \psi(p)|p\rangle$. Then, if you measure the position on top of that, the latter state will be itself projected onto $|x\rangle$.

The integral expression your book provides is nothing else than the closure/completeness relation of the position eigenbasis applied to the momentum eigenstate $|p\rangle$ in order to change the basis and facilitate some calculations. It is true in general and doesn't explain the measurement per se, even if it may highlight the fact that the final state will be colinear to $|x\rangle$. However, this step can be avoided, since the successive measurements, namely $|\psi\rangle \rightarrow |p\rangle\langle p|\psi\rangle \rightarrow |x\rangle\langle x|p\rangle\langle p|\psi\rangle \propto |x\rangle$, clearly shows this structure already.

Answer 2

The measurement postulate states that if we measure an observable (such as position or momentum) on a quantum system, we can only get one of the possible eigenvalues of that observable, with a certain probability. The probability is given by the square of the amplitude of the state vector projected onto the corresponding eigenstate. For example, if the system is in state ∣ψ⟩, and we measure the momentum, we can get any value p with probability ∣⟨p∣ψ⟩∣2, where ∣p⟩ is the eigenstate of momentum with eigenvalue p. Immediately after the measurement, the system will collapse into the eigenstate ∣p⟩, and any subsequent measurement of the momentum will yield the same value p with certainty.

1. To answer your first question, yes, the system is now described by ∣p⟩ after the measurement of the momentum. This means that the system has a definite momentum, but an indefinite position. The uncertainty principle tells us that we cannot know both the position and the momentum of a quantum system with arbitrary precision, so if one of them is sharp, the other must be fuzzy.

2. To answer your second question, if you measure the position of the particle after measuring the momentum, you will get a random result, with a uniform probability distribution over the entire space. This is because the momentum eigenstate ∣p⟩ is a plane wave, which has the same amplitude everywhere. Mathematically, this means that the position eigenstate ∣x⟩ has the same overlap with ∣p⟩ for any value of x, so the probability of getting any position is the same. Physically, this means that the particle is equally likely to be found anywhere, and has no preferred location.

3. To answer your third question, the integral you wrote is the expression of the state ∣p⟩ in terms of the position basis. It is a superposition of all possible position eigenstates, weighted by the amplitude ⟨x∣p⟩. This amplitude is given by the Fourier transform of the momentum eigenstate, which is a complex exponential:

This means that the state ∣p⟩ has a constant phase factor that varies linearly with x. When you measure the position, you are projecting the state ∣p⟩ onto one of the position eigenstates ∣x⟩. This projection will collapse the state into ∣x⟩, and eliminate the phase factor. The probability of getting a particular value of x is given by the square of the modulus of the amplitude, which is constant:

The integral you wrote can be interpreted as the sum of all possible outcomes of the position measurement, weighted by their probabilities. It is equal to the state ∣p⟩, because it satisfies the completeness relation, which states that the sum of all projectors onto the position eigenstates is equal to the identity operator:

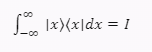
This relation ensures that the total probability of all possible outcomes is one, and that the state vector is preserved after the measurement.