I've seen so many times that the expression of the tangential acceleration is known to be: $$a_t=\ddot{s}$$ but from the expression of the acceleration in spherical coordinates, in the tangential component I can't see where it comes from $$\vec a=(\dots)\vec u_r+(r\ddot{\theta}+2\dot{r}\dot{\theta}-r\sin\theta \cos\theta \dot{\phi^2})\vec u_\theta+ (\dots) \vec u_\phi$$ being $\phi$ the angle between the $y$-axis and the projection of that vector in the $xy$-plane, as usual, and $\theta$ the angle between the $z$-axis and the $\vec r$ vector. I don't see how any of that expression transforms to that of the $\ddot{s}$
-
$\begingroup$ @Ghoster I recall now, it comes from the Frenet trihedron, I lack of how to connect that trihedron to spherical coordinates I think what's happening $\endgroup$– UlshyCommented Nov 26, 2023 at 23:27
-
$\begingroup$ @Ghoster but then how does the Frenet trihedron tell me that $a_t=\ddot{s}$ where $s$ is the length of the arc? I think I'm mixing up $\ddot{r}$ and $\ddot{s}$, isn't it? $\endgroup$– UlshyCommented Nov 26, 2023 at 23:40
-
$\begingroup$ Yes, $r$ and $s$ are different things. $\endgroup$– GhosterCommented Nov 26, 2023 at 23:58
-
$\begingroup$ I’m going to delete my earlier comments since they don’t make sense after your edit. $\endgroup$– GhosterCommented Nov 27, 2023 at 0:00
-
1$\begingroup$ The acceleration in spherical coordinates is not directly related to the tangential acceleration along an arbitrary path. I think your confusion comes from mixing the word "spherical" in the name of the coordinate system with a "circular" path that can be followed by some arbitrary object. $\endgroup$– Pato GalmariniCommented Nov 27, 2023 at 0:01
1 Answer
The portion of the acceleration in spherical coordinates you have shown is the portion that is in the direction of the $\hat{\theta}$ unit vector. This vector is in the direction of a small change in theta while holding other coordinates constant. Because of this, $\hat{\theta}$ is only tangential when $r$ and $\phi$ are held constant. If you plug in $\dot{r} = 0$ and $\dot{\phi} = 0$, you will get $r \ddot{\theta}$ as the $\hat{\theta}$ component, and since $r$ and $\phi$ are held constant, that is now the tangential component. It is easy to see that $r \ddot{\theta}$ is equal to $\ddot{s}$ when you are moving along a circular path by differentiating the circular arc length formula twice with respect to time.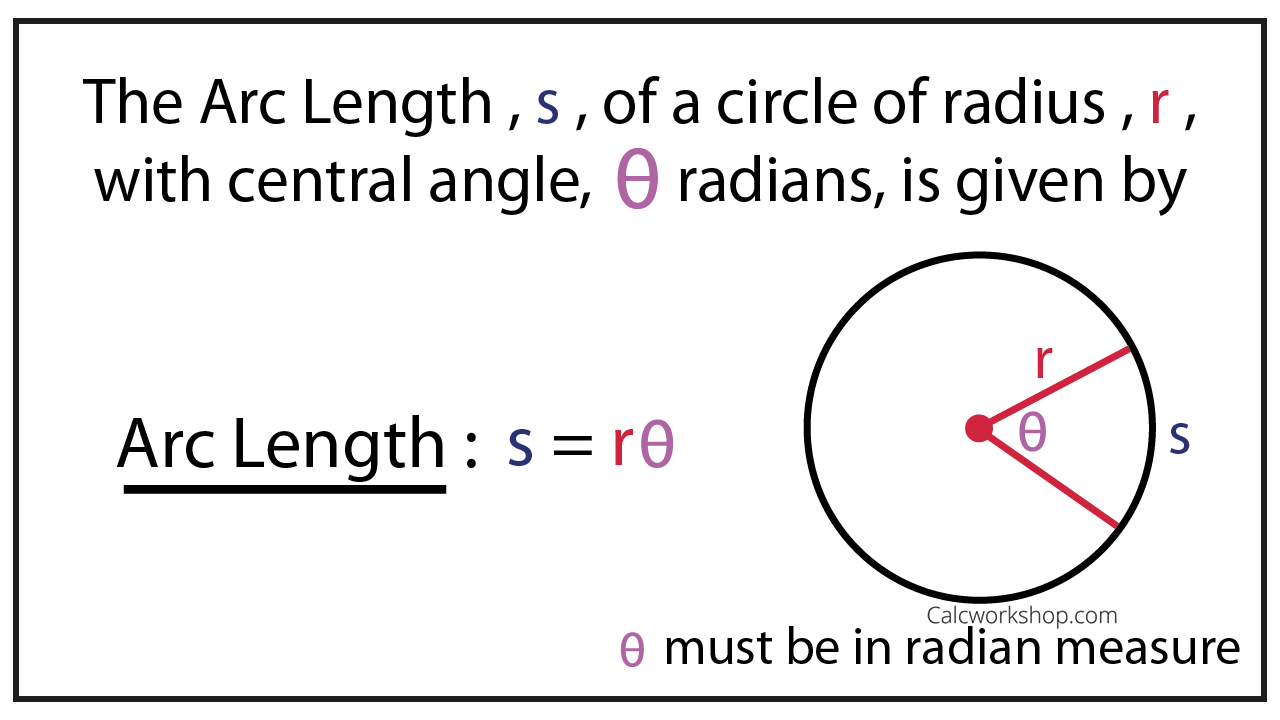
-
$\begingroup$ I don't see how $r\ddot \theta$ is equal to $\ddot s$ though, can you expand on that please? $\endgroup$– UlshyCommented Nov 27, 2023 at 19:55
-
$\begingroup$ When you are moving in a circular path with only $\theta$ changing you can use the arc length formula $\Delta s = r\Delta\theta$. Differentiating twice with respect to time gives $\ddot{s} = r\ddot{\theta}$. I added a picture to my answer to illustrate. $\endgroup$ Commented Nov 27, 2023 at 20:03
-
$\begingroup$ oh my god, I forgot about this, thank you! Even though why is the second derivative equivalent to the delta? shouldn't it be the first derivative? $\endgroup$– UlshyCommented Nov 27, 2023 at 20:45
-
1$\begingroup$ No problem. The second derivative isn't equivalent to the delta, it's just when you differentiate the delta twice with respect to time it becomes equivalent to the second derivative. If it's easier, you can think of the equation $s = r\theta$ with $s$ set to 0 when $\theta = 0$. Then it is easier to see that differentiating both sides twice leads to the correct equation. $\endgroup$ Commented Nov 27, 2023 at 21:05
-
1$\begingroup$ The normal acceleration is $\frac{v^2}{r}$. If we are still talking about $r$ and $\phi$ being held constant, then setting $\ddot{r} = \dot{\phi} = 0$ leaves us with $-r \dot{\theta}^2$ for the $\hat{r}$ component. The velocity is $r\dot{\theta}$, and when you square it and divide by $r$, one of the r's cancels. $\endgroup$ Commented Nov 28, 2023 at 14:14
