Meaning of $C$ in wavefunction equation ($\Psi_{MO} = C_1\phi_A(1s) + C_2\phi_B(1s)$, where $C_1=\pm C_2$)

Question

I've just cracked open a biophysics textbook and it's all fine up until the introduction of the letter C in a wavefunction equation, and declaring C₁= ±C₂

I've had lectures on eigenfunctions etc. before and no recollection of what C is, and it's not introduced earlier in the book (Biophysics, Pattabhi and Guatham, 2002).

A scan of the section in question (1.4) is below:

Anyone care to enlighten me?

Answer 1

This is the LCAO approximation i.e. approximating the wavefunction of a hydrogen molecule as the sum/different of the two atomic orbitals. If $\phi_A$ is the wavefunction of one hydrogen atom and $\phi_B$ the wavefunction of the other then we guess that the wavefunction of the hydrogen molecule can be approximated as:

$$ \Psi_{H_2} = C_1\phi_A + C_2\phi_B $$

where $C_1$ and $C_2$ are constants to be determined. From symmetry we know that the ground state must have $C_1$ and $C_2$ equal in magnitude, so the only possibilities are $C_1 = C_2$ and $C_1 = -C_2$. That's why we can write the two molecular wavefunctions as :

$$ \Psi_+ = C\phi_A + C\phi_B = C \left( \phi_A + \phi_B \right) $$

and:

$$ \Psi_- = C\phi_A - C\phi_B = C \left( \phi_A - \phi_B \right) $$

where I've dropped the subscript $1$ because it isn't needed. The molecular orbitals $\Psi_+$ and $\Psi_-$ look like:

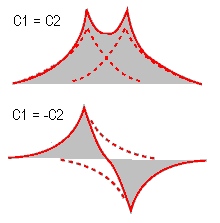
To actually calculate the value of $C$ you use the fact that $\Psi$ must be normalised so:

$$ C = \frac{1}{\sqrt{2 (1 + S)}} $$

where $S$ is the overlap integral $\langle\phi_A|\phi_B\rangle$.