0
$\begingroup$

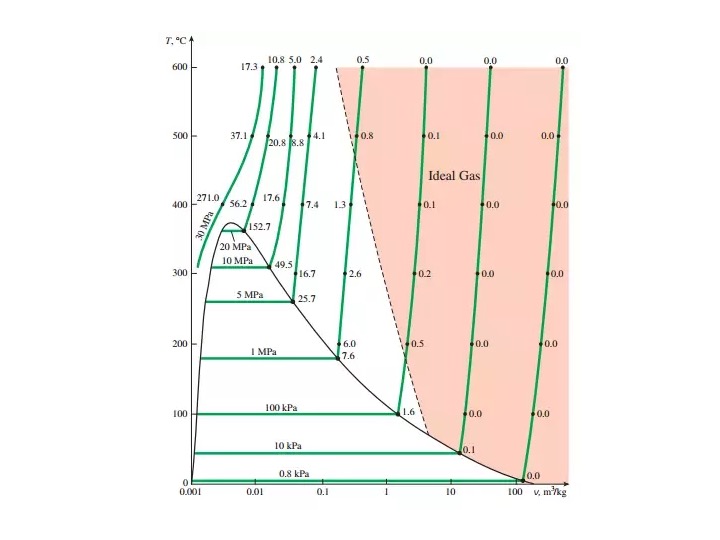
I am studying a chapter on pure substances in thermodynamics book by Yunus Cengel, where the author explains that a gas or vapour can be treated as ideal gas, when the temperature is sufficiently high and pressure is low relative to the critical temperature and pressure of the gas.

But when the author illustrates this concept in example problems in the succeeding chapters, for example analysing steady flow devices such as steam engines or pressure cooker, where the working fluid is primarily steam, the author deems the steam to superheated vapour in some problems, while on the hand treats it as saturated vapour (which cannot be an ideal gas) in others. This has significant implications on the results, which I am unable to capture.

Can you please explain, if possible, with the help of some state variable with numerical values to help understand 1.) when to treat steam as saturated vapour or superheated vapour and 2.) when do we treat any gas (including steam) as an ideal gas relative to its critical point (i.e., what does low pressure mean relative to the critical point, how low should it be?).

Thank you for taking the time read my doubts and answering them.

Improve this question
$\endgroup$
3
  • $\begingroup$ Why do you say that saturated water vapor cannot be treated as an ideal gas? $\endgroup$
    – Chet Miller
    Commented Oct 16, 2023 at 9:35
  • $\begingroup$ Get yourself a set of steam tables and calculate Pv/RT as a function of pressure for saturated steam vapor. Plot the points on a graph. $\endgroup$
    – Chet Miller
    Commented Oct 16, 2023 at 13:28
  • $\begingroup$ For steam in particular, engineers have compiled exquisitely detailed empirical data tables of steam's characteristics under different temperature and pressure regimes. If you're looking for useful rules of thumb about them and you don't have a professor or a TA that you can ask, you might try Engineering SE. You can find steam tables in any edition of the CRC Handbook published in the last hundred years or so. $\endgroup$
    – g s
    Commented Oct 16, 2023 at 16:29

2 Answers 2

Reset to default
2
$\begingroup$

When does steam or any gas be deemed ideal gas?

There are no truly ideal gases. Only those that sufficiently approach ideal gas behavior to enable the application of the ideal gas law with little error.

Generally, a gas behaves more like an ideal gas (obey the ideal gas law) at higher temperatures and lower pressures. This is because the internal potential energy due to intermolecular forces becomes less significant compared to the internal kinetic energy of the gas as the size of the molecules is much much less than their separation.

...saturated vapor (which cannot be an ideal gas) in others.

Saturated water vapor can approach ideal gas behavior at very low pressures. Note that according to the diagram below, along the saturated vapor curve, at pressures below 10 kPa water vapor can be treated as an ideal gas with negligible error (less than 0.1 percent).

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ Hi Bob D, thank you for your answer. In your third paragraph, where you mention about saturated water vapour, does this behaviour of ideal gases existing at low pressures & high temperature, and only apply to saturated water vapour, OR is this generally true for most liquids (such as refrigerants and other working fluids). Secondly, can we just roughly approximate a fluid to be an ideal gas as long its state lies beyond the saturated vapour point in the P-v and T-v diagram? $\endgroup$
    – Prajwal Kori
    Commented Oct 22, 2023 at 5:51
  • $\begingroup$ @PrajwalKori (1) Refrigerant (R134a) molecules are much larger and heavier than water vapor molecules, which are even lighter than dry air (O2 and N2). I think it highly unlikely it could behave like an ideal gas, but see below. (2) It depends on how much “error” you are willing to accept. For example, from the graph above, is a 25.7% error acceptable at 5 mPa for water vapor? $\endgroup$
    – Bob D
    Commented Oct 22, 2023 at 11:36
1
$\begingroup$

A gas may be treated as an ideal gas if its equation of state coincides with that of an ideal gas ($PV=nRT$). On the practical side, this is almost invariably true as long as you stay sufficiently far away from phase boundaries (gas-solid, gas-liquid, etc).

Improve this answer
$\endgroup$
2
  • $\begingroup$ You should have used $PV\approx nRT$ rather than $PV=nRT$. The latter (strict equality) is never exactly correct for a real gas. The former (approximately equal) is oftentimes close enough. $\endgroup$
    – David Hammen
    Commented Oct 16, 2023 at 9:58
  • 1
    $\begingroup$ @DavidHammen By that rationale, the area of a circle is $\approx \pi R^2$ $\endgroup$
    – Chet Miller
    Commented Oct 16, 2023 at 13:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.