Here's a level scheme for cobalt-60 which I decorated recently to answer another question about gamma emission. The 99.9% decay pathway is highlighted. This is a beta emitter instead of an alpha emitter, but I don't think that's a big deal for your question.
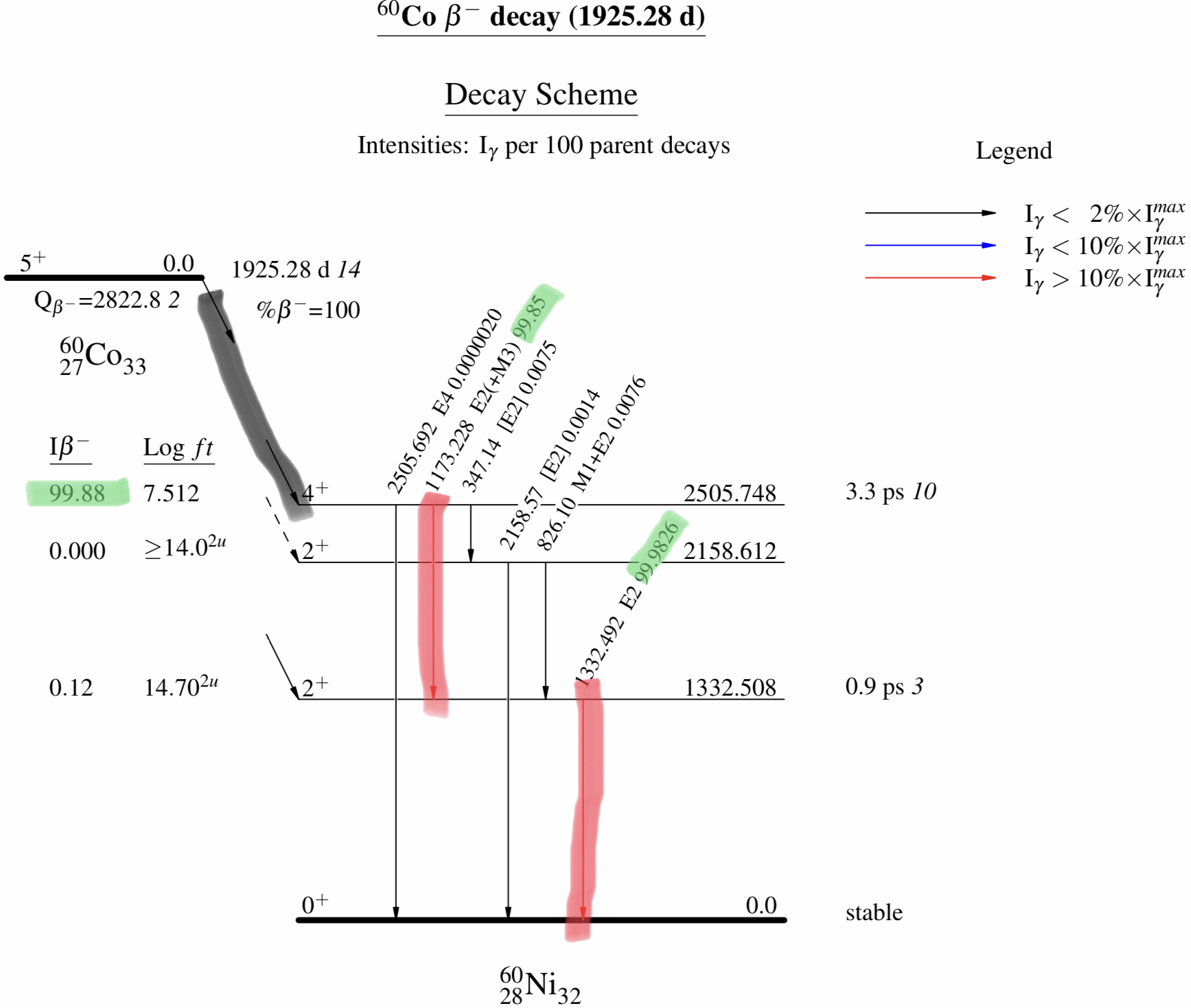
The diagram includes high-precision energies in kilo-eV, but for the sake of brevity I'm going to quote them to lower precision and use mega-eV.
From the $Q$-value we see that we have 2.82 MeV total energy to play with. Nearly all of the decays go to the $4^+$ excited state, for reasons related to angular momentum, which has an excitation energy of 2.51 MeV above the nickel-60 ground state. In most cases the nickel emits two photons, each carrying two units of angular momentum. The 1.17 MeV photon takes you to an intermediate state with energy 1.33 MeV, and then a 1.33 MeV photon takes you to the ground state. We have $1.33 + 1.17 = 2.50$, so the two photons have carried away all of the excitation energy (within a rounding error). The electron and neutrino actually don't carry off much of the energy in this dominant path, only about 0.32 MeV, which gets us to the entire $Q$-value of 2.82 MeV.
About one decay per thousand goes to the $2^+$ intermediate state at 1.33 MeV, which then decays by the same single photon as before. In that case the electron and neutrino share more energy, about 1.5 MeV.
If you look at the gamma energies through the fourth or fifth significant digits, you'll notice that they are actually different from (and slightly less than) the energies of the associated states.
For example, the $4^+$ state at $2\,505.748\rm\,keV$ can very rarely decay at rest by emitting a single photon, whose lab-frame energy is only $2\,505.692\rm\,keV$.
That's because the approximation where the nickel nucleus has infinite mass, relative to the energies of the decays, is only good to three or four significant figures. In order to conserve momentum, the decaying nucleus must recoil when it emits its photon. The energy liberated in each step of the decay is shared among all of the decay products, including the heavy nucleus.

