Why should the shape of the surface affect the flux in Faraday's law?

Question

Many 'proofs' of Faraday's law use the third of the Maxwell's equations $\nabla \times \bf {E} = -\dfrac {\partial B}{\partial \it t}$ to simplify the flux integral by first splitting it into two integrals. One for the time-dependent magnetic field and one for the change in flux when the surface is time dependent.

$$\dfrac {d\phi_{B}}{dt} \Bigg|_{t=t_{0}}= \int \int_{\Sigma (t_{0})} \dfrac {\partial \textbf {B}}{\partial t} \Bigg |_{t = t_{0}} \cdot d \textbf {a} + \dfrac {d}{dt} \int \int_{\Sigma(t)} \textbf B( t_{0}) \cdot d \bf a $$

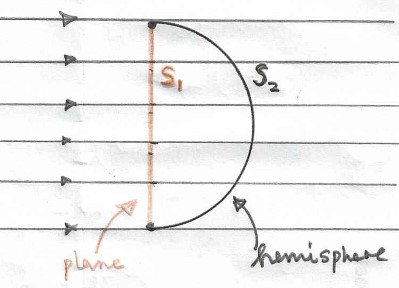
Why is the second of these integrals even needed? $\nabla \cdot \bf B$ $ = 0$ so from vector calculus we already know that the flux across surfaces with the same boundary curve $\partial \Sigma$ is the same...

This also doesn't make sense intuitively - $\Sigma$ is not a physical surface so the flux should't depend on it's actual shape but only it's boundary (i.e. the (physical) conducting loop). I get the derivations but can't see why the SHAPE of the surface should effect the flux. So what's going on here? Or is the divergence of time-dependent magnetic fields not zero in some cases?

Answer 1

My bad - some ambiguous language in the proof really made things confusing.

Most sources will use the term 'time-dependent surface' to mean that the surface is changing (i.e. changing shape and/or position) because the LOOP ITSELF is changing (i.e. moving through the field or changing shape). So the second of these integrals actually gives the MOTIONAL e.m.f.

Changing ONLY the surface while keeping it's boundary curve (i.e. the conducting loop) and the magnetic field the same won't effect anything - this follows directly from the fact that $\nabla \cdot \bf B$ $= 0$ which means that it can be written as $\bf B$ $=$ $\nabla \times \bf A$ and it's flux will be the same across any surface with the same boundary. (Apply Stokes' theorem to $\bf A$)

Answer 2

The double integral is an integral over an open surface $S$, your $\Sigma (t)$, bounded by closed loop $L$ as shown below.

The dot product ${\bf B}\cdot \rm d{\bf a} $ evaluates the scalar magnetic flux passing through area $\rm d{\bf a} $.

Pulling $\frac {\partial}{\partial t}$ out of the integral means that it can be changed to $\frac{d}{dt}$.

---

The flux through the plane, surface area $S_1$, is the same as the flux through the hemisphere, surface area $S_2$.

The mathematics is here - Electric flux through hemisphere

Answer 3

I don't understand the question completely, but I'll say that the surface integral of divergence-less vector fields only depend on the integration boundaries involved.

In other words since $$\nabla\cdot \mathbf{B}=0,$$ we have in general $$\mathbf{B}=\nabla\times\mathbf{A},$$ and so by Stoke's theorem $$\int(\nabla\times\mathbf{A})\cdot d\mathbf{a}=\oint\mathbf{A}\cdot d\mathbf{l}$$ which means that the flux only depends on the boundary curve.