I am searching, without success, what is the meaning of each component of the metric tensor in the context of General Relativity.
$$ g_{\mu\nu}=\left[\begin{matrix}g_{00}&g_{01}&g_{02}&g_{03}\\g_{10}&g_{11}&g_{12}&g_{13}\\g_{20}&g_{21}&g_{22}&g_{23}\\g_{30}&g_{31}&g_{32}&g_{33}\\\end{matrix}\right]\ $$
This is like finding the meaning of individual coordinates of a classical position vector.
The vertical component has something of a special meaning because gravity is vertical and it matters to just about everything we do. Inside the space station, it isn't so important.
East and North have meaning if you don't want to get lost. But nothing in physics keeps me from basing a map on NorthEast and NorthWest.
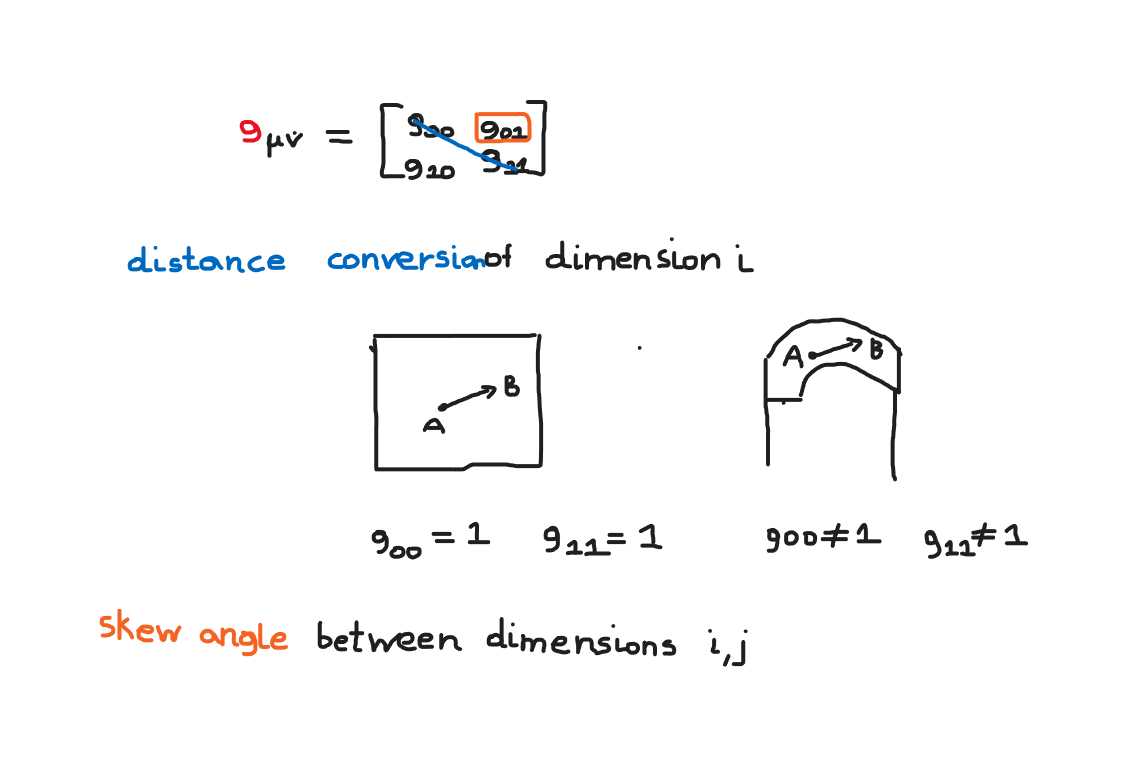
For simplicity (and for easier drawing) let us first consider a metric in 2-dimensional space instead 4-dimensional space-time, i.e. $$g_{\mu\nu}=\begin{bmatrix} g_{11} & g_{12} \\ g_{21} & g_{22} \end{bmatrix}$$
Now consider a unit-parallelogram in this 2-dimensional space, with both its coordinate differences $=1$.
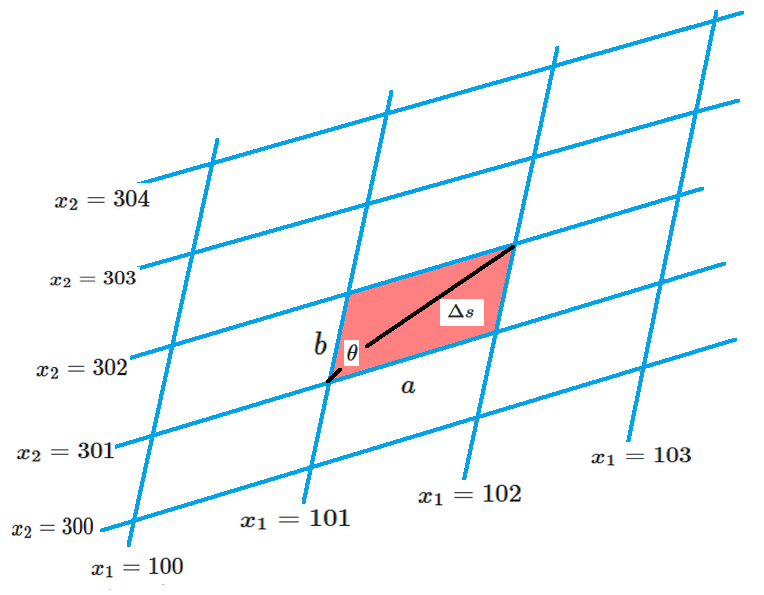
It has side lengths $a$ and $b$, and an angle $\theta$ between the two sides. Then, according to the law of cosines, the diagonal $\Delta s$ of this unit-parallelogram is given by $$(\Delta s)^2=a^2+b^2+2ab\cos\theta$$
For a general parallelogram (with arbitrary coordinate differences $\Delta x_1$ and $\Delta x_2$) its diagonal length $\Delta s$ is given by $$(\Delta s)^2=a^2(\Delta x_1)^2+b^2(\Delta x_2)^2+2ab\cos\theta\ \Delta x_1\ \Delta x_2$$
That means the metric is $$g_{\mu\nu}=\begin{bmatrix} g_{11} & g_{12} \\ g_{21} & g_{22} \end{bmatrix} =\begin{bmatrix} a^2 & ab\cos\theta \\ ab\cos\theta & b^2 \end{bmatrix}$$
which gives you the geometric meanings of all the metric components.
Now you can repeat this reasoning in 3-dimensional space (using a parallel-epiped instead of a parallelogram) or in 4-dimensional space-time.
The diagonal metric components $g_{ii}$ give the square of the length of the unit-parallel-epiped in $x_i$ direction. And the off-diagonal metric components $g_{ij}$ are related to the angle between the $x_i$ and $x_j$ direction.
It is perhaps better to exercise with simpler examples before deal with GR to have an intuition on the metric tensor.
Plane coordinates $xy$ with non-orthogonal axis leads to a metric with non-zero diagonal elements. On the other hand, polar coordinates $r \theta$ in the plane, where the basis vectors are perpendicular for every point, has zero diagonal elements.
Back to GR, the Schwarzschild metric $t, r, \theta, \phi$ has the unit vectors mutually orthogonal at every point. Its diagonal elements are also zero.
GR uses tensors to describe spacetime.A tensor is a vector in non-Euclidean space of any dimension.Any tensor can be written in the form of a nxn matrix.
Note that the matrix which describes a non-Euclidean space is a upper or a lower triangular matrix because the angle between dimensions i,j is the same with the angle between dimensions j,i.
The trivial answer is $$g_{ik}(x) = G(e_i,e_k)$$ where $G$ is the abstract bilinear symmetric map form tangent vectors at point $x$ to the the number field, defining the local geometry, and $e_i = \partial_{x^i}$ is the local basis of tangent vectors, defined by translation of a point with all coordinates constant except $x^i$.
The triviality is best seen by the mixed metric tensor, that pairs coordinate differentials a md tangent vectors $$g^i_k = G(dx^i,e_i) = \delta_{ik} = \partial_{x^1}\ dx^k$$ So the diagonal elements are the euclidean lengths squared, the off-diagonal elements are what is called the cos-theorem in euclidean geometry $$g_{ik} = e_i . e_k = |e_i||e_k| \quad \cos (e_i,e_k)$$ In space time $g_{00}$ measures the proper time squared along a curve, positive for a physical worldline and negative for spatial distances. The off-diagonal space-time elements $g_{0k}$ measure Lorentz boost angle between time and spatial directions in the sense of the cos-theorem with imaginary angles.
