Assuming the earth is either at the perihelion or at the aphelion, it is easy to see the Runge-Lenz (RL) vector is directed along the line joining the perihelion and aphelion. Since the RL vector is a constant vector, its direction (and also magnitude) must remain the same at all points of the orbit. So if we want to draw this vector at other points of the orbits, it will be drawn as a vector parallel to the line joining the perihelion and aphelion. Is this right?
$\begingroup$
$\endgroup$
1
asked Sep 9, 2023 at 18:19
-
1$\begingroup$ Have you tried just directly calculating the vector for general position along earths orbit? $\endgroup$– TriatticusCommented Sep 9, 2023 at 18:27
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
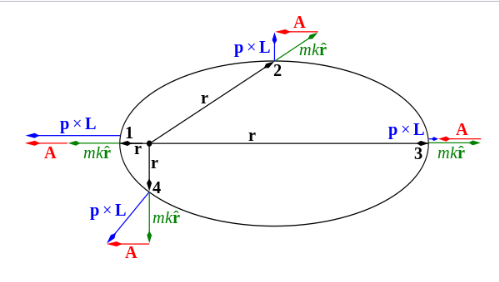
Yes, you are right. The Laplace-Runge-Lenz vector is constantly pointing in the same direction. See the red vector $\mathbf{A}$ in the image below.
Figure 1: The LRL vector $\mathbf{A}$ (shown in red) at four points (labeled 1, 2, 3 and 4) on the elliptical orbit of a bound point particle moving under an inverse-square central force. The center of attraction is shown as a small black circle from which the position vectors (likewise black) emanate. The angular momentum vector $\mathbf{L}$ is perpendicular to the orbit. The coplanar vectors $\mathbf{p}\times\mathbf{L}$ and $(mk/r)\mathbf{r}$ are shown in blue and green, respectively; these variables are defined below. The vector $\mathbf{A}$ is constant in direction and magnitude.
(image/text from Wikipedia Laplace-Runge-Lenz vector)
answered Sep 9, 2023 at 18:48