How do you know mercury changes monotonically with temperature if mercury itself is used to make the thermometer?

Question

In the book I am reading recently "Concept of physics" volume 2 by professor H.C. Verma it says that (I am just summarizing the main points chronologically)

• Energy is transferred from hot bodies to cold bodies when they are placed in contact.
• The energy that transfer from one body to other without any mechanical work involved is called heat. (then the book states zeroth law of thermodynamics)
• All bodies in thermal equilibrium assigned equal temperature(I am assuming this definition of temperature).A hotter body is assigned higher temperature than a colder body.

Then the book says

Our next task is to define a scale temperature so that we can give numerical value to the temperature of a body. To do this we can choose a substance and look for its measurable quantity which monotonically changes with temperature. The temperature can then be defined as a chosen function of this property.

and then it explains how to make a mercury thermometer (by taking boiling point and freezing point then dividing it to 100 equal parts).

My doubt is how do we know mercury's volume increases monotonically with temperature.

Edit:- Yes I was confused between monotonicity and linearity as pointed out by you guys,I actually wanted to ask how it varies linearly and yes the answers below also answers this questions.

Thank you

Answer 1

Monotony just means that hotter always means bigger, unlike water that shrinks as you heat it from 0 °C to 4 °C. Above 4 °C it expands again. This means that about 2 °C and about 5 °C will give the same reading on a water thermometer. And this is why we do NOT use water thermometers.

You probably thought that monotony means "at the same speed". It does not mean that. All it means is that it increases all the way, so that you won't get the same volume at two different temperatures.

Fortunately, most substances, mercury included, work like that. They always increase in volume as they heat up.

To see if this holds, simply heat it slowly and look at it. Does it increase all the time? That is monotony, and you have a working thermometer.

Next put notches on the glass. This allows to you to say things like "if the mercury rises above this notch, the patient has a fever."

You start needing a standardized scale when you want to compare results with other scientists. That is when you start putting a notch at ice water temperature and another at boiling water, and dividing the difference in a hundred steps.

It is reasonable to expect that if another scientist does the same in their laboratory, they should get the same result. This assumes that mercury and water behaves the same in both places.

Note that in the beginning, all you care about is that 37 °C in one laboratory is the same as 37 °C in another. It does not matter if the scale is distorted in some way, as long as it is distorted in the same way for everybody.

I assume that later chapters of your book will go on to explain how we can make different types of thermometers and how they don't always agree. And how to resolve those disagreements. But you haven't reached that part yet. Read on!

Answer 2

This has actually been a very important problem in the history of measurement and physics more broadly. The solution was an iterative process of increasing internal consistency and precision of measurements. The story has been extensively covered in Hasok Chang's fascinating Inventing temperature, which is my source for the text below. A Twitter thread with some highlights from the book: https://twitter.com/zerdeve/status/1099815636652371968

1. Perception

Very roughly, early thermometers (1600s) were calibrated to our perception of heat - anything that measures temperature, should give higher value on a sunny summer day than on a winter night, its value should increase when placed closer to a flame and decrease when moved away etc. Any device that passes a lot of such tests is likely to be monotonically related to temperature. Note that already we can move beyond just sensation - if we know a device was well calibrated to perception on many ocasions and then we find our perception contradicted by the device, we may come to believe that this time it is our perception that is at fault.

2. Fixed points

Then people tried to establish "fixed points", i.e. events that reliably happened at the same temperature. The idea is that you take a device that seems to agree well with subjective perception of heat and measure the event multiple times. If it is truly fixed, you should measure the same response everytime. You can then try with a proto-thermometer based on a different principle. Many fixed points were proposed, but same later failed that criteria (e.g. bodily heat was tried as a fixed point, but found varying when measured with precise - but uncalibrated - thermometer). The freezing and boiling point of water was first used as fixed points around 1690.

Here the iterative nature of the process reveals itself again. Once we establish a fixed point with decent confidence, we can use that fixed point to validate thermometers and rule out devices that don't yield sufficiently fixed values at fixed points. We can then use those better devices to better validate fixed points, ... If we do this right, we will find better and better internal consistency in our methods.

As an example of the iterative nature, boiling point of water was a pretty strong candidate for a good fixed point initially (after it was realized you need to control the pressure). But it turned out that it is pretty fixed only if you are not too careful. If you take care to avoid all impurities etc., you can easily superheat the water beyond 100°C without boiling. So thermometers calibrated to boiling point were then used to disprove the boiling point as a fixed point. The steam point (temperature of saturated vapour) at atmospheric pressure is in fact a bit more consistent and was preferred since ~1780s.

3. Linearity

The next challenge was then find a linear scale of temperature. Below we see see measurements made with three different thermometers based on expanding liquid in a tube, calibrated to freezing and steam point of water as 0/100°C. The distance between 0 and 100 was then evenly split into 100 segments:

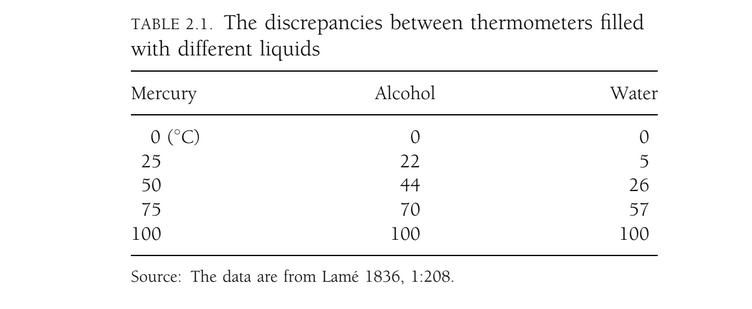
Obviously, there is a problem. One answer was mixing: if we mix equal amounts of say 0 degree water and 50 degree water, we should get 25 degree water. In this experiment, mercury clearly gives superior results to alcohol, water or other liquids.

However, mixing implicitly relies on the fact that the specifc heat (heat required to increase the temperature of 1 unit of volume by 1 degree) is the same for all baseline temperatures. This assumption was considered suspect (e.g. because the volume of liquid water changes slightly with temperature, so why wouldn't the specific heat change as well), and thus no full agreement on how to divide the scale between the fixed points was possible.

Enter gases. In early 1800s people have established that gases expand with temperature and this expansion is extremely regular for many different gases. This led to interest in air-based thermometers, but theoretically, there was still no reason to believe that the measurements of air thermometers are linear. Different theories of heat led to different conclusions.

The impasse was resolved in ~1850 by Henri Victor Regnault with a simple idea, high experimental skill and big budget: measuring the same substance (e.g. the same bath of oil) simultaneously by multiple thermometers should give the same values across the whole scale (e.g. as you slowly heat the oil up). So any thermometer technology where multiple devices do not agree across their whole scale is suspect. When such experiments were performed with high precision, it was determined that even relatively minor variations in the construction of a mercury thermometer (e.g. different glass used for the tube) meant that two thermometers that agreed on 0 and 100 did not agree at other points of the scale. However, even quite different models of constant-volume air-based thermometers (different volumes of gas, different gases) did in fact agree quite closely at all points of the scale. Air was thus clearly superior and remained the candidate for a good linear scale.

Finally, as we got to understand thermodynamics and got a good grasp on what heat actually is, we could justify thermometer measurements and the linearity of temperature by that (very succesful) theory. But note that this happened after the events described above and would likely be much harder to achieve without precise thermometers already available.

Answer 3

You do not know it a priori, you assume it to be true and if it leads to contradiction with other experiments and theories based on all prior experiments then you have to investigate the source of said contradiction and revise your theories accordingly. While the volume of mercury (and thus its length in constant cross section pipe) is a monotonic function of temperature that of water is not. The density of water is famously maximum at 4C, see, it is smaller before and after, thus thermometer based on water would not be monotonic between 0 and, say, 10C, and anybody in my neighborhood who forgets it before winter sets in will have a very unpleasant surprise comes thawing in spring time.

Answer 4

If you have no better theory of what temperature is, you define it as what your thermometer measures. That allows you to get started. Now, you can do experiments in thermal physics. If you don't see evidence of non-monotonicity in your experiments, you gain confidence. You invent other thermometers, and find relationships like Charles' law. That helps you abstract your notion of temperature away from your original realization. You get into an interplay of theory and experiment, redefining temperature and its realizations to better capture the physics, both quantitatively and conceptually.

Answer 5

Monotonicity is easy to observe. Hazard a guess, time might be involved to get linearity. A flame isn't the most stable source of heat, but could possibly boil water in a consistent amount of time. Take a thermometer of whatever composition and if it reads the same at the freezing point and the boiling point of water, add it to your collection.

Put the passing thermometers in an ice bath so they read the same. Apply consistent heat source and see how long it takes them to rise to the mark they show when exposed to boiling water. Collect the thermometers that move up half the distance in half the time, quarter the distance in quarter the time, and so on. Intuitively, a consistent heat source will raise the temperature and the distance at a steady rate. This turns out to be false in general, but for reasons mentioned later, it's not that relevant.

Heat capacity frequently depends on the temperature range involved. Because the molar specific heat of metals is roughly constant independent of temperature you'll find a metal liquid is the best option for an analog thermometer based on thermal expansion (depending on temp ranges). As I recall there are three liquid metals at room temperature and mercury, though very toxic, is the safest to work with.

So I think that's how you get to mercury thermometers as a good temperature measuring device without access to some other thermometer.

Answer 6

You could formulate that each increase by 1 degree uses the same amount of energy. Then heat the test tube in insulated container (calorimeter) and see if each equally sized piece of fuel results in same exact rise in temperature.
I guess an electrical coil would be best as you don't have to worry about containing the burn products of say a gas burner.
Or hold the test tube in open flame for n seconds, assuming this gives exact same thermal flux independent of the tube current temperature.

Such experiment should answer if the volume increase is linear or not.