How can flows of gases at low Mach number have approximately constant density despite varying pressure?

Question

The Wikipedia article on Bernoulli's Principle says:

In most flows of ... gases at low Mach number, the density of a fluid parcel can be considered to be constant, regardless of pressure variations in the flow.

Next to that is this picture:

I understand from the picture and from the article that higher velocity air flowing through the narrower part of the tube has lower pressure than lower velocity air flowing through the wider part of the tube. Assuming a constant temperature, wouldn't a mole of that lower pressure air take up more volume than a mole of the higher pressure air? How, then, can the density be considered constant regardless of pressure variations in the flow?

Answer 1

Here is another way of looking at Bernoulli's theorem, pressure and Mach number. Your question seems to be talking about two related concepts: (1) Relation between density and pressure and (2) Applicability of Bernoulli's theorem to low Mach number flows. I have addressed these two separately below and hope it adds some more insight to your question.

1. Based on the Gibbs phase rule for a single phase and single component system (such as water or air), there are two Degrees of Freedom (DoF), that is two intensive properties can be independently varied. This leads to the Equation of State (EoS). So, irrespective of flow speed or phase of matter (liquid or gas), just from Gibbs phase rule, one can say $\rho=f(p,T)$. For example the perfect gas equation may be familiar; $\rho=p/(RT)$. This equation is not applicable for condensed phases or near saturation states. But the general EoS $\rho=f(p,T)$ is applicable nevertheless. Then one can write: $$ d\rho=\frac{\partial\rho}{\partial T}dT+\frac{\partial\rho}{\partial p}dp$$ Now, lets set apart temperature variation for a moment and consider an isothermal process such that $d\rho=\rho \tau dp$, where $\tau$ is the isothermal compressibility. For water at room temperature, its value is $5.10^{-10}m^2/N$ and for air it is $10^{-5}m^2/N$ - thats five orders of magnitude higher. Therefore, qualitatively, a given pressure change in gas flows can bring about a significantly larger change in density than for liquids for the same pressure change. All the consideration so far is purely based on thermodynamic properties of a 2-DoF system undergoing a certain process. This makes sense; because, after all, density and pressure are thermodynamic properties of matter (unlike velocity).

Speaking of velocity, lets bring in flow effects.

2. Lets take an example of a fluid coming to rest. We consider a constant-density fluid (constant-density automatically means incompressible). $\rho=constant$ is itself the EoS and there is no dependence on pressure or temperature. Pressure satisfies a Poisson equation and temperature does not come into the picture. Thus there is no need for the energy equation. Under these conditions, obviously considering zero viscous effects the famous Bernoulli's theorem yields the stagnation pressure as: $$ p_o=p+\frac{1}{2}\rho v^2 \implies \frac{p_o-p}{\frac{1}{2}\rho v^2}=1 $$ If its a variable density flow (may be incompressible or compressible), then an explicit EoS and energy equation are required to close the system of governing equations. From gas dynamics, the stagnation pressure can be computed as: $$ p_o=p\{1+\frac{\gamma -1}{2}M^2\}^{\frac{\gamma}{\gamma-1}} $$ Using Taylor series $(1+x)^n=1+nx+\frac{n(n-1)}{2}x^2+h.o.t$, the above equation can be rewritten as: $$ \frac{p_o-p}{\frac{1}{2}\rho v^2}=1+\frac{M^2}{4}+h.o.t $$ Clearly, comparing the constant and variable-density EoS, the RHS is $1$ in the former and deviates by additional terms $\frac{M^2}{4}+h.o.t$ in the latter. Now, for low Mach number flows, $M<0.3$, the deviation (neglecting highr order terms) is $M^2/4<0.0225$, i.e., less than 2.25% - a small value. For many engineering flows $M<0.05$ and $M^2/4<0.000625$, i.e., less than 0.0625% - clearly, a very small value. It makes sense in such cases to altogether neglect compressible equations of motion and just use the standard Bernoulli's theorem to compute required flow field.

Hope, the above two points (EoS and how it comes into incompressible/compressible formulations via the Mach number) adds some insight to your question.

-With best regards.

Answer 2

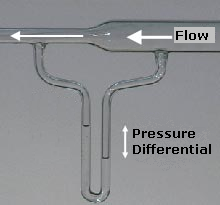
Density is indeed proportional to pressure in an ideal gas at a fixed temperature. So, when Bernoulli's principle states that pressure varies with the square of velocity assuming a fixed density, the conclusion seems to contradict the premise. However, even very small changes in pressure can have significant effects.

Consider the pressure difference required to lift a large airplane weighing 2 meganewtons: With a wing area of 430 $m^2$ (source), a pressure differential of ~4600 $N/m^2$ would be needed. While that may seem like a large pressure differential, keep in mind that atmospheric pressure is 101325 $N/m^2$, so the pressure differential needed to lift the airplane is relatively small: only ~4% of atmospheric pressure. A density variance of 4% in a gas flow can be considered insignificant.

Furthermore, in order for a gas flow to have a significant density change, the velocity change of the flow would have to be very large, at which point the "low Mach number" premise of Bernoulli's equation would no longer hold.

By Bernoulli's equation, that lift pressure calculated above, 4600 $N/m^2$, corresponds to a velocity difference of ~87 m/s, which is getting close to Mach 0.3. Above Mach 0.3 is not considered a low Mach number, so Bernoulli's equation would not apply.

There are variations on Bernoulli's equation that are applicable to compressible flows and take into account density varying within the flow.