The Lorentz factor $γ$, and Fermilabs derivation of it

Question

Before diving into my question, we first must acknowledge Einsteins two assumptions:

1. The laws of physics are the same for every observer and reference frame.

2. The speed of light is constant for different observers.

Background

I was trying to learn about the Lorentz Factor ( γ), and happened to come across Fermilabs video on it. Here is one question which arose to me after brainstorming for several hours on it and looking at different sources, such as CrashCourse; But I still do not get it when I compare with Fermilabs video.

Question

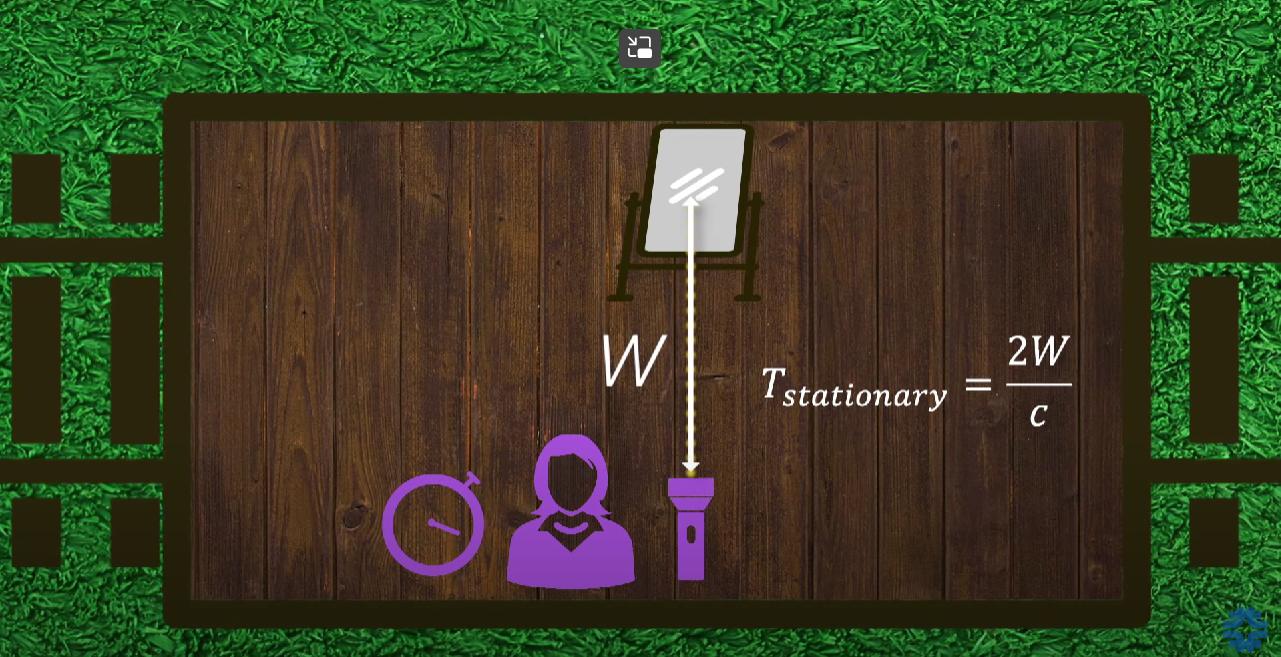
The distance from the flashlight to the mirror will be denoted as W and c as the speed of light. The time it will take from the flashlight back and forth will be 2W/c relative to the person inside the train.

---

Now, let us ask the observer outside the train how much time it will take for the light to travel to the mirror and bounce back to the person inside the train.

If we assume the speed of the light ray that the observer outside the train sees is c , and the train is moving with a velocity v; then using the Pythagorean Theorem, the speed from the flashlight to the mirror is $\sqrt {c^2 - v^2} $ relative to the person outside.

Therefore, the time it takes from the flashlight to the mirror and back to the person inside the train, relative to the observer outside will be 2W/$\sqrt{c^2 - v^2} $.

Now, here is my question. If both observers experience the same speed of light from the flashlight to the mirror and back, then it means that 2W/$\sqrt{c^2 - v^2} $ = 2W/c; but that is only true when v = 0! This simply does not make sense, it should also apply for objects in motion. That is the whole point of time dilation!

And that too, how is it even possible that both of the observers obtain different equations for the speed from the flashlight to the mirror if all observers experience the same speed of light, no matter their reference frame (according to Einsteins second postulate)? What does $\sqrt{c^2 - v^2} $ actually mean?

Answer 1

You're assuming the times are the same for both observers, but the whole point is that the times are not the same. The times measured by the train ($\Delta t$) and ground ($\Delta t'$) observers are different by a factor of \begin{align} \frac{\Delta t'}{\Delta t} = \frac{2W/\sqrt{c^2 - v^2}}{2W/c} = \frac{c}{\sqrt{c^2 - v^2}} = \frac{1}{\sqrt{1 - v^2/c^2}} = \gamma \end{align} This is the Lorentz factor, and it's always greater than 1 as long as $v$ is not zero. So the time dilation formula \begin{align} \Delta t' = \gamma \Delta t \end{align} tells us that for the ground observer, for whom the train is in relative motion, each "tick" of a clock on the train takes a longer time than it does for the train observer.

Answer 2

From the perspective of the outside observer, the light takes the longer, diagonal path shown in your second image, at the same speed $c$. It's the component of the speed in the direction of the mirror that is smaller and equal to $\sqrt{c^2-v^2}$.

Think of it like walking diagonally across a road. It will obviously take you longer to get to the other side than if you walked straight across at the same walking speed.