Cosmic Strings as Topological Defects (heuristics)

Question

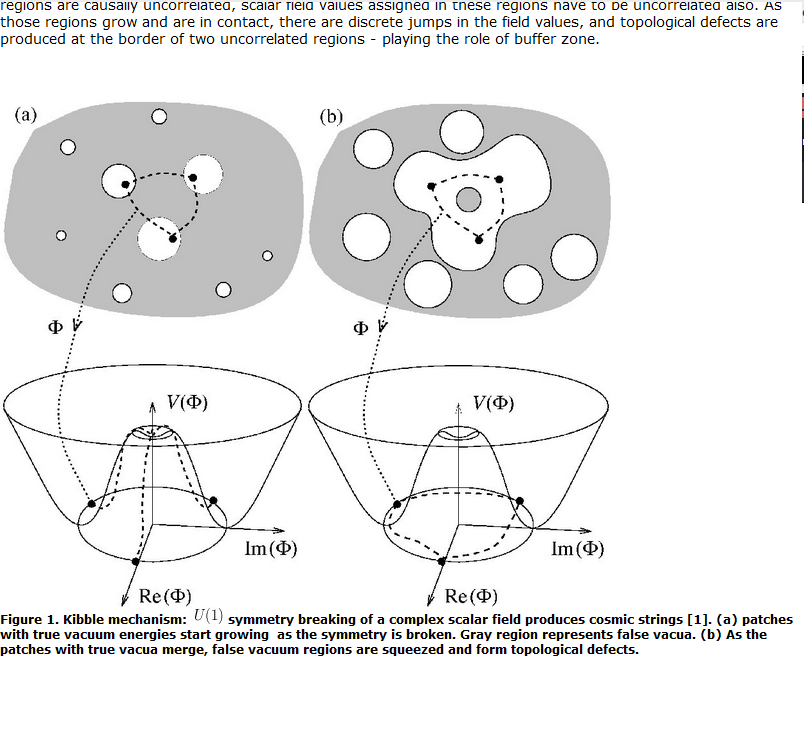
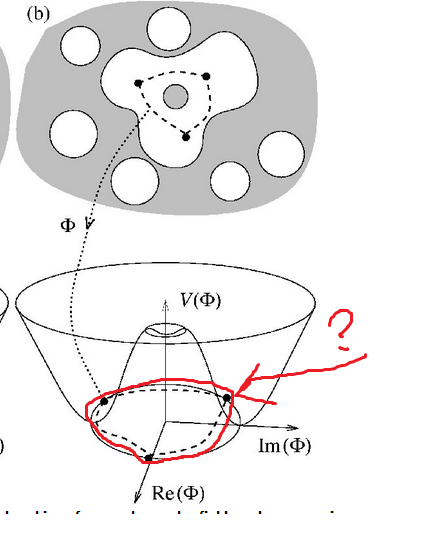
I have a lot of troubles to understand heuristically the principle behind Kibble's model for genesis of cosmic strings via Kibble mechanism. More precisely I not understand how to interpret following two pictures I found here:

Prelude: We start with usual Higgs field $\Phi$ with brocken $U(1)$-symmetry with model Lagrangian of the shape

$$ \mathcal{L}= \frac{1}{2}(D_{\mu}\Phi)^{\dagger}(D^{\mu}\Phi) - \frac{1}{4}F_{\mu \nu}F^{\mu \nu}-V(\Phi)$$

where $F_{\mu \nu}$ is the field strength tensor associated with the vector gauge boson $A_{\mu}$, the $D_{\mu}= \partial_{\mu} +igA_{\mu}$ the covariant dirivative with respect the gauge and $V(\Phi)= a \cdot (\vert \Phi \vert^2- \eta_{\nu}^2)^2 $
where $\eta_{\nu} \neq 0$ the degenerated vacuum expectation value of the Higgs field $\Phi$. Therefore the vacuum manifold $S^1 \cong U(1)$ can be parametrized ba $\eta_{\nu}e^{i \theta}$, $\theta \in [0,2\pi)$

Key Questions:

• In the upper two pictures (a) and (b) with grey background & white circles: Which kind of "space" is preciesely depicted there? The configuration space of states or the spatial space (="domain" of wave functions)

• in the right picture at the bottom: which meaning has the red marked dotted trajectory? It looks strange because it seemingly not lies on the graph of $V(\Phi)$

• which state function $\Phi$ is depicted there? An arbitrary one or such one coming from the "old" vacuum and after cooling down becoming frustrated

• What is here meant concretely by "false vacuum"?

• Finally: what is in this context precisely meant by topological defects (like eg cosmic strings): literally some defects is the space as depicted in a) and (b) (...and in which space do they "live"? The space of configurations of states or the "spatial" space) or certain solutions of the equation of motion ("solitons"). If the later is the case: why they are called "topological" defects?

Upshot: To forestall the legitimate objection that there are too many questions posed, it should be said that morally the concern of this question can be concisely summarized as "What are the depicted dashed trajectories in the picture, in which space do they live ( ...spacetime, configuration space?) and which role do they play in Kibble mechanism?"

Answer 1

1. The gray regions represent spatial regions which are still in the false vacuum state $\Phi =0$. The white regions are spatial regions in which the field has decayed to the true vacuum manifold $\Phi = \eta_\nu e^{i\theta}$.
2. The dotted line is a path through physical space along which the phase of the field changes by $2\pi$. In other words, the winding number of the phase of the Higgs field is $1$.
3. The $\Phi$ being depicted is an example of how, once the $U(1)$ symmetry is spontaneously broken, the decay of different spatial regions from the false vacuum to the true vacuum manifold can result in a non-zero winding number.
4. The important thing to understand is that if the winding number of the field around some loop is ever non-zero, then the field must vanish (or become singular) somewhere on the interior of the loop. You simply cannot remove that inner gray disc where $\Phi=0$ without introducing a discontinuity into the field. That region may remain a disk or it may shrink down to a point, but it cannot be continuously removed.

The fact that the inner gray region cannot be removed without introducing a discontinuity is what we mean when we say it is topologically-protected. A discontinuity in $\Phi$ would generally incur a formally infinite energy cost due to the presence of the spatial gradient terms in the Hamiltonian. Therefore, if the field every finds itself in a configuration with a non-zero winding number as depicted in those figures, then that inner region where $\Phi=0$ cannot decay, and so we obtain a stable configuration which is topologically distinct from the vacuum (where topologically distinct refers to the presence of a non-zero winding number around the "defect" region where $\Phi=0$).

This stable configuration is the cosmic string under consideration, and this mechanism - in which the decay of causally-separated regions into the true vacuum manifold creates a non-zero winding number of the phase - is the Kibble mechanism.