I am a complete layman and I read somewhere that the condensation point temperature of water is 100°C, same as boiling point temperature.
Thinking intuitively, this doesn't make sense to me. Doesn't water always condense at different relative temperatures like when air is warm and moist it condenses on a cold glass? Can anyone please explain to me how this works?
I read somewhere that the condensation point temperature of water is 100°C
Please try to give a pointer to sources so that the context, assumptions, reasoning, and credibility can be reviewed. Readers have no idea what you read or whether it applies in all circumstances.
Fortunately, we get a lot of online search hits for the claim. We find that the temperature being referred to is actually the maximum temperature at which condensation will occur at 100% relative humidity. (This is related to the dew point, or the temperature to which air should be cooled to reach 100% relative humidity.) This explains condensation on your cold glass, colder than the dew point; heat transfer with the glass cools the air and water vapor such that the latter is saturated and condenses, and the water lost through this gas-to-liquid phase change is continually replenished by air circulation.
Can anyone please explain to me how this works?
A quick run-through of thermodynamics, phase changes and vapor pressure: Nature seeks to maximize entropy (this is the Second Law), which usually means minimizing energy. For our familiar environment of near-constant temperature and pressure, the relevant energy is called the Gibbs free energy $G\equiv U+PV-TS$, with internal energy $U$, pressure $P$, volume $V$, temperature $T$, and entropy $S$.
So a lower value of $G$ is favored. You can see immediately that this function penalizes a large volume, for example (because the system needs to push the atmosphere out of the way), but rewards a large entropy (because it provides many more arrangements, one of which we're more likely to observe). So we can already obtain useful predictions; for example, the pressure–volume aspect indicates that higher-density, lower-volume phases must be the more stable phases at higher pressures, which is confirmed by experiment. Essentially everything solidifies at sufficient pressure.
But our focus is temperature. Similar reasoning predicts that everything boils or gasifies at sufficient temperature. Here's a plot of $G$ for the phases of a typical simple material (such as water) at different temperatures:
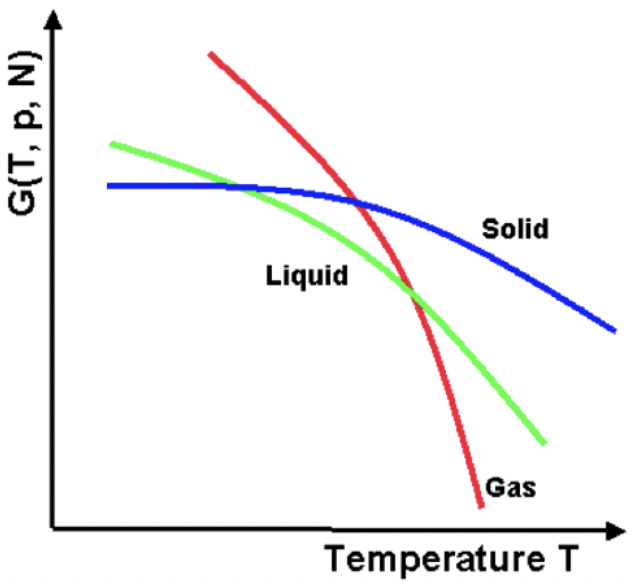
Condensed matter—solids and liquids—have a lower internal energy than gases because they're well bonded (which releases energy), and solids are the better bonded of the two. So at low temperatures (small $TS$ term), solids win the race for lowest Gibbs free energy. At high temperatures, high-entropy gas wins because of that temperature coefficient. This is consistent with the familiar transition of melting and then boiling upon heating, condensation and then freezing upon cooling.
So far, we've been talking about materials at atmospheric pressure, and this is consistent with our definition of boiling: the temperature where the vapor pressure matches the atmospheric pressure, recognizable as the temperature where vapor bubbles can push water out of the way and buoyantly rise in boiling liquid.
However, the vapor pressure of water can certainly be less than the saturated pressure (which is the equilibrium pressure in a closed space over the liquid phase). The possible range is quantified by the relative humidity over a scale of 0–100%. Open water spontaneously evaporates until the relative humidity is 100% or the liquid is depleted.
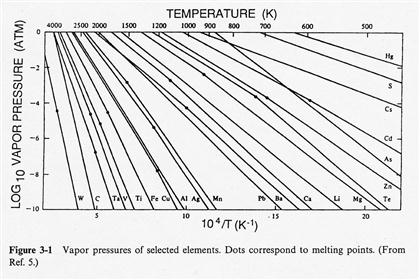
(Skip this paragraph if it's too math-intensive.) To show this, we can look at the Gibbs free energy change for constant temperature and amount, which in differential form is $dG = V\,dP$. Applying the ideal gas law $PV=nRT$, we have $dG=\frac{nRT}{P}dP$, which we integrate to obtain $\Delta G=nRT\ln\Delta P$ or $\Delta P=\exp\left(\frac{\Delta G}{nRT}\right)$. $\Delta G$ is negative for a gas relative to a liquid, so we find that the vapor pressure increases exponentially with increasing temperature. Remarkably, this is true for all the elements:
The summarized explanation for condensation is thus:
Water vapor exists in our atmosphere, quantified by the relative humidity, nearly always less than the saturated vapor pressure. This saturated pressure can be calculated from basic material properties of the different phases and reflects a tradeoff between strong bonding (low internal energy), density (low volume), and many possible arrangements (high entropy), mediated by the surrounding pressure and temperature.
The equilibrium vapor pressure is an exponentially increasing function of the temperature. When moist air is cooled by an adjacent object, the water content stays the same, but the relative humidity rises because it's measured relative to the saturation temperature, which as dropped. Essentially, Nature is less interested in the favorably high entropy of the gas phase because the temperature has dropped in the $TS$ term. Nature is now more interested in the $U$ or internal energy term, as forming bonds between the water molecules would release energy and decrease $U$.
So condensation becomes spontaneous as we cool through the dew point of 100% relative humidity. The water vapor is of course depleted during this process, but in practice it's replaced by fresh moist air that's ready to cool and condense out its moisture. Note that much of the energy released from condensation goes into heating that cool drink, ultimately driving an equilibrium of uniform temperature and 100% or lower relative humidity. To avoid this heating, you could suppress condensation with one of those koozies.
