I am reading "An Introduction to Mechanics" by Daniel Kleppner and Robert Kolenkow.
The sketch shows a simple pendulum of length $l$, with mass $M$, and corresponding weight $W=Mg$.
The mass moves in a circular arc in a vertical plane. Denoting the angle from the vertical by $\theta$, we see that the velocity is $l\frac{d\theta}{dt}$ and the acceleration is $l\frac{d^2\theta}{dt^2}$. The tangential force is $-W\sin\theta$. Thus the equation of motion is $$Ml\frac{d^2\theta}{dt^2}=-Mg\sin\theta$$ or $$\frac{d^2\theta}{dt^2}+\frac{g}{l}\sin\theta=0.$$
I don't understand the above solution.
The mass doesn't move in a straight line. (The mass moves in a circular arc.)
But the authors derived the differential equation like the above.
Let $s$ be the length of the curve from the vertical to the mass.
Then $s=l\theta$.
Then $$M\frac{d^2s}{dt^2}=Ml\frac{d^2\theta}{dt^2}=-Mg\sin\theta=-Mg\sin\left(\frac{s}{l}\right)$$ holds.
My question is the following:
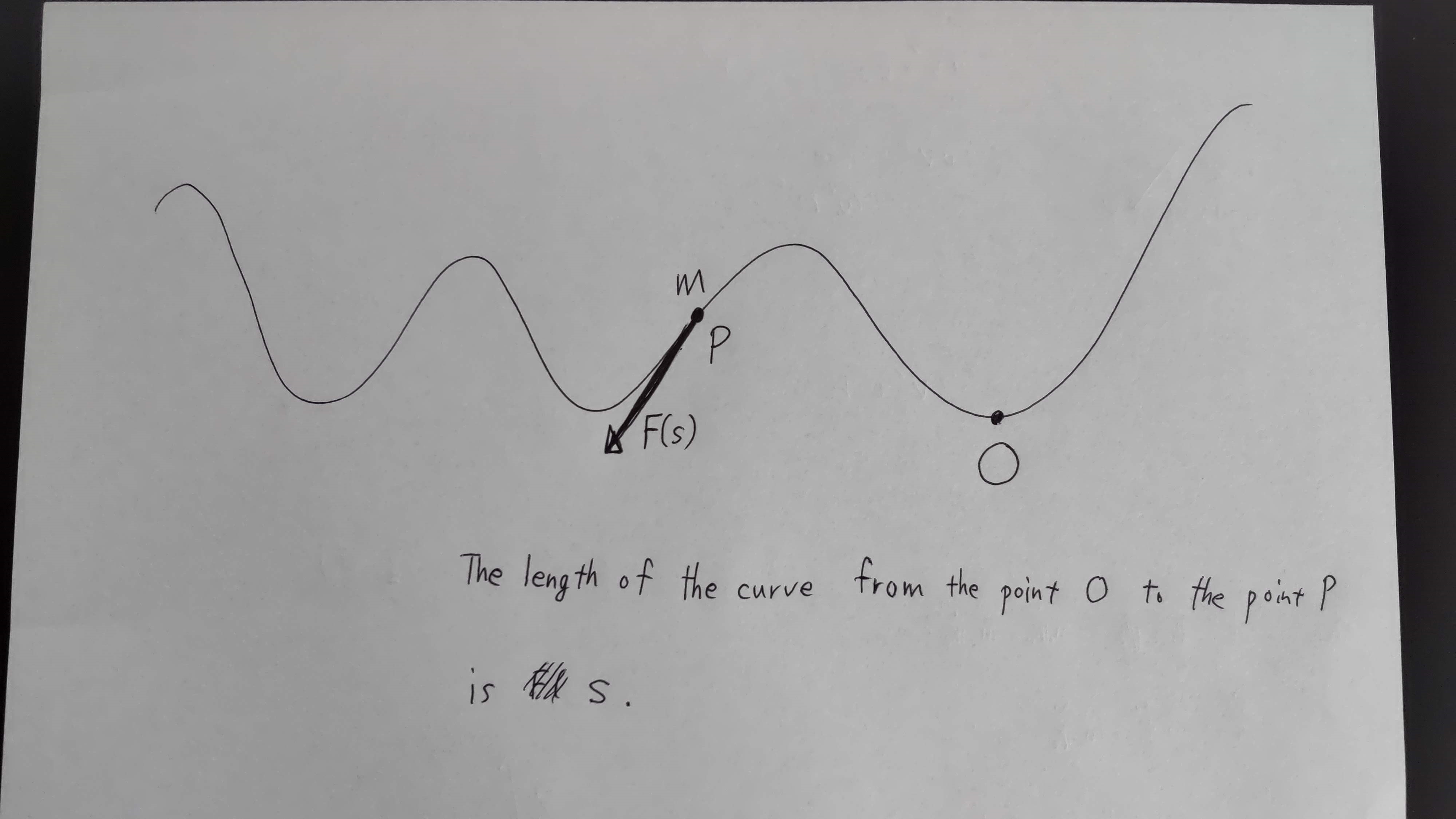
Please see the above figure.
The mass $m$ is at the point $P$.
Suppose $s$ is the length of the curve from the point $O$ to the point $P$.
Suppose the tangential force at the point $P$ is $F(s)$.
Does $$m\frac{d^2s}{dt^2}=F(s)$$ hold?