Explaining Newton's Laws of motion to a 6 year old

Question

An old professor of mine once said that an effective means to get people interested in Physics is to get them started early.

What would be an effective and meaningful (and fun) means to explain Newton's 3 laws of motion to a 6 year old?

Answer 1

I'd like to continue with Mark Betnel's answer. Children at this age vary enormously in their abilities, so I believe I am not too out of place saying that both Mark's answer and my answer are general rules, not applicable to all children. I do have a bit to do with primary school science and serve on the education committee at my daughter's primary school, where I do meet with several education researchers and get to hear their insights and I must say Mark's answer rings very true with my observations of my own children and other children who come to our school's "science room".

To teach children of this age Newton's laws fully is far too big an undertaking, and something that is likely to grab the imagination of very few children at this age. However, as Mark says, they are beginning to form common sense (Einstein's immortal quote "Common sense is the collection of prejudices acquired by age eighteen" is pertinent here) notions that will hinder them later on, so there are some things about motion that you might try to get across. The idea of getting children to ask questions and putting questions to them is going to be far more effective than a didactic approach. So here are some suggestions and background further to Mark's answer. Let me begin with a little poem I wrote for my daughter when she was indeed six years old as it holds the notions that I think you can concentrate on.

'Most everything one lays put, stays put,

'Most everything a-go stays a-go,

When do these rules not hold?

Mr. Newton was so bold

To say his second law is how we know.

I begin with a little ditty to illustrate something our little people find very compelling at this age: playful and slightly nonsensical language. This is the very age where the "higher" language abilities like figurative description, metaphor and allegory are beginning to take root, particularly when these are grounded in the human relationships important in the child's life. I will come back to this idea several times.

Another thing to bear in mind about this age that education research shows: this is the age where children are still learning that systems can have abstract properties aside from the "outward" ones like colour and shape. If you show a five year old a bottle full of water, then tip the water into several different cups, it can be shown in pschological experiments that most children of this age cannot foretell that the quantity of water in the cups will fill the bottle back to the same level it was at before. However, by the age ten, they can foretell this. What's going on here is they child learns that a quantity of stuff has an abstract extrinsic property "volume": it doesn't matter how you split this up and put it back together, or what sequence of shapes it morphs through, when you put the stuff back into its original container, it still takes the "same amount of space up". So this is the very age when the child is understanding "properties" both extrinsic and intrinsic. At six, therefore, the constant of proportionality in Newton's second law i.e. mass is likely to be utterly meaningless to most of them.

Overview: Changes of States of Motion as Interactions

Whatever you teach about Newton's laws, the overall idea I think you can seek to get across is that "interactions change states of motions". If something is "minding its own business" and not interfering with something else, it will stay as it is. It's when "things get together and do things to one another" that their motion changes. I would try this kind of language in quotes - little people are imagining inanimate things alive and bringing to life little stories and allegories everywhere, so they will imagine balls bumping from the ball's standpoint, as though it were alive.

'Most Everything One Lays Put, Stays Put

This is one aspect of Newton's first law of course, and this is utterly and altogether self evident to a six year old. You can put a ball on a shelf in a cupboard and ask a child whether it would still be there when you open the cupboard up again and all six year olds will know what will happen. The first line of my poem needs no further explanation.

'Most Everything A-Go, Stays A-Go

This is the one that of course challenge their "common sense". Mark's idea of asking a child why they think a ball stops when the shove stops is a good one, letting the question bake for a while and encouraging speculation is a good one. The idea one must get across here is friction. Further to Mark's idea of a hockey puck I would try to get some dry ice or air pucks and show the children how they glide around on their tables so smoothly and easily. It then becomes much easier to imagine the ideal case without friction.

There is also a story that many children will find highly compelling here and that is the tale of Voyager I. Of course, this spacecraft has be coasting for nearly forty years. Tell the children how far away it has gotten, ask them to imagine how much fuel they think it would need to travel that long, and slowly let it sink in that it really is just coasting without any help whatsoever!

The Second Law

This is the one that I think you likely need to leave alone the most. The mass - extrinsic, abstract property as discussed above - and proportionality relationships are likely beyond what most six year olds will grasp. I think the second law should mostly be emphasized as the negation of the first law: things deflect, start and stop moving when the are NOT "minding their own business" and when they ARE "doing things to one another".

However, children do understand "force": shoving, weight, pushing and so forth. They will probably have some concept of the harder you shove something, the faster it goes (what we're talking about here is impulse rather than force) and the faster something goes the longer, harder you have to shove against it to stop it. There is the risk here of course that the concept of $F=m v$ rather than $F= d_t(m v)$ will take root, which is why it is likely best to leave the second law out of the picture aside from being the negation of the first.

Something that might be worth trying is getting the children to push a goodly size toy wagon around with varying quantities of sand and ask them how hard it is to stop it once it gets moving. This is something that a child learns "kinetically": they may not have noticed before that it's exactly when something is hard to get going that it is also hard to stop once going.

The Third Law

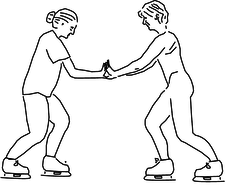
How do we teach children this one - the subtlest of the three? Actually, the six year old mind can grasp this at one level really well through the following experiment. My picture speaks better than words:

Two toy carts or wagons that run really freely and a hall with a smooth floor. Two children launch themselves off one another. Play a game: can you push the other child away without starting yourself rolling, if you can't "cheat" by steadying yourself with your feet (as my son is doing here!)?

It really needs no further explanation. I suspect "feeling" the third law like this at this age is quite compelling: and the words to describe it by are "you can't shove something else without its shoving you back", something that they learn deeply in the little game I just described.

Answer 2

It's important to be aware of the limitations and strengths of your audience. A typical 6 year old is unlikely to have any interest at all in Newton's laws per se, nor the ability to follow an explanation, a mathematical model, or to appreciate the distinction between, for example, a linear progression and a quadratic one. The sophistication to handle those details begins to develop in middle school, and is not yet complete even for many college students. If your aim is merely to get them interested in physics, your strategy should not be didactic.

On the other hand, 6 year olds are fantastic observers and question askers, since they have none of the fear of being wrong that prevents older students from challenging themselves. They have also already begun to form common sense generalizations that will be a serious impediment when they do begin to study physics more formally.

So when they notice that a ball pushed into motion always stops soon after the push stops, encourage them when they ask "Why did it stop?" Let them speculate. Let them notice that friction is ubiquitous in their everyday experience, always call it out whenever it is a relevant factor, and occasionally ask them to imagine what might happen if it wasn't there. Take them to an ice rink and let them notice the difference between a hockey puck's behavior on the ice and on the deck next to the rink.

Put some paint in a box, drop a marble in it, and let them move the box around to make the ball roll, forming patterns in the paint. Encourage them to plan what the painting will look like. Ask them to compare the result to their plan.

Put them on a skateboard or bicycle and ask them to predict where a dropped ball will land when the bike is moving -- teenagers will always say "behind you", but a 6 year old might not, and you can quickly do the experiment and ask them to consider why it lands at their feet, and also why it stops moving forward with the bike soon after it hits the ground.

When they get hurt playing baseball or soccer (and they will), after you have comforted them, talk about the sting of the ball, and compare it to other hurts they have experienced. "Was that tennis ball worse than the toy truck your sibling threw at you? Why?"

Let them imagine. Help them test their imaginations. And when they are ready, but not before, help them explore new and more powerful ways to describe their imagination, and those tests. Help them channel their natural scientist, and when they are ready, they will tell you everything you want to know about Newton's laws.

Answer 3

If you have a good camera you could take multiple and quick snapshots of

1)Objects in Uniform Motion(For first law). Show him that the distance traveled by a ball in a unit snapshot is constant.

2) Objects Accelerated motion(For second law, you could use Gravity). Show him that the distance travelled by a falling object in each shapshot increases Linearly.

You could also play around with springs, and balls of different masses.

3) Collisions(Of carrom coins, or marbles perhaps, equal mass). Then illustrate that combined distance traveled by both the Objects in each snapshot interval remains the same before and after collision.

You could then experiment with variable masses, and Show that d1 + 2*d2 remains constant, if the second object is twice massive.

Maybe you can develop the idea, further. This is one way.

Answer 4

Probably demonstration is better than explanation. The trick, I think, is finding slick surfaces.

An ice rink might do, or a smooth floor on well-greased wheels. Air hockey tables remove friction with pneumatics. Newton's laws of motion are intuitive, I think, in a frictionless environment. (This is why "driving on ice" is so often invoked by adults to get other adults thinking right.)

Once you've got that you can show how

1. continually applied constant pressure differs from "slaps"
2. hockey pucks keep gliding after you hit them once
3. pushing the child once, it continues to skate forward in a straight line
4. (you might want to use a spring to demonstrate constant force/acceleration and show that pull = push)
5. (so a spring pulling at constant stretch = constant force = acceleration, not staying the same!)
6. pushing on a wall shoves you backwards
7. pushing on a heavy person on wheels shoves both backwards … the lighter person going farther/faster
8. skating into a ball, sends the ball forward and slows you down
9. like a white ball hitting a coloured ball on the billiards table
10. what makes the energy transfer more efficient, anyway? (time for hypothesis testing, experiment design, and falsification!)

A really nice capstone would be push the child on an office chair on ice, then have it fire off a shot of something heavy to the side, therefore angling \ the path … this would demonstrate all three principles at once.