Why can't entropy pile up?

Question

I need help to interpret the following paragraph from Kittel and Kroemer, Thermal Physics 2ed, page 228-229:

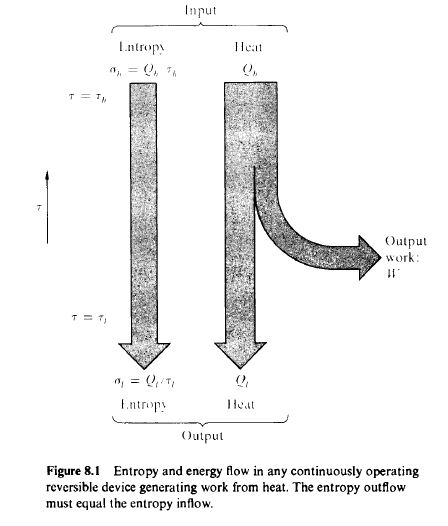
Work can be completely converted into heat, but the inverse is not true: heat cannot be completely converted into work. Entropy enters the system with the heat, but does not leave the system with the work. A device that generates work from heat must necessarily strip the entropy from the heat that has been converted to work. The entropy removed from the converted input heat cannot be permitted to pile up inside the device indefinitely; this entropy must ultimately be removed from the device. The only way to do this is to provide more input heat than the amount converted to work, and to eject the excess input heat as waste heat, at a temperature lower than that of the input heat (Figure 8.1). Because $\tfrac{\delta Q}{\sigma}=\tau$, the reversible heat transfer accompanying one unit of entropy is given by the temperature at which the heat is transferred. It follows that only part of the input heat need be ejected at the lower temperature to carry away all the entropy of the input heat. Only the difference between input and output heat can be converted to work. To prevent the accumulation of entropy there must be some output heat; therefore it is impossible to convert all the input heat to work!

Especially, two sentences are unclear to me:

(1) "A device that generates work from heat must necessarily strip the entropy from the heat that has been converted to work."

What does it mean to "strip the entropy from the heat"?

(2) "The entropy removed from the converted input heat cannot be permitted to pile up inside the device indefinitely"?

Why the "The entropy removed from the converted input heat cannot be permitted to pile up inside the device indefinitely"

Answer 1

What Kittel & Kroemer are quietly saying is that "heat" does not convert to work, only work converts to work, if one uses a conventional meaning of the English word "convert". The reason for this is that the total entropy in all, yes all, thermodynamic transformation can never decrease. In other words, entropy is indestructible the same way as gravitating mass or electric charge are but with the difference that if it does not stay the same then it increases.

If you assume that heat converts to work then it should follow that the entropy being carried with thermal energy representing "heat" somehow disappears for work has no entropy. Since that is not allowed, Clausius introduced the concept of simultaneous heat compensation, a separate irreversible process to restore that disappearing entropy in the working fluid before rejected at a lower temperature.

What Kittel & Kroemer are quietly saying with "A device that generates work from heat must necessarily strip the entropy from the heat that has been converted to work" is that Clausius is wrong about that "heat compensation", there is no compensation, instead the entropy in the thermal energy is, by some magic, removed from it and it is the same entropy that is to be rejected at the lower temperature. Kittel & Kroemer do not explain that if that is indeed the case then what is being converted in the first place, and how does the converted heat become work.

There is one consistent explanation to all that is to view the entropy and not heat as being the agent of the work performed. No conversion, just entropy transport from a higher to a lower temperature, the same way as a gravitating mass dropped from a higher gravitational potential to a lower one performs work.

And to your question why entropy cannot pile up, is because you are interested in a cycle, and you want to return the engine to its starting state, and whatever entropy was absorbed at the higher temperature it is rejected at the lower one along with any irreversibly generated excess entropy (say, from friction or conduction).

Answer 2

Heating shifts entropy; doing work via an impermeable barrier (e.g., expansion work) doesn't.

Therefore, energy that participates in the former and then the latter is automatically separated from the associated entropy. This is the "strip[ping]" being referred to.

Why can't we have entropy pile up indefinitely?

We do; it piles up in the cold reservoir or in the engine. For a cyclic heat engine that must return to its original state every cycle, only the former remains as a possible entropy depository.

Answer 3

Why is this the case? Why can't we have entropy pile up indefinitely?

What Kittel & Kroemer are referring to is entropy cannot "pile up" over a complete heat engine cycle. The reason is entropy is a system property and has only one value at any equilibrium state. By definition, a cycle returns a system to its original equilibrium state and thus the change in entropy of the system after completing a cycle is necessarily zero. Thus any entropy a system acquires during some process in the cycle must be removed (discarded to a lower temperature reservoir) in the form of heat, to return the system to its original state.

For example, in the Carnot Cycle for an ideal gas the system acquires entropy during the reversible isothermal expansion when heat is taken in and work is performed equal to the heat taken in. The entropy acquired during the process is temporarily kept in the system. In order to return the system entropy to its original value at the start of the cycle, a reversible isothermal compression is performed to transfer heat (and the acquired entropy) out of the system and to a low temperature reservoir. That "waste heat" is not available for work.

Hope this helps.