Vertical manometer
Consider a manometer filled with water. Given a pressure $p_0$ at height $y=h$, The formula for the pressure $p$ at $y=0$ is $$ p = p_0+\rho g h $$ One way to derive this is to consider the slug of liquid/gas above the fluid at $y=0$, which has mass $\rho h A$. The pressure force at $y=0$ is the static pressure plus the pressure needed to balance this force.
Tilted manometer
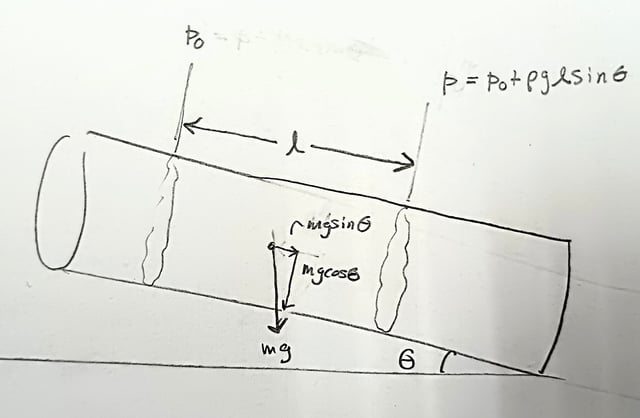
For a manometer tilted at angle $\theta$, the change in height is instead $l\sin\theta$, so the pressure is $$ p=p_0+\rho g l \sin\theta $$ where $l$ is the length of manometer going off at angle $\theta$ from $y=0$.
I was trying to derive this formula by considering the "slug of mass" above the fluid at $y=0$. This mass is $$ m=\rho l A $$ The gravitational force acting at the center of this slug of fluid/gas is $$ W = \rho g l A $$ This gravitational force can be decomposed into a force acting axially along the manometer and adding to the fluid pressure, and a force perpendicular to the lower manometer surface.
$$ W_{axial} = \rho g l A \sin\theta $$ $$ W_{perp} = \rho g l A \cos\theta $$
Question
My question is, what do we do with the force component acting perpendicular to the manometer? It's a fluid force that acts on the lower, inner surface of the manometer, which makes me think it should somehow add to the pressure. But for a given height, the pressure in a static fluid is the same everywhere.
Somewhere, my reasoning is breaking down. I'd appreciate some help to understand where I'm going wrong.