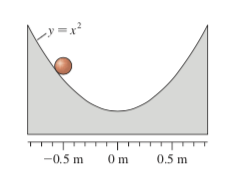
Is this because the surface 'comes up against' the object due to it's curved nature
1
-
1$\begingroup$ The flat surface has a fixed angle $\vartheta$, and the normal force depends upon that. For the parabolic surface this angle changes, and so the normal force changes along with it. $\endgroup$– naturallyInconsistentCommented May 11, 2023 at 13:56
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Yes, but the thought behind your intuition need to be spelled out.
A rigid object does not deform when you push on it. If you push on it, it pushes back with a reaction force just hard enough to prevent deformation. If an object exerts a force on a surface, the reaction force is just hard enough to prevent the object from moving into the surface.
This force does not prevent sideways motion. That would be a friction force. Both ice and concrete are rigid and exert a reaction force. But concrete also exerts a friction force. Note that friction may prevent sliding, but not rolling.
On a flat horizontal surface, the normal force is equal to the weight of the object.
On a tilted flat surface, weight is vertical and the normal force is perpendicular to the surface. You have to break the forces up into components. We will choose components normal and parallel to the surface.
$$\vec F_{net} = \vec w + \vec F_{norm}$$
$$= \vec w_{parallel} + \vec w_{norm} + \vec F_{norm}$$
The angles do not change as the object rolls downward, and so $\vec w_{parallel}$ and $\vec w_{norm}$ do not change. The surface adjusts $\vec F_{norm}$ to be just enough to cancel $\vec w_{norm}$. $\vec F_{norm}$ is constant.
$$\vec F_{norm} = - \vec w_{norm}$$
Also
$$\vec F_{net} = m \vec a_{net} = \vec w_{parallel}$$
The forces add up to a constant net force and the object accelerates uniformly parallel to the surface. As long as the object stays in contact with the surface, the velocity is always parallel to the surface. In this case, velocity is in the same direction as the force.
$$\vec F_{net} = \vec F_{parallel} $$
For a curved surface like a parabola, it is the same idea but the angles do change. As the object rolls downward, you have to figure out a new angle for each point.
$$\vec F_{net} = \vec w + \vec F_{norm}$$
$$m \vec a_{parallel} + m \vec a_{norm} = \vec w_{parallel} + \vec w_{norm} + \vec F_{norm}$$
We can write the components separately.
$$m \vec a_{norm} = \vec w_{norm} + \vec F_{norm}$$
$$m \vec a_{parallel} = \vec w_{parallel}$$
Because the velocity changes direction, there must be a component of $\vec a$ perpendicular to $\vec v$.
You may need to think about that a bit to convince yourself. Keep in mind that if $\vec a$ is always parallel to $\vec v$, the object speeds up or slows down. But the change in velocity is always in the same direction as the velocity.
If $m \vec a_{norm} \ne \vec 0$,
$$\vec F_{norm} = m \vec a_{norm} - \vec w_{norm}$$
The surface must push back on the object hard enough to
- Oppose the component of weight that tries to push the object into the surface
- Change the direction of the object's motion.
As you more or less said, the object comes up against the surface due to its curved nature.
answered May 11, 2023 at 15:14
$\begingroup$
$\endgroup$
If you have learned circular motion then this explanation may be helpful.
Imagine replacing the parabolic surface by a circular surface, this ball will go through circular motion and has centripetal acceleration pointing to the center. According to Newton's 2nd law, the acceleration should be caused by a force and in this case the only possible force pointing to the center is normal force so extra normal force is required to keep the mass in circular motion.
Now in your case, the curve is not a circle but a parabola, but there is no difference. At each point, you can find a circle tangent with the parabola and treat the parabolic motion as a connection of a lot of small circular motions. This idea is pretty like take derivative where we can find tangent lines at each point. Here I attached a image from Wikipedia illustrating this idea.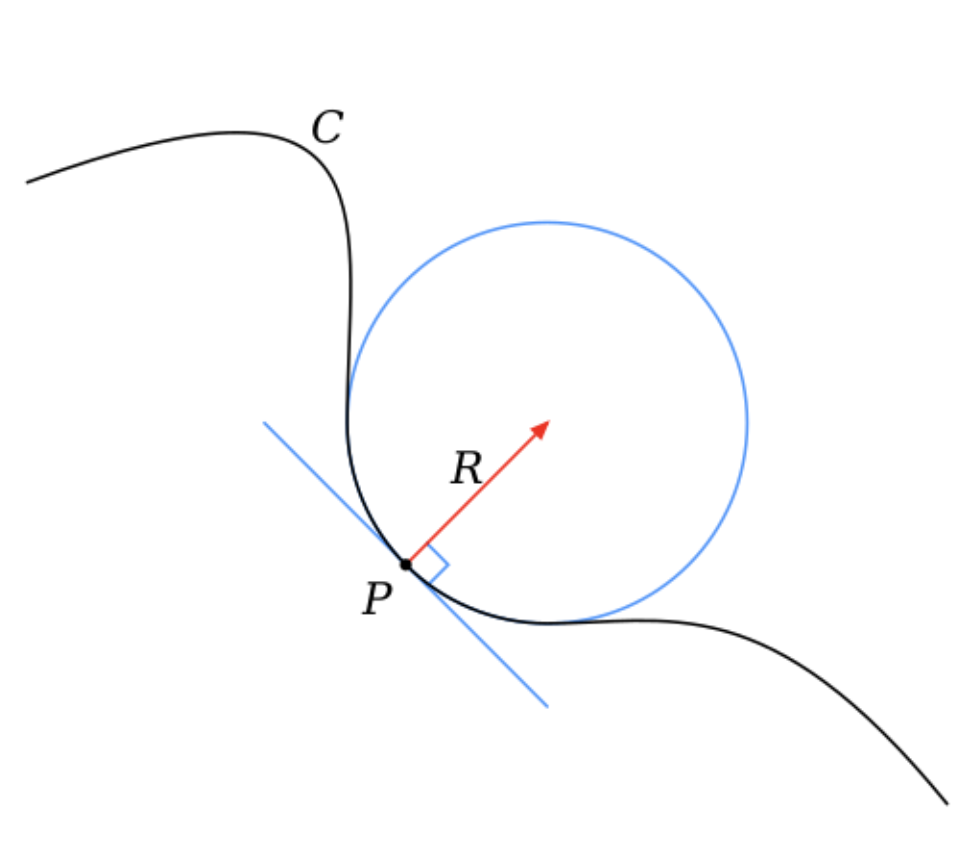
Going back to the fundamental, Newton's 2nd law says that the acceleration is probational to the force $F=ma$. In a curved motion, the direction of the velocity is changing, which induce an acceleration $a_{d}$. This acceleration induced by the changing of direction is along the normal direction and that's why you have extra normal force in this case.
$\begingroup$
$\endgroup$
Two forces act on the object; gravity and the normal force. From Newton's second law, we know that the acceleration $a$ of the object must obey $$ ma = F_\mathrm{total} = F_\mathrm{gravity} + F_\mathrm{normal}.$$
If the object slides along a flat frictionless surface, its acceleration is zero. Therefore the two forces must exactly cancel each other, and we have $F_\mathrm{normal} = - F_\mathrm{gravity} $.
This is not the case when the object travels on a parabolic surface, because to stay on a parabolic surface the object must accelerate. The magnitude of the acceleration will depend on the speed of the object and the curvature of the parabola, but we can certainly say that its vertical acceleration must be upward. The gravitational force is the same as before, so the additional force required to make the object accelerate upwards must come from the normal force. Therefore we can deduce that the magnitude of the normal force must be greater for an object moving on a parabola compared to an object sliding on a flat surface.
