For orbits in the Schwarzschild metric, then for a given value of the specific angular momentum, the effective potential experienced by a body with mass has two turning points - see for example the effective potential below for $L/m = 4.02 GM/c$, where $M$ is the mass in the Schwarzschild metric.
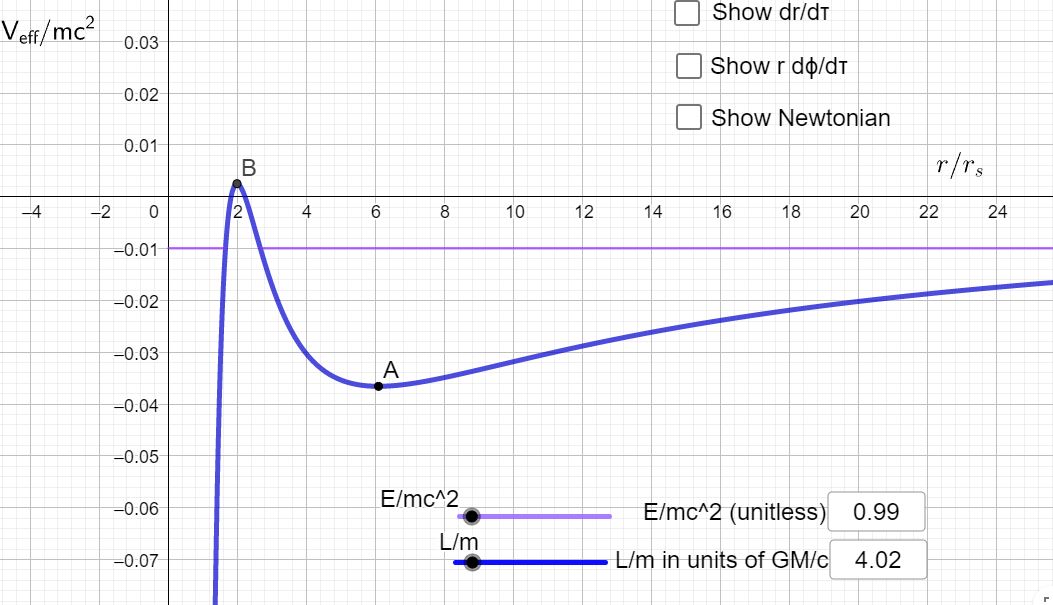
This example comes from a Geogebra widget I created. You can play with this and confirm that the minimum of the effective potential (if it exists) is at $3 < r/r_s < \infty$ and that the maximum is at $1.5 < r/r_s < 3$. The smallest value in the latter case is achieved as $L/m \rightarrow \infty$.
The maximum in the potential at point B represents the radial coordinate of an unstable circular orbit. The specific energy must achieve a value such that the body sits exactly at the peak of the potential; in which case $dr/d\tau = 0$ and the orbit is circular.
If the energy is increased then any perturbation to the motion inwards or outwards will result in the body either falling into the black hole or escaping to infinity respectively. i.e. The orbit is unstable.
Since the unstable circular orbit can achieve a smallest value of $r = 1.5r_s$ for $L/m \rightarrow \infty$, then it possibly makes sense to call this the innermost unstable circular orbit. A stationary "shell observer" at $r=1.5r_s$ would measure the speed of the orbiting body $\rightarrow c$.
The paper about tachyons is arguing that the peak of the effective potential for bodies with imaginary rest mass can occur at values $1 < r/r_s < 1.5$ and thus $r=r_s$ would be the innermost unstable circular orbit for such tachyons. The energy of a tachyon in such a circular orbit would be zero - and hence a "transcendent tachyon".
Appendix (some details)
The radial motion of a massive body in the Schwarzschild metric can be written:
$$ \frac{1}{2}\left(\frac{dr}{d\tau}\right)^2 = \frac{c^2}{2}\left(\frac{E^2}{m^2c^4} -1 \right) - \frac{c^2}{2}\left[\left(1 - \frac{r_s}{r}\right)\left(1+\frac{L^2}{m^2r^2c^2}\right)-1\right]\ , $$
where $E/mc^2$ and $L/m$ represent the energy and angular momentum of the orbiting body as measured by an inertial observer at $r \gg r_s$.
The second term on the right hand side is known as the effective potential and it can be differentiated to find turning points at
$$ \frac{r}{r_s} = \frac{L^2}{m^2c^2r_s^2} \left[ 1 \pm \left(1 - \frac{3m^2c^2 r_s^2}{L^2} \right)^{1/2} \right] \ . $$
If the dimensionless ratio $L^2/m^2c^2r_s^2$ becomes very large, then the smaller root becomes $r/r_s \rightarrow 3/2$ (use a binomial approximation on the bracket).
The speed of the circular orbit at a given radial coordinate, as measured by a stationary (in space) observer at that radial coordinate is given by
$$ v_{\rm shell}^2 = c^2 \left(\frac{r_s}{2r -2r_s}\right) $$
and $v_{\rm shell} \rightarrow c$ as $r \rightarrow 1.5r_s$
You can use the same equations for tachyons with imaginary $m$ and find that the innermost unstable circular orbit occurs when $L^2/m^2c^2r_s^2 = -1$ at $r =r_s$ and the first equation above shows that $dr/d\tau = 0$ (a circular orbit) if $E=0$.

