What is the missing part of the argument needed to justify the claim of (2.52) in Peskin and Schroeder's QFT textbook?

Question

[This paragraph has been added to make clear that this is not a homework question having been branded as such by a mod of some kind. The question is attempting to the core of a very important question relating to causality in Quantum Field Theories. The whole point of QFTs (or at least one of the main points) is that they are able to be causal and local. The causality is suppose to flow from the vanishing of commutators of observables outside the light-cone -- and so looking at propagators of field operators outside the light-cone (which nearly vanish but don't quite vanish) is a precursor to that. It is therefore significant that Peskin and Schroeder have a big hole in one of the most important arguments in their book. At exactly the point where they could/should be demonstrating how these terms become exponentially small, they neglect to discuss an issue which (from my investigations) seems capable of derailing their argument. I claim, therefore, that my question is relevant to anyone who wants to trust arguments about causality in Pesikin and Schroeder. ]

Peskin and Schroeder (P&S for short) try to argue on p.27 in section 2.4 of their Introduction to Quantum Field Theory that the propagator (2.52) of two Klein-Gordon Fields at different positions $x$ and $y$ is non-zero but exponentially small when $x$ and $y$ are separated by a spacelike interval.

It has always seemed to me that part of their argument has a big hole in it, however, and I would like to see that hole closed by a good answer to this question.

A related question elsewhere on this site yielded an answer ( https://physics.stackexchange.com/a/557390/362800 ) which touches upon half of the issue I am concerned with, but does not address all of it. In any case the question it was answering was somewhat different to mine. Other related questions such as Why is it necessary to wrap our contour around the branch cut at $+ im$ in the spacelike Klein-Gordon propagator? (P&S) and Contour for Klein-Gordon field transition amplitude don't mention my concern at all. Hence I am asking a new question here specifically on the issue which concerns me.

I will summarise the Peskin and Schroeder argument as follows:

They begin (in effect) by motivating interest in a function which I will call $f(p)$ of a real variable $p$, and which also depends on two positive real parameters $m$ and $r$. Since $m$ will always be constant but $r$ is sometimes varied, $r$ appears in some of the subsequent notation as a function parameter whereas $m$ never does.

$$f(p)\overset{\mathrm{def}}{=}\frac{-i}{2(2 \pi)^2r}\frac{p e^{ipr}}{\sqrt{p^2+m^2}}\qquad\text{(for $m,r>0$)}\qquad(1)$$

The above $f(p)$ is then integrated along the real line to generate a quantity which I will call $D_0(r)$ in (2) below. It is this $D_0(r)$ which is P&S's field propagator, and it is therefore this quantity which they want to show goes like $e^{-mr}$ as $r\rightarrow\infty$.

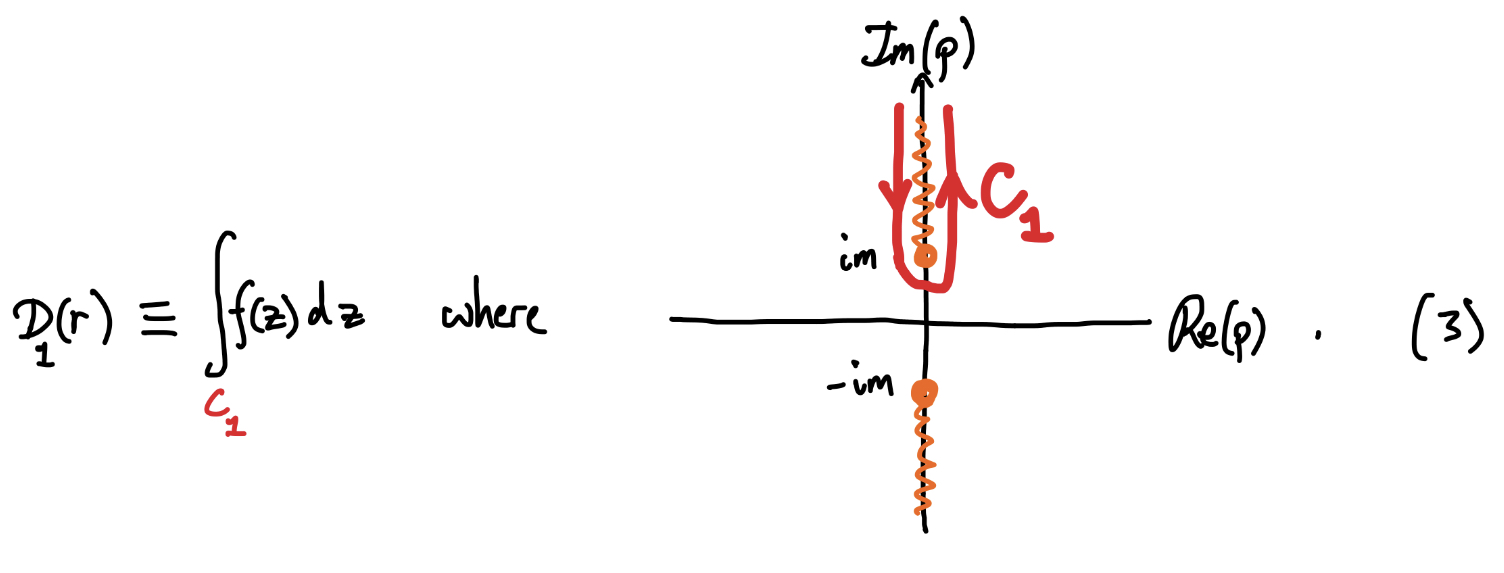
Equation in (2) below shows how P&S define this quantity $D_0(r)$ and how they immediately (and correctly) note that it can also be evaluated as a contour integral along the path $C_0$ which is shown in the image below to run along the whole of the real axis (and thus passes between two branch points at $\pm i m$) :

The somewhat dodgy step which then follows consists of P&S then claiming (or sort of claiming) that all we need to do is `push the contour up on either side of the upper branch cut' so that all we need to do is evaluate the quantity which I have called $D_1(r)$ below along the different curve $C_1$ also shown below in (3):

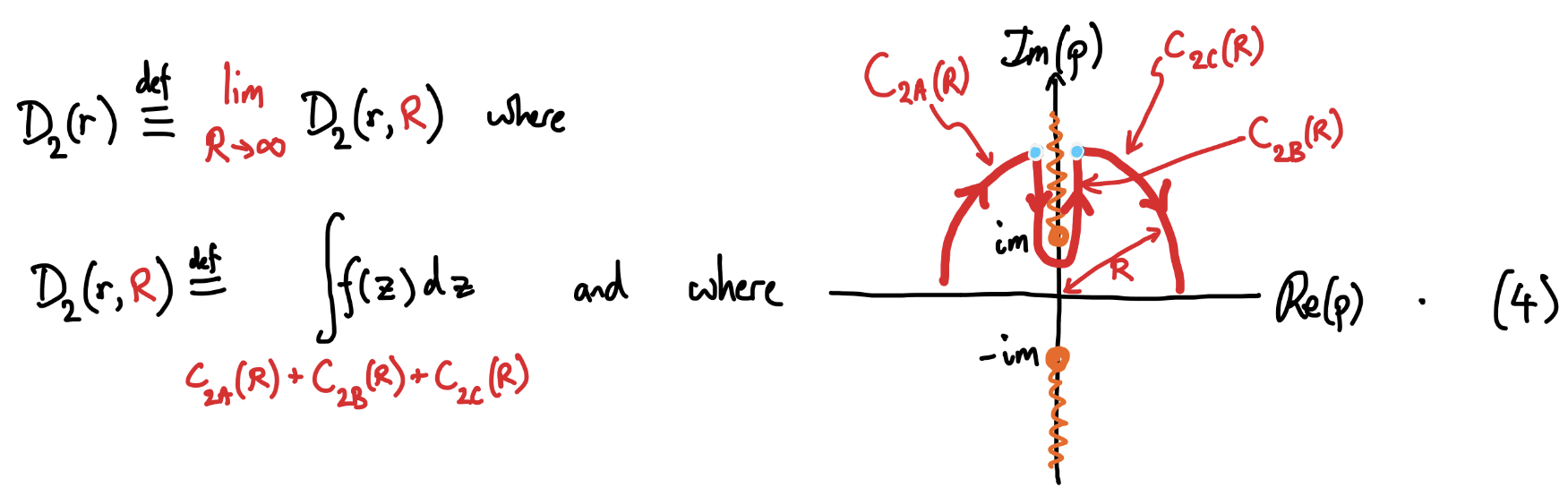
The reason I call the above step a bit dodgy, is that all my training in contour integration has taught me that there is no reason, in principle, why the integral along $C_1$ should equal the integral along $C_0$ as they have different end points. What my training tells me is that if one wants to push the contour up onto either side of the branch cut, then one should actually distort it to a more complex shape such as the one shown in the following diagram:

At fixed $R$ the above curve has three parts: first an arc $C_{2A}(R)$, then a central branch-cut-sandwich $C_{2B}(R)$ and a second arc $C_{2C}(R)$. Furthermore the idea is that we can increase $R\rightarrow\infty$ to recover a curve $C_{2A}+C_1+C_{2C}$ that should have the same integral as $C_0$.

Since P&S neglect to ever mention the arcs $C_{2A}$ and $C_{2C}$, and since they use a claim (2.52) that

$$D_1(r)\underset{r\rightarrow\infty}{\sim} e^{-mr}\qquad(5)$$

to justify their higher/ultimate goal of claiming (in the text surrounding and following their (2.52)) that:

$$D_0(r)\underset{r\rightarrow\infty}{\sim} e^{-mr}\qquad(6)$$

we can infer that they must believe that the integrals along $C_{2A}$ and $C_{2C}$ result in contributions to $D_0(r)$ which are `small enough' that they don't conflict with (6).

But rather than take that idea on trust, can we prove it ourselves? I.e. can we prove that the statement voiced in (7) below?

$$D_{2AC}(r) \overset{\mathrm{def}}{=} \lim_{R\rightarrow\infty} D_{2AC}(r,R)\lesssim e^{-mr}\qquad(7)$$ where $$D_{2AC}(r,R) \overset{\mathrm{def}}{=} \int_{C_{2A}(R)+C_{2C}(R)} f(z)dz.\qquad(8)$$

The lack of a proof or good argument for (7) is the hole I wish to see plugged!

Usually when trying to show that integrals on large arcs are irrelevant, the go-to theorem is Jordan's Lemma (https://en.wikipedia.org/wiki/Jordan's_lemma). However it is not much help here. It only tells me that:

$$\left|D_{2AC}(r,R)\right |\le \frac{\pi}{r} \max_{p\in C_{2A}(R)+C_{2C}(R)}{\left|{\frac{p}{\sqrt{p^2+m^2}}}\right|} =\frac{\pi}{r} \frac{1}{2(2\pi)^2r}{{\frac{R}{\sqrt{\left|{R^2-m^2}\right|}}}}\qquad(9)$$

and that therefore:

$$\left|D_{2AC}(r)\right| \le \lim_{R\rightarrow\infty} \frac{\pi}{r} \frac{1}{2(2\pi)^2r}\frac{R}{\sqrt{\left|{R^2-m^2}\right|}} =\frac{\pi}{2(2\pi r)^2}\qquad(10)$$

which only shrinks like $1/r^2$ which is slower not faster-than-or-equal-to the desired $e^{-mr}$. This doesn't mean that P&S is wrong, though, it just means that Jordan's Lemma is not (on its own) providing a strong enough bound. (Aside: the first comment on an answer https://physics.stackexchange.com/a/94098/362800 to a related question asks if Jordan's Lemma helps. The respondent says that it probably does but gives no justification. )

I have not found a good (ideally simple) way of justifying why (7) should be true.

Can any of you find a good proof of (7)?

Alternatively, can you demonstrate that P&S is in fact wrong, and that actually they should be arguing that $D_0(r)$ goes like $1/r^2$ not like $e^{-mr}$ as $r\rightarrow\infty$?

Answer 1

As you and knzhou mentioned above, Peskin’s procedure does not work because this integrand does not vanish at large contour circle.

However, the important point is the final argument of Peskin is correct although their explanation is a little bit strange. This follows from what J.G. mentioned in his comment. From today, you should be familiar with the procedure to treat this type of integral because it is really well-known among the QFT community .

Here I summarize the point.

We don’t need an analytical continuation, but we can directly evaluate this integral thanks to the modified Bessel function of second class.

Let’s see how we know this relation.

Let us consider the following integral: $$\int_{-\infty}^{\infty}dk \frac{e^{ikr}}{\sqrt{k^2+m^2}},$$ Where we assume $m>0$ for simplicity.

Firstly, we rewrite a denominator of the integrand: $$\frac{1}{\sqrt{k^2+m^2}}=\frac{1}{\Gamma(1/2)}\int_0^\infty d\lambda\ \lambda^{-1/2}e^{-\lambda(k^2+m^2)},$$ and substitute it to the original integral: $$\int_{-\infty}^{\infty}dk \frac{e^{ikr}}{\sqrt{k^2+m^2}}= \frac{1}{\sqrt{\pi}}\int_{-\infty}^{\infty}dk \ {e^{ikr}} \int_0^\infty d\lambda\ \lambda^{-1/2}e^{-\lambda(k^2+m^2)} $$ $$= \frac{1}{\sqrt{\pi}}\int_0^\infty d\lambda\ \lambda^{-1/2} e^{-\lambda m^2}\int_{-\infty}^{\infty}dk\ {e^{-\lambda k^2+ikr}} $$ $$= \int_0^\infty d\lambda\ \lambda^{-1/2} e^{-\lambda m^2}\times \frac{e^{-r^2/4\lambda}}{\sqrt{\lambda}}$$ $$= \int_0^\infty d\lambda\ \lambda^{-1} e^{-\lambda m^2-\frac{r^2}{4\lambda}}$$ $$= \int_0^\infty d\lambda\ \lambda^{-1} e^{-\lambda-\frac{m^2r^2}{4\lambda}}$$

The last integral above is one of the integral representations of the modified Bessel function: $$K_\nu(z)=\frac{1}{2}\Big(\frac{z}{2}\Big)^\nu\int_0^\infty dt\ t^{-1-\nu} e^{-t-\frac{z^2}{4t}}\ \ \ \ (|\mathrm{arg}(z)|<\pi/4)$$ Thus, we conclude that $$\int_{-\infty}^{\infty}dk \frac{e^{ikr}}{\sqrt{k^2+m^2}}=2K_0(mr).$$

An asymptotic form of $K_\nu(z)$ on the complex plane is also well known: $$K_\nu(z)\sim\sqrt\frac{\pi}{2}\frac{1}{\sqrt z}e^{-z}\ \ \ (|z|\gg 1,\ |\mathrm{arg}(z)|<{3\pi}{/2}),$$ then we will obtain $$\int_{-\infty}^{\infty}dk \frac{e^{ikr}}{\sqrt{k^2+m^2}} \sim \frac{e^{-mr}}{\sqrt{mr}}.$$

Your integral is the r-derivative of the integral above, and r-derivative does not change the exponential behavior, so Peskin’s argument is correct.

I give one comment here. Let us consider the following integral: $$\int_{-\infty}^{\infty}\frac{d^D k}{(2\pi)^D} \frac{e^{ik\cdot r}}{{k^2+m^2}}, $$ where $k$ and $r$ are a D-dimensional vector and metric is Euclidean’s one. This integral can be treated all the same way to the integral above, and the result is $$\int\frac{d^D k}{(2\pi)^D}\frac{e^{ik\cdot r}}{{k^2+m^2}}=\frac{1}{(2\pi)^{D/2}} \Big(\frac{m}{r}\Big)^{\frac{D-2}{2}}K_{\frac{D-2}{2}}(mr)\sim e^{-mr}.$$ This integral is an integral form of free propagator of the Euclidean scalar theory. This is why this type of evaluation is familiar among QFT community.