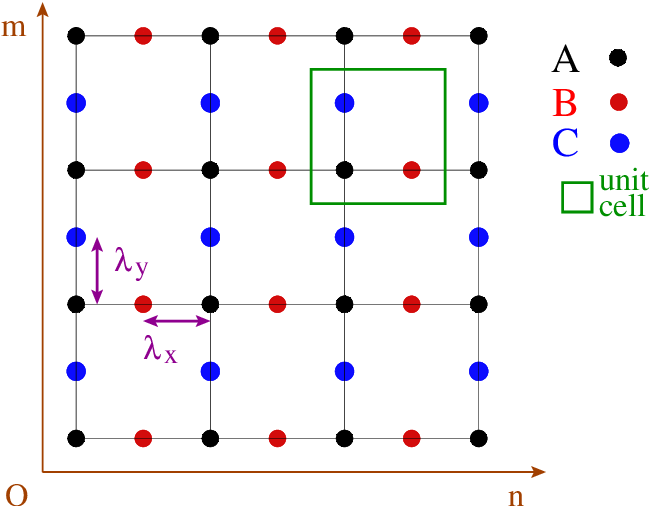
I know that a crystal structure is formed by the addition of a motif to a lattice (crystal structure = lattice + motif). I also know that an arbitrary lattice will in general exhibit certain symmetries (It must by definition have translation symmetry but it may also have rotational symmetries, reflections etc as well). An arbitrary motif on the other hand may also exhibit certain symmetries (i.e reflection, rotation etc) however it may only exhibit point symmetries and not translational symmetries. Additionally, the crystal structure itself will then exhibit certain symmetry (translational is required but it may exhibit further point symmetries as well).
What I don't understand is why the symmetries of these two entities (a lattice and a basis) have to be compatible with one another in order to form a valid crystal structure. In the first link provided below, it is stated that "If a motif has certain symmetry, the lattice must have at least that much symmetry." This statement is labelled as an all-important second law of crystallography. Yet it is left entirely unjustified. To illustrate this law, the link shows as an example a square lattice (with its characteristic 4 fold rotational symmetry) coupled with a motif that has 3-fold rotational symmetry. The combination of these two is shown below
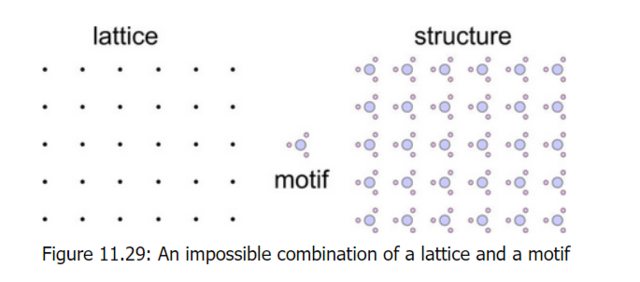
The link states that this is an "impossible combination of a lattice and a motif". But what makes this combination impossible? It is clear that the combination of these two results in a structure that exhibits the same translational symmetry as its underlying lattice. The structure is periodic and even has a horizontal mirror line as well. So why is it deemed an invalid or impossible crystal structure ? It is also clear that the lattice has 4-fold rotational symmetry while the motif has 3-fold rotational yet the resulting structure has neither 4-fold nor 3-fold rotational symmetry. The combination of these two evidently destroys some of the symmetry. But so what? Why is nature not okay with the resulting structure?
I can extend my issue further by instead populating a square lattice with a motif consisting of a circle. In this case, the motif has an infinite-fold continuous rotational symmetry while the square-lattice certain does not. So according to the crystallographic law in question this is an invalid combination. If this is so, why can we have simple cubic crystals like $\alpha$ polonium? In this case, the motif is a polonium atom which is presumably spherically symmetric. Hence it should be incompatible with a cubic lattice according to the law in question. But we know that this is not the case. Clearly, something is amiss here. So why is it requirement that the lattice of a crystal have at least as much symmetry as its motif?
EDIT I think most of my issue can be resolved if the following can be answered:
what is the plane group of the structure in fig 11.29 (if it has a plane group)
If it doesn't have a plane group, why does it's symmetry group (its mirror plus square translational symmetry) not count as one of the 17 crystallographic plane groups
Does nature ever allow for the existance of crystals that do not have the symmetry of one of the 230 space groups (3d crystals) or 17 plane groups? If so, what is the use of the 230 space groups/17 plane groups?