Does work done by a non-conservative force involve distance rather than displacement?

Question

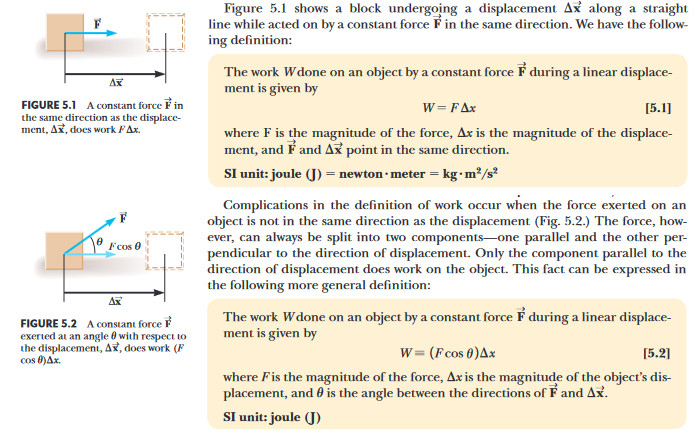
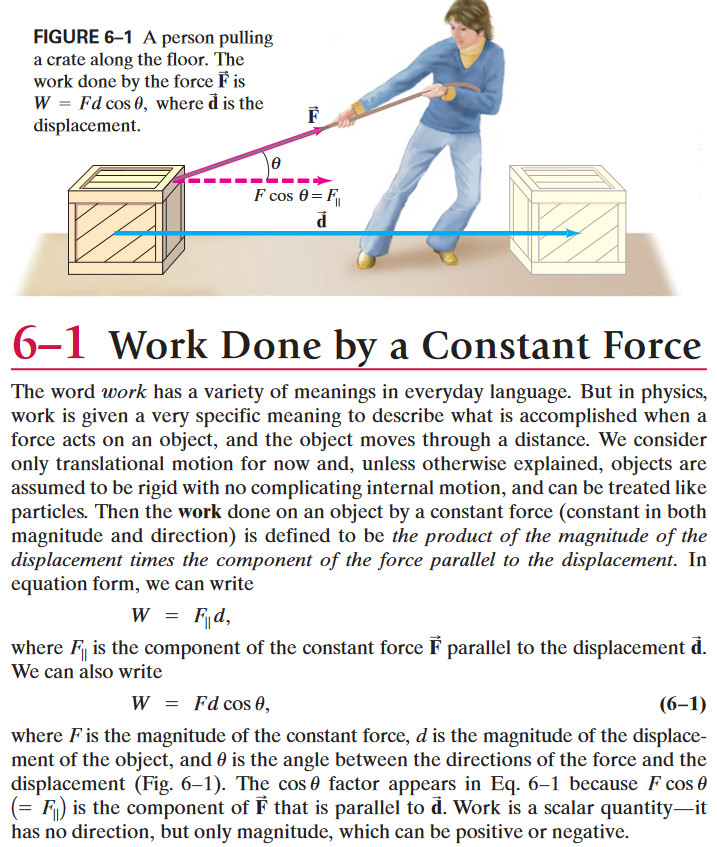
I am a new physics teacher and struggling to piece out the nuance of work calculations for my Advanced Placement (AP) students.

I feel like after a fruitful year of distinguishing between vector and scalar quantities for the use of kinematics and Newton's laws, all distinctions have been blurred in the work and energy unit. In the textbook and all resources I've found, work done by a force (no distinction between non-conservative and conservative) is found by the dot product of the force and displacement, but displacement is represented by $d$ (distance) rather than $\Delta x$. Then, we get to the topic of conservative and non-conservative forces, and it becomes clear that only conservative forces are path independent (so when displacement is zero, work done by the force is zero). So it seems that work done by non-conservative forces might be the dot product of force and distance.

Honestly, I hate this unit, as it feels like there is a lot of hand-waving and ambiguity in the way the topics are presented... and I want to make it clearer for my students but unfortunately am struggling myself to define the terms and assumptions with precision.

Answer 1

Both conservative and nonconservative forces do work as the path integral $\int _L \vec F \cdot d\vec s$.

If force and path are antiparallel (as for friction*) and force is constant in magnitude along the path, since the dot product of antiparallel vectors is negative the product of their magnitudes, we can replace the dot product with the magnitude product and pull -F out of the integral, leaving $-F \int_L ds =-FL$

If the force vector is constant in magnitude and direction along the path (as in gravity*), we can pull the whole vector out of the integral and simplify to obtain the high school version

$\vec F \cdot \int_L d\vec s = \vec F \cdot \vec s$

---

*...as typically framed in high-school appropriate problems.

Answer 2

Suppose a point particle with mass $m$ moves along the trajectory $\vec{x}(t)$ with velocity $\vec{v}(t) = d \vec{x}(t) / dt$ under the influence of some (in general nonconservative) force $\vec{F}(\vec{x}, \vec{v})$.

The time derivative of the kinetic energy $T(t)=m \, \vec{v}(t)\cdot \vec{v}(t)/2$ of the particle is given by $$\frac{dT(t)}{dt}=\frac{d}{dt} \frac{m \, \vec{v}(t)\! \cdot\! \vec{v}(t)}{2} = m \, \vec{v}(t) \! \cdot \! \frac{d \vec{v}(t)}{dt} =\vec{v}(t) \cdot \vec{F}(\vec{x}(t),\vec{v}(t)), \qquad (1)$$ where Newton's equation of motion $$ m \frac{d \vec{v}(t)}{dt}= \vec{F}(\vec{x}(t), \vec{v}(t)) \qquad (2)$$ was used in the last step. The difference $T(t_2)\! - \!T(t_1)$ of the kinetic energies at two instants of time $t_{1,2}$ is obtained by integrating eq. $(1)$ between $t_1$ and $t_2$, $$T(t_2)\!-\!T(t_1) \! = \!\int\limits_{t_1}^{t_2} \! dt \, \vec{v}(t) \! \cdot \! \vec{F}(\vec{x}(t),\vec{v}(t)) = \int\limits_{t_1}^{t_2}\! d \vec{x}(t) \cdot \vec{F}(\vec{x}(t), \vec{v}(t)), \quad (3) $$ where the resulting integral $$ W= \int\limits_{t_1}^{t_2} \! d\vec{x}(t) \cdot \vec{F}(\vec{x}(t),\vec{v}(t)) \qquad (4)$$ serves as the definition of the work done by the force acting on the particle during its motion in the time interval $[t_1,t_2]$. According to eq. $(3)$, $W$ gives the gain (for $W >0$) or loss ($W <0$) of kinetic energy of the particle. Note that the actual path taken by the particle during its motion has to be inserted in the integral given in eq. $(4)$! For an infinitesimal time interval $dt$ with the infinitesimal displacement $d \vec{x} = \vec{v} \, dt$, the corresponding work $dW$ is just given by the scalar product (dot product) $dW= d \vec{x} \cdot \vec{F}$.

For the special case of a conservative force, where $\vec{F}$ can be written in the form $\vec{F}(\vec{x}) = - \vec{\nabla} U(\vec{x})$, the determination of the work defined by eq. $(4)$ facilitates considerably. The integral is now independent of the actual path taken by the particle between its initial position $\vec{x}_1= \vec{x}(t_1)$ and its endpoint $\vec{x}_2 =\vec{x}(t_2)$, yielding $$ W= - \int\limits_{\vec{x}_1}^{\vec{x}_2} \! d \vec{x} \cdot \vec{\nabla} U(\vec{x}) = U(\vec{x}_1)-U(\vec{x}_2). \quad (5)$$ Inserting $(5)$ into $(3)$ implies energy conservation $T(t_2) + U(\vec{x}_2) = T(t_1)+U(\vec{x}_1)$ in the case of a conservative force.

Answer 3

Lot of thorough answers already, but I feel it could be stated even more clearly:

The following is always true for a differential element of work $dW$:

$$dW = \vec F \cdot d \vec s $$

To find the total work done, e.g. along a curve $C$, you integrate along all the $d \vec s $'s that make up that curve:

$$\int_C dW = \int_C \vec F \cdot d \vec s $$

This then depends on the details of how $\vec F$ varies in magnitude and direction along that curve.

This is a general principle in physics, that when you have a relationship that changes across space and time, you take the differential in space or time, where the relationship is simple, and then integrate as necessary. In fact it's why calculus was invented, to solve physics problems like this.

For a frictional force that is always negative and parallel to your path, you end up computing the arc length of your path times the force. For a conservative force that varies as $\vec F(x,y,z)$ or $\vec F(r,\theta,\phi)$, you could express the force and $d\vec s$ in terms of functions of the spatial coordinates and do the algebra from there.

Answer 4

One of the difficulties may be the overuse of one dimensional examples (to simplify things), where the difference of vectors and scalar quantities can be easily blurred.

If we use 3D examples, and the vectorial representation of force $\mathbf F = (F_x, F_y, F_z)$, and the same for infinitesimal displacement: $\mathbf {dr} = (dx, dy, dz)$, the infinitesimal work as dot product (and so a scalar) becomes clear: $dw = \mathbf F.\mathbf {dr} = F_xdx + F_ydy + F_zdz$.

The definition is the same for conservative or non conservative forces.

Answer 5

Looking through the AP Physics 1 Course and Examination description and a number of AP Physics textbooks the emphasis is on a constant force analysis possibly with a variable force introduced as an extension.

Although work done (scalar) is defined as the dot product of a force (vector) and a displacement (vector) it should not be introduced in this way at High School level.

Here is how work is a summary of how work done is introduced in College Physics by Selway et al.

Note that in reference to Figure 5.1 the force $\bf \vec F$ and displacement $\Delta \bf x$ are shown to be vector quantities in the same direction and motion is along a straight line whereas in the equation $W=F\,\Delta x$, $F$ and $\Delta x$ are magnitudes of the vectors.
When the force and the displacement are not in the same direction the equation for work done is given as $W=(F\,\cos \theta)\,\Delta x$ and again $F$ and $\Delta x$ are magnitudes.
It is the $\cos \theta$ term which assigns a sign to the work done but it is important to point out that the sign is nothing to do with direction.
$\Delta x$, the magnitude of the displacement, is called the distance.

The same ideas are also in Physics - Principles and Applications by Giancoli.

In this text a displacement is shown as $\bf \vec d$ and distance as $d$.
Again it is the $\cos \theta$ term which dictates the sign of the work done.

---

As to conservative and non-conservative forces, the definition of work done is identical for both.
The difference is that for a conservative force the work done is path independent whereas for non-conservative forces the work done will usually be path dependent.