Maxwell's Demon as a heat source on memory erasure

Question

The paradox of Maxwell's Demon, consisting of a box a gas, separated in two compartments, with a door, are initially both sides in thermal equilibrium hence at maxim entropy. By the action of an imaginary being, a Demon, who is able to observe the directions and speeds of each individual particle in each compartment at each moment, is able to open the door between the two side in the right moment, resulting in more faster particles gathered on one side, and more slower particles gathered on the other side, in this way a temperature difference produced between the two sides, no longer in thermal equilibrium, meaning the entropy of the system decreased (also the Universe's total entropy). As the Demon having a finite amount memory, after a while it is forced to erase the information accumulated during his action, but according to Lanadauer's principle, which states that, the erasure of information is an irreversible, physically heat dissipating process. This released heat increases the environment's (also the total entropy of the Universe) entropy, balancing out the entropy decrease created by the Demon, thus the Second law is claimed to be preserved, and the paradox resolved. The problem is that, in this way, the Demon (his memory) on erasure becomes a heat source itself, possibly available to do useful work with it, in this way invalidating the resolution to the paradox. So, the question is, how the total entropy still increases, and how the Second law is preserved, if the Demon's memory -on erasure- is a heat source itself, good to do useful work with it? Is this long enough now? I expect answers then.

Answer 1

possibly available to do useful work with it, in this way invalidating the resolution to the paradox

The issue with the initial premise lies here (as I noted on your previous question that you deleted and reposted—please don't do that, as it discards discussion that some might find useful).

The Second Law prohibits total entropy from being destroyed.

Suppose the Demon is used as the hot reservoir in a heat engine. Then we have two phenomena occurring:

1. The Demon reduces local entropy in the box, but this is argued to be acceptable because entropy is generated elsewhere from erasing information.

2. The Demon loses entropy from being used as a hot reservoir, but this is acceptable because the entropy of an associated cold reservoir is increased by at least the same amount, as with any heat engine. (This is cleverly done in the usual manner by removing entropy $\frac{q}{T_\mathrm{hot}}$ from the hot reservoir and depositing at least as much entropy as $\frac{q-w}{T_\mathrm{cold}}$ in the cold reservoir, allowing work output $w$.)

The two phenomena can be uncoupled. If there is no Second Law problem with either individually, there is no problem with them used together in series. Therefore, it's not clear why any explanation would be invalidated.

Answer 2

You mention "[Landauer]'s principle, which states that, the erasure of information is an irreversible, physically heat dissipating process", and jump to the conclusion that erasure is therefore a source of heat. This isn't what Landauer's principle is saying. It means that the demon has to find an external source of heat and dissipate it in the environment in order to perform the erasure.

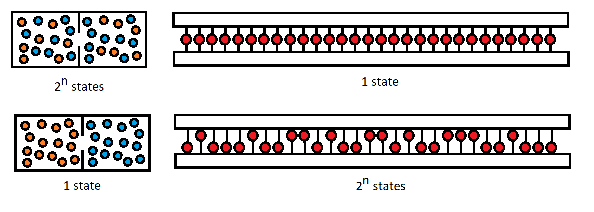
However, it's not a good explanation of the Maxwell's demon argument. For this, consider the classic case of a demon separating hot and cold molecules, using an abacus for data storage.

The demon measures the temperature of each gas molecule, and reduces the $2^n$ states of the well-mixed gas to $1$ state. At the same time, he transforms the $1$ state of an empty memory store to the $2^n$ possible states of a full one.

Now we can see that the demon is faced with exactly the same problem when it comes to erasing the memory store. The beads of the abacus are divided into two groups representing 'hot' and 'cold'. In order to erase the data, the demon has to measure the state of each one so it knows how to perform the transformation to the 'empty' state. All we have done is moved the problem, not solved it.

This is where Landauer's principle comes from - erasure of a data store is exactly the same sort of problem as separating a mix of hot and cold molecules, and thus requires an external source of entropy increase to perform the erasure, such as heat dissipation. Thus, Landauer's principle assumes the demon's impossibility, and assumes the standard thermodynamic formula for heat engines to calculate an amount of energy to dissipate.

Answer 3

Thanks for your answer @Chemomecanics & Nullius In Verba. Second, sorry for my english, my phrasing can be imperfect. I think it is my mistake I forgot to mention one very important, but officially accepted fact: only memory erasure requires energy investment, memory write doesn't!

Correct me if I understand wrongly the Second Law of Thermodynamics, this is how I understand it:

"It is impossible to create an engine which working in cycle, results in no other than the extraction of heat energy without at least the same amount of external energy invested first."

The Demon does exactly this: without any energy investment is able to extract heat energy, because memory write has no cost. This is the paradox in short. The solution is, that yes yes, the Demon can extract without any energy investment, but when he needs to erase his memory, he needs to use at least the same amount of energy as he extracted, so he needs to use the energy he extracted to do the erasure. In short, in this way the problem is solved, no net energy is gained for no investment. However let's notice a cyclic energy flow is created either way, without any energy investment, and what prevents me to insert a heat engine to extract some energy, and do dome external useful work with it? Because when the Demon does the memory erasure, uses the energy extracted, so the energy flows through his memory back to the box-system. The Demon's memory becomes a heat source (the hot body) and the box-system becomes the cold body, actually becoming a possible energy-extraction point. Do you observe that the energy-neccessity-to-erase of the Demon is actually redundant?

Here are some presentations about the cycle:

      Box
      / ^
     /   \
    /     \
   /       \ <--- Possible energy extraction point
  /         \
 /           \
V             \

Ext.energy-------->Demon storage

Since here I suppose to write an answer, then my answer would be that Landauer's principle is wrong, memory write and erasure has the same cost, a Demon would be able to do this action. The classical thermodynamic Second Law was formulated, at a time when the atomic composition of the matter was still under heavy debate, many even rejected, and gases (and heat) was considered as "indivisible bodies", to which the original formulation of the Second Law what I wrote above was true. Science moved on, atoms were proven, thermodynamics haven't: physicists still today cling convulsively to the impossibility of extraction of the internal energy of the gases at no cost, because that would be something "too good to be true perpetuum mobile". To cut short, -here I can't explain in short-, to this adds the deep confusion between physical and informational entropy. The reason I opened this question is, if anyone could find a reason, what I haven't thought of, why the Demon's memory on erasure cannot be used as a heat source for energy extraction. I think it was never intended to ... by definition the heat generated on erasure is "waste heat" which supposed to be considered as lost in the environment (to increase back the global entropy), but one can argue why not to use it, since it can be considered coming from a source, the Demon's memory.