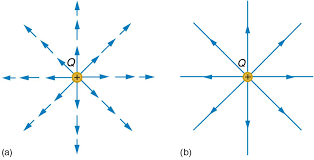
I don't understand how streamlines are related to electric fields. I thought they are related to the lines of fluids.
I searched and saw that streamlines represent curves of velocity vectors, whereas electric fields lines represent curves of electric field vectors.
So I'm confused, what's the difference?
But in our electrostatics course, they asked us to get the streamline equations of $\vec{E}$ (electric field). Do they mean to get the equations of the electric field lines a.k.a. "streamlines"?
Because the way I reasoned this, is the definition of streamlines, is the field lines that are tangent to the velocity vector of the fluid, and here in electrostatics, the electric field vectors are tangent to the electric field lines, so that must mean that electric field lines — streamlines right?