Confusing case of completely inelastic collision

Question

A piece of clay falls from height $h$ on the inclined surface of an inclined plane. After it hits the plane, it sticks to it and slides down the incline. Is this kind of collision completely inelastic? Let's say the plane rests on a horizontal frictionless floor. It should receive an impulse directed perpendicular to the inclined surface. This should move it horizontally on the floor. But the piece of clay slides down in the opposite direction. Are they considered to be sticking together and moving at the same speed after the collision? That certainly doesn't seem to be the case to me. If not, how can I tackle this problem?

Answer 1

It should receive an impulse directed perpendicular to the inclined surface.

Depending on how you define the situation this is not true. The fact that there are 3 bodies (the clay, the wedge and the floor) makes this problem harder so first consider the clay and the wedge in a vacuum in space. The clay will now hit at a point along a line that goes through both centers of mass to prevent any rotation. For a collision there always a normal force and sometimes a tangential force. If there was only a normal force then the wedge would receive an impulse in the direction normal to the surface of the wedge. If we add back the floor then the wedge would start moving.

However, you specified that the clay would completely stick to the surface after collision. This means that the impulse is parallel to the initial velocity of the clay. Sticky surfaces allow for tangential forces.

Now we will add back the floor and allow the clay to slide. The wedge will start to slide in the opposite direction to the clay. We specified the clay now slides (even though it didn't during the collision) so the tangential force is now no longer sufficient to stop the clay. This means the normal + tangential force is no longer pointing vertically down and there is a net force on the wedge in the horizontal direction.

Is this collision completely inelastic? The initial collision, after which it stuck to wedge, is completely inelastic. The maximal amount of kinetic energy is lost, which is what defines an inelastic collision. After that we would be hard pressed to call the situation a collision. Gravity is continuously acting on the clay and the three bodies are continuously touching each other.

Answer 2

It is a common misconception that sticking is the same as inelastic collosion. Sticking $ ≠ $ inelastic collision. Inelastic collisions are ones in which the coefficient of restitution, $ e = 0$, meaning $ \vec v_{2} - \vec v_{1} =0 $ where $ \vec v_{1}$ and $ \vec v_{2} $ are initial and final velocities along the common normal. Velocities of the colliding objects come out to be the same, which equates to sticking in one dimension. but in multi dimensional collisions, this "sticking" idea falls apart. Consider two balls, moving in different directions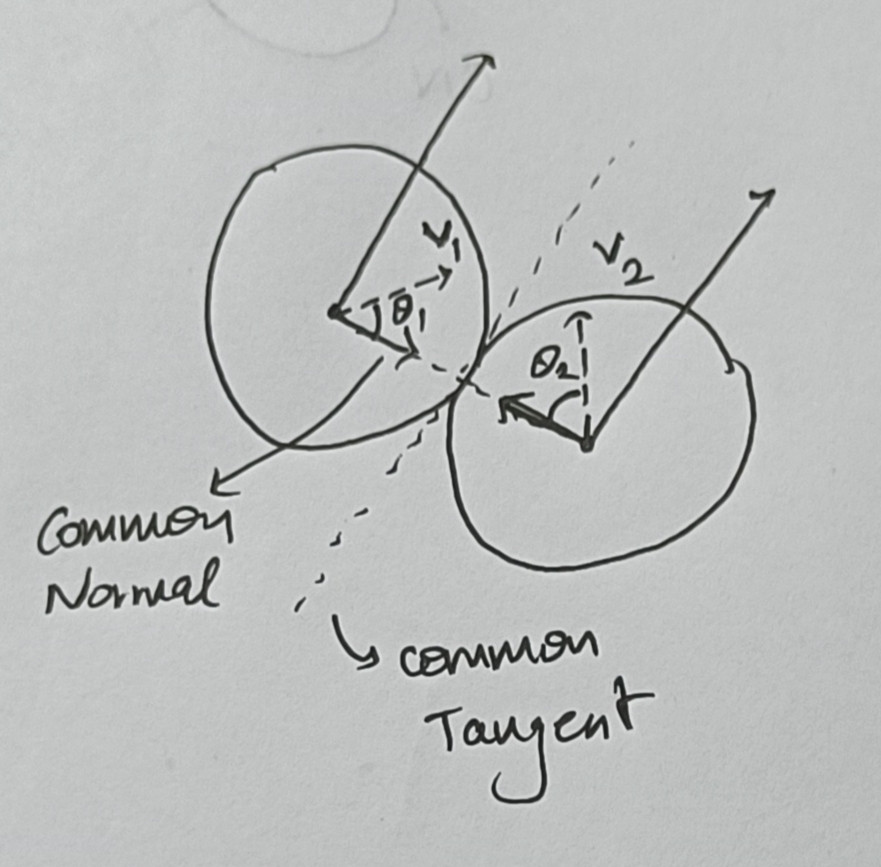

Collision only occurs along the common normal, and hence, the velocities we plug into the equation $ e= \frac{\vec v_{2} - \vec v_{1}}{\vec u_{1} - \vec u_{2}} $ are the components of the respective velocities, along the common normal. Notice how even after the collision, the velocities of each ball along the common tangent stays exactly the same as what it was before collision? This is a case of inelastic collision with no sticking, if $ e=0$

If in your question it is mentioned that the collision is inelastic, then just apply $ e=0$ along the common normal and proceed. But if it is the case that the particle is sticking to the wedge, then you need to use impulse momentum equations, and apply the condition that the final velocity component of the ball along horizontal is equal to that of the wedge, use these to calculate whatever is required.