1
$\begingroup$

The tide on Earth appears absolutely whenever the moon is overhead. Is that tide caused by spacetime, re-curvature in space or attraction gravity?

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ Related: physics.stackexchange.com/q/3009/2451 $\endgroup$
    – Qmechanic ♦
    Commented Aug 17, 2013 at 12:06
  • $\begingroup$ BTW--The tide also appears when the moon is underfoot (as it were). This is obvious when you understand the physics and may help you in establishing that understanding. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Aug 17, 2013 at 21:32

4 Answers 4

Reset to default
7
$\begingroup$

Well, basically gravity is a consequence of curved spacetime!

Space-time is distorted by the presence of mass. This results in any other mass in the vicinity being attracted to it. This attractive force is called gravity. So, basically gravity is caused because masses curve space-time.

Improve this answer
$\endgroup$
3
  • 2
    $\begingroup$ Actually the rubber sheet or mattress analogy, though very common, is terrible! See physics.stackexchange.com/q/7781, physics.stackexchange.com/q/16925 and physics.stackexchange.com/q/3009 $\endgroup$
    – Michael
    Commented Aug 17, 2013 at 14:09
  • 1
    $\begingroup$ Well it may not be true, but it works something like that - where there's mass, other mass will be attracted. For a layman to understand I think it's quite a good way to show it. $\endgroup$
    – mikhailcazi
    Commented Aug 17, 2013 at 14:27
  • 2
    $\begingroup$ Anyway, since it is NOT true, I'll remove that part. $\endgroup$
    – mikhailcazi
    Commented Aug 17, 2013 at 14:31
1
$\begingroup$

First,tides can also occur when the moon is directly below us.Tides occur at an interval of 12 hrs so twice a day.

Now,we don't need curvature of space to explain tides on earth.They can simply be explained by Newtonian gravity.

Consider the situation as shown in the figure.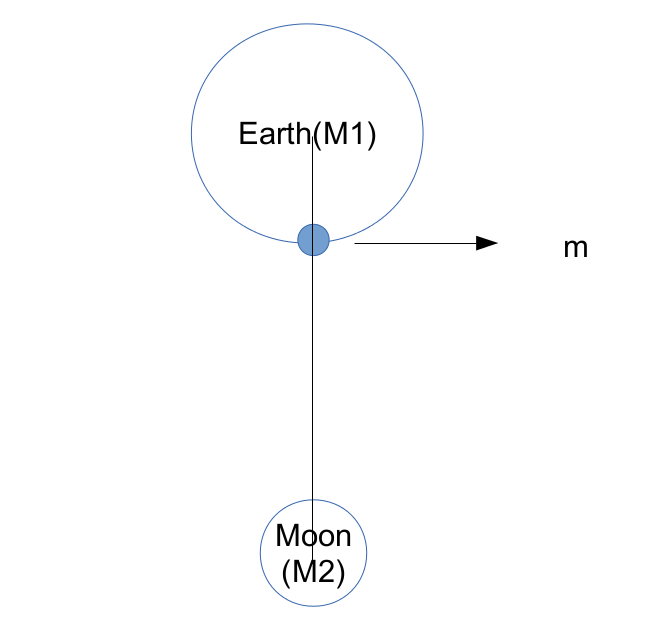enter image description here

m stands for some small mass relative to $M_1$ and $M_2$ which represents say the oceans.

Lets calculate the difference between force of moon acting at centre and acting at edge on $m$(This gives us the tidal force)

$F_m=(\frac{GM_2m}{(d-R_1)^2} - \frac{GM_2m}{d^2})\hat{r}$

where

$d=$distance between earth and moon,

$\hat{r}$ is the radius vector from the earth towards the moon.

Now expanding the first term as a Taylor series and neglecting terms of order three and higher($d>>R_1$):

$F_m=(\frac{GM_2m}{d^2}+\frac{GM_2mR_1}{d^3} - \frac{GM_2m}{d^2})\hat{r}$ $=\frac{GM_2mR_1}{d^3}\hat{r}$ Now this force points towards the moon(from our definition of $\hat{r}$) and therefore the water slightly moves towards the moon.

If we were to repeat our calculation for the mass $m$ on the other side of earth,we would observe this "tidal force" to point away from the moon which makes $m$ go away from the earth.This leads to the familiar shape of earth's oceans.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Indeed this demonstrates the basic mechanism. Then put the system in motion (earth's rotation and moon's orbit) and it no longer lines up properly: en.wikipedia.org/wiki/Lunitidal_interval. Then add solar tidal force. $\endgroup$
    – Steve Jessop
    Commented Mar 11, 2014 at 11:42
0
$\begingroup$

"we don't need curvature of space to explain tides on earth. They can simply be explained by Newtonian gravity"

Isn't that contradictory? first, Apparently, Einstein actually disproved Newton's theory of gravity as a force. If Newton's gravity force theory was wrong, and even if it can be used to explain tides, that doesn't mean it is what is happening in reality. Ptolemy's solar system, with the Earth at its center, did a very good job explaining the apparent movements of the planets; and although it was mathematically coherent, we now know it was absolutely wrong. In Addition, how can the curvature of space-time produced by the presence of the moon, stabilize the Earth axis? Whatsmore, "For many animals, particularly birds, the moon is essential to migration and navigation; others time their reproduction to coincide with the specific phases of the lunar cycle" ("Three ways the Moon Affects Wild Live" https://www.discoverwildlife.com/animal-facts/ways-moon-affects-wildlife/. I have tried to find answers to those puzzles!

Improve this answer
$\endgroup$
-1
$\begingroup$

The oceans , seas , all objects in the earth are already masses added to the earth and objects attracted to it because the total mass earth had already distorted the space time curvature and any object in the vicinity will follow geodesic lines to the center of that mass, which in turn will be thought of gravity forces. This is according to relativity theory but another explanation if we cosider Newtonian mechanics in which case it is the force of gravity attraction between the earth and other objects that cause the objects tied to the earth. This force is $$F=Gm.M/r^2$$, where $F$ is the attraction force , $M$ is the big mass, $m$ is the small mass , $r$ is the distance between the two masses. It is clear that if $r$ approaches zero then the force of attraction can approach infinity and that there is no way for oceans or seas or objects to escape the earth

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.