My question is from chapter #18 "The impulse-energy law for matter as a consequence of the field equations", from the derivation of equation above (57). He says that we can get (57) by multiplying the partial derivative $∂g^{μν}/∂x_σ$ with equation (53), I tried but didn't get it.
I know why $g^{μν}\frac{∂g^{μν}}{∂x_σ}$ vanish, it's just because we can choose coordinates that $\sqrt{-g}=-1$
Note: This problem isn't the same as physics.stackexchange.com/q/742803/2451
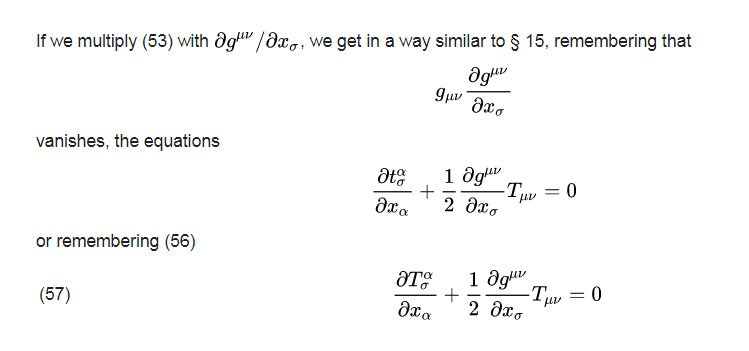
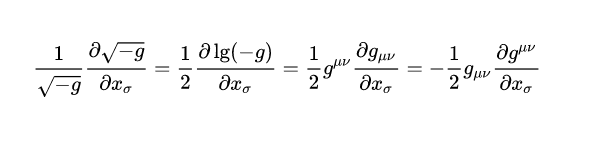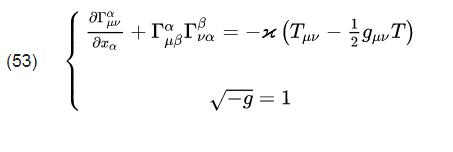Reading the whole chapter, you can get two useful equations. The first one is the conservation of energy: $$\frac{\partial(t^{\alpha}_{\sigma}+T^{\alpha}_{\sigma})}{\partial x^{\alpha}} = 0\tag{1}$$
And the other one is an equation that you get after multiplying the LHS of (53) with the metric derivative i.e. $$ \frac{\partial g^{\mu\nu}}{\partial x^\sigma}(\frac{\partial\Gamma^{\alpha}_{\mu\nu}}{\partial x^{\alpha}}+\Gamma^{\alpha}_{\mu\beta}\Gamma^{\beta}_{\nu\alpha}) = -2\chi\frac{\partial t^{\alpha}_{\sigma}}{\partial x^{\alpha}}\tag{2}$$
I'll edit my answer if I found an easy way to derive it. But with this, you can easily get equation (57). Indeed, using the vanishing equation: $$ -2\chi\frac{\partial t^{\alpha}_{\sigma}}{\partial x^{\alpha}} = -\chi(\frac{\partial g^{\mu\nu}}{\partial x^\sigma}T_{\mu\nu}-\frac{1}{2}\frac{\partial g^{\mu\nu}}{\partial x^\sigma}g_{\mu\nu}T) =-\chi\frac{\partial g^{\mu\nu}}{\partial x^\sigma}T_{\mu\nu}$$
Since $\frac{\partial t^{\alpha}_{\sigma}}{\partial x^{\sigma}}=-\frac{\partial T^{\alpha}_{\alpha}}{\partial x^{\alpha}}$, we recover equation (57).
Edit : I found how to get (2) from the previous equations, but you might want to go on the detail on your own (not trivial). For other people reading this answer, the link to paper's section is here.
Using (49), you have the following equation : $$ -2\chi t^{\alpha}_{\sigma} = \frac{\partial g^{\mu\nu}}{\partial x^\sigma} \frac{\partial H}{g^{\mu\nu}_{\sigma}}-\delta^{\alpha}_{\sigma}H\tag{3}$$
You also know from (47b) that : $$ \frac{\partial\Gamma^{\alpha}_{\mu\nu}}{\partial x^{\alpha}}+\Gamma^{\alpha}_{\mu\beta}\Gamma^{\beta}_{\nu\alpha} = \frac{\partial}{\partial x^{\alpha}}(\frac{\partial H}{\partial g^{\mu\nu}_{\sigma}})-\frac{\partial H}{\partial g^{\mu\nu}}$$
If you multiply the RHS by the metric derivative, you get (it is explained a bit in the paper) : $$ \frac{\partial}{\partial x^{\alpha}}(\frac{\partial g^{\mu\nu}}{\partial x^{\sigma}}\frac{\partial H}{\partial g^{\mu\nu}_{\alpha}})-\frac{\partial H}{\partial x^{\sigma}}\tag{4}$$
Where $\frac{\partial H}{\partial x^{\sigma}} = \frac{\partial H}{\partial g^{\mu\nu}_{\alpha}}\frac{\partial g^{\mu\nu}_{\alpha}}{\partial x^{\sigma}}+\frac{\partial g^{\mu\nu}}{\partial x^{\sigma}}\frac{\partial H}{\partial g^{\mu\nu}}$. Thus, if you multiply the RHS of (3) by $\frac{\partial}{\partial x^{\alpha}}$, you obtain (4), getting finally equation (2).
