Intuitive and/or qualitative meaning of Heisenberg's uncertainty principle

Question

I'm trying to figure out what the uncertainty principle really means, and I've arrived to construct this 'mental experiment': let's suppose to know with great precision the momentum of an electron (or any other particle as well); with this information I can say, for example, that the particle is moving in a straight line (am I right?). However, due to the uncertainty principle, I cannot say where the particle is on this straight line.

On the other hand, if I could describe the motion of this particle with a highly localized wave, I would know for every instant where the particle is (at least the region of space where one is more likely to locate the particle). If I'm right until this point, it would be sufficent to measure the position of the particle at any time to know that it is moving on a straight line (and thus, to know its momentum)? Where am I wrong? What is the right counterpart of the first part of my reasoning?

(Blue is the wave function showing the momentum, I can't say where the particle is; red: the wave function not knowing momentum, I can say where the particle is.)

Answer 1

with this information I can say, for example, that the particle is moving in straight line (am I right?).

Particles don't have trajectories in quantum mechanics (meaning, there's no way represent a particle's motion with a function $x(t)$ that tells you where the particle is at every time). In that sense, there's no way you can know, within quantum mechanics, that a particle is moving on any specific trajectory, such as a line.

With a good measurement of momentum, the particle could be located anywhere in space. All you would know is the direction of its motion and magnitude of its momentum. However, for a "free particle" (meaning with no external momentum applied), the momentum measurement would be repeatable -- so long as you don't disturb the system, measurements of the momentum will continue to give a consistent answer after you make the first one.

If I'm right until this point, would be sufficent to measure at any time the position of the particle to know that is moving on a straight line (and thus, to know its momentum)? Where I'm wrong?

Let's say you first measure the momentum, so you know the particle's momentum fairly well. Then you perform a measurement of position. This measurement of position will necessarily disturb the particle, so that the information you originally had about the particle's momentum is lost. This is the essence of the uncertainty principle.

In particular, you can learn that the particle is located in a small region around the lower southeast corner of your detector (say). However, after you make this measurement, there will be a huge uncertainty in the momentum of the particle. The particle can be traveling in any direction with any speed.

You can see this in different ways.

1. If you measure the position, then measure the momentum again, in an ensemble of identically prepared systems, you will get a random distribution of momentum measurements. You can predict the distribution, but not the outcome of any one measurement.

2. If you measure the position, wait a "long" time, and measure the position again, in an ensemble of identically prepared systems, you will find that the distribution of second positions forms some kind of "spherical cloud" around the original position. The distribution of second measurement positions won't lie along a line. (If you measure the second position a very short time after the first one, you will find that the position will not have changed much -- this is the quantum zeno effect).

TL;DR: you can't "cheat" the uncertainty principle by alternating measurements of position and momentum to learn the trajectory of the particle. In fact, there is not a single trajectory you can assign to a particle in quantum mechanics.

Answer 2

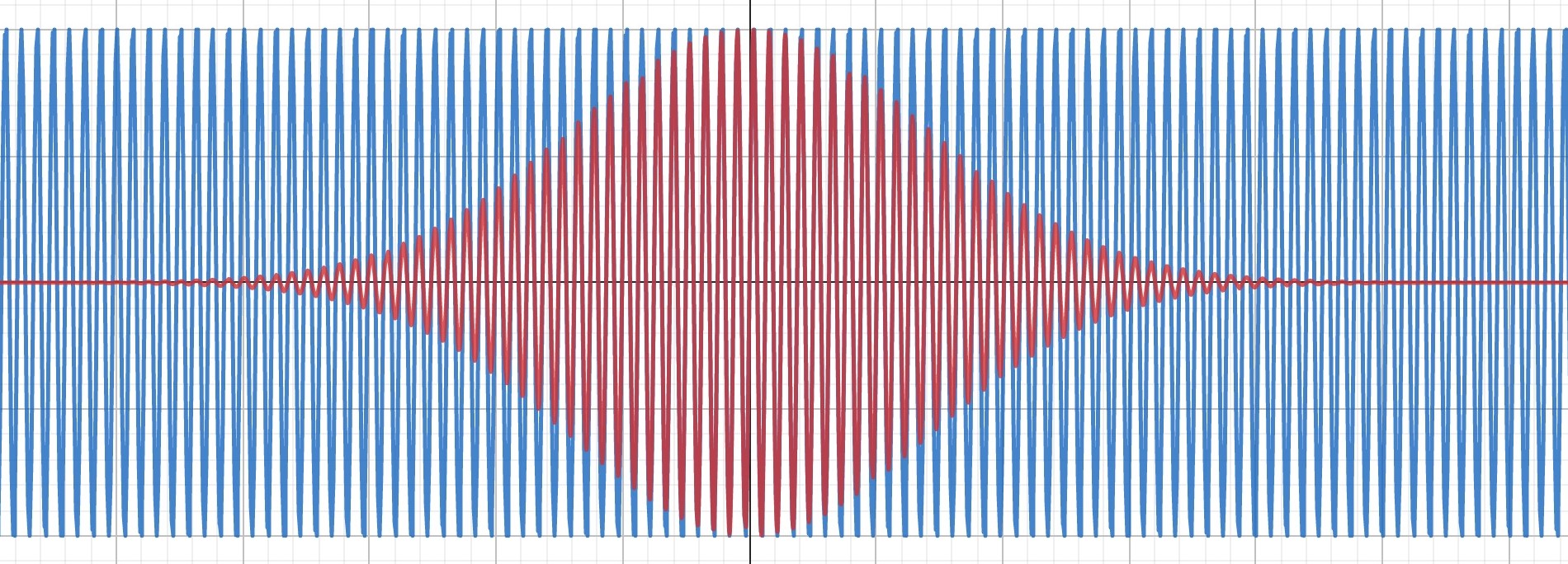
Your diagram gives the general idea about the HUP, which is that when a particle has well-defined momentum, it has a wave function that is widely spread (your blue wave), while a particle with a more localised wave function (your red wave) does not have a well-defined momentum. Where your diagram is wrong is that wave functions are 'normalised', so that they integrate over all space to the value of 1 - your blue wave therefore cannot have the same maximum amplitude as your red wave. However, that is not so important if you just want a general idea of how the HUP works.

The problem with your reasoning is that according to quantum mechanics, if you want to measure the position of a particle whose wave function is very spread-out (i.e. it has a reasonably well-defined momentum) you must subject it to some form of interaction that causes its wave function to change and become more localised. There are two important constraints here. Firstly, the localising happens at random, so the peak of the new wave function could be anywhere within the scope of the original wave function. The second is that the change in the shape of the wave function means that the particle no longer has a well defined momentum. Importantly, if you now want to measure the momentum again, you have to subject the particle to another interaction to change its wave function back to one that corresponds to a more tightly defined spread of momentum values, and when you do that there is a randomness to the effect, so that the new momentum you measure will not be the same as the earlier momentum associated with the particle before you tried to establish its position.

So the problem, therefore, is that to narrow down the momentum of a particle you must manipulate its wave function into one that is very spread out, which means that you are very unsure where the particle is. On the contrary, if you want to know where a particle is, you have to manipulate its wave function to be very localised, in which case it no longer has a well-defined momentum. And every time you cause the particle to switch between a localised or unlocalised wave function by performing an experiment on it, you introduce changes that are random in nature, so you can never really pin down the position and momentum together.

If you want a more mathematical insight...

The momentum of a particle is determined by the frequency of its wave function. The only type of wave that has a single frequency - i.e. that can be equated to a perfectly defined momentum - is a pure sine wave, which has to be spread-out everywhere (and is in effect an idealised mathematical abstraction).

The location of a particle can be anywhere where its wave function is non-zero, so in the ideal case of a particle whose wave function is a sine wave spread through all space, the particle could be anywhere.

In formal quantum mechanics, it is possible in theory to localise a particle at a point in space, in which case its wave function is a spike with an infinitesimally narrow width, known as a Dirac delta function, which does not have a frequency (so you cannot associate a momentum with it).

You can, however, express a delta function, or any roughly localised function such as your red wave, as a sum of lots of component waves, each component being a sine wave with a well-defined frequency. You can therefore think of your red wave as being a mix of sine waves, where the mix has a spread of frequencies.

What is especially beautiful and intriguing about quantum mechanics is that when you measure the momentum of a particle which is in a state represented by your red wave, i.e. one that has a mix of frequency components, you effectively force the particle's wave function to switch to become just one of those components, unpredictably, where the probability of the particle picking one component over another is related to how much each component figured in the mix of components that formed the red wave.

Answer 3

Every instance of measurement is random whether classical or quantum. By quantum means divide observable into fundamental smaller units that is multiple of constraints. Good thing is that random things are not random once we tag them with probability. So all burden goes to find probability, now see how easy is to find average. Okay, what if probability is varying or not assigned with equi-partition. Well then get the probability of one part and then sum all over.

There is no way to find momentum or energy without losing particles forever. Though momentum and energy are similar but one with direction because it has to be something with spatial distribution. To trace momentum one has to observe position multiple times. And every measurement of position is affecting future measurement of position, this is similar to be conscious in human nature.

Suppose one toss a coin its sample space has two outcomes, {h,t}. Now if one observe it during flight then its sample space has four outcomes {hh, ht, th, tt}. So probability of any outcome is thinning by observing, that is spreading of function and a spreaded function is broader or more random. If one has to observe event which has $n$ possibility, then its possibility increases by $2^n$.