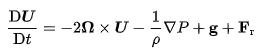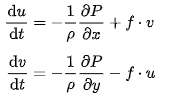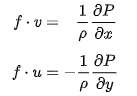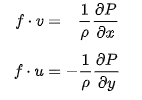How can flow along a stream line with constant pressure spatially change its velocity?

Question

I'm going crazy:

In meteorology there is a term "geostrophic wind". It is wind moving along surfaces of constant pressure.

https://en.wikipedia.org/wiki/Geostrophic_wind

How is the step from

to

carried out?

I thought all the time, that material derivative is defined by

$$\frac{DU}{Dt}= \frac{\partial U}{\partial t}+ \vec \nabla U \cdot U$$

Why do they suddenly change from D to d ?

Stationary means for me, that local speed does not change with time

$$\partial U/\partial t=0$$

so for stationary flow I would write instead

$$\vec \nabla U \cdot U = -2 \Omega \times U -\frac{1}{\rho} \ldots $$

What they do instead is to set the material derivative to Zero to end up finally with

Why? By what argument? This would mean that magnitude of speed U cannot change along the flux line of motion, because both pressure- and Coriolis force are perpendicular to U: flow is along lines of constant pressure.

But, if magnitude of U cannot change on a line of constant pressure, this is contrary to the statement that U depends on the gradient of p. So if gradient of p changes (e.g. the lines of constant pressure converge and get tighter), then the speed U must change too:

In fact this means, that U is proportional to the gradient of p, which is not constant but normally changes spatially.

So I end up with a dicrepancy: How can an air parcel which moves along a line of constant pressure ever get faster or slower, when all forces are only perpendicular to its velocity? Pressure force is perpendicular because we move on a p=const line and Coriolis force is perpendicular to U too. So from which force should the air particle get energy to get faster or slower?

When I remember Bernoulli's Law, the sum

$$\rho v²/2+p = const.$$

is constant on a stream line (set gravity to zero). So alone from this it cannot be, that speed is gained on a line with constant pressure: The more v I have, the less p I get.

Unfortunately I can't solve this puzzle. Although I'm trying my best, I can't understand even the answers I've received elsewhere and I'm not sure if my question was understood correctly.

Answer 1

Your wiki reference mentions which assumptions are made to derive the equations:

Neglecting friction and vertical motion, as justified by the Taylor–Proudman theorem

The Taylor-Proudman theorem states (according to the wiki):

If we assume that ${\displaystyle F=\nabla \Phi =-2\rho \mathbf {\Omega } \times {\mathbf {u} }}$ is a scalar potential and the advective term on the left may be neglected (reasonable if the Rossby number is much less than unity) and that the flow is incompressible (density is constant)

In that case your material derivative ($D$) simplifies to a normal derivative ($d$)