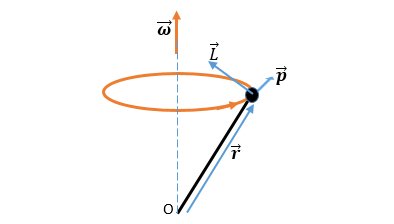
For this object to rotate there has to be a force $F_x$ pointing towards the center of the circle. Therefore there has to be a torque $\vec{r} \times \vec{F}$ pointing out of the page. If torque points in the direction in which L is increasing, shouldn't the mass rotate counterclockwise? (because torque points out of the page which corresponds to counterclockwise rotation by right hand rule). I fail to understand how torque influences the angular momentum in cases where the angular momentum and angular velocity do not point in the same direction.
Or better asked, we know that $\vec{L} = {\bf I}\vec{\omega}$ where ${\bf I}$ is a tensor and $\vec{\omega}$ is a vector. Suppose a torque is applied to a system. The direction of $\vec{L}$ is going to change, but what about $\vec{\omega}$? Will $\vec{L}$ and $\vec{\omega}$ preserve the angle that is between them?