Inelastic static tetrahedron with 3 fixed vertices, and 1 free vertex with an applied force

Question

This is a problem I've posed myself, which I cannot for the life of me figure out, despite it clearly having a definite answer.

Given:

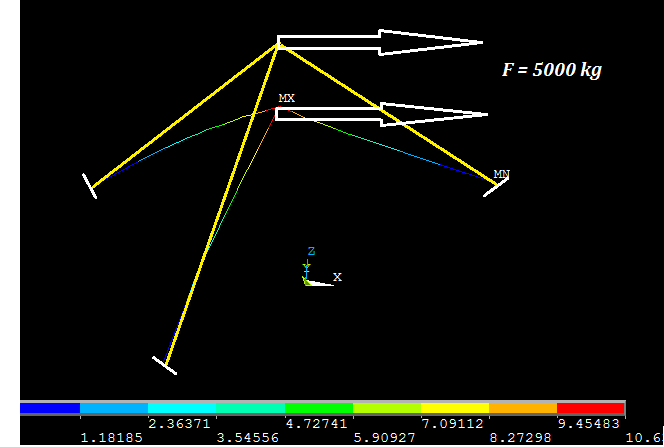
• A perfectly inelastic tetrahedron in three-dimensional space with vertex coordinates: $$A = \langle a_{1}, a_{2}, a_{3}\rangle$$ $$B = \langle b_{1}, b_{2}, b_{3}\rangle$$ $$C = \langle c_{1}, c_{2}, c_{3}\rangle$$ $$D = \langle 0,0,0\rangle$$
• A force vector $\vec{F}$ is applied to point $d$.

Assume:

• Points $A$, $B$, and $C$ are fixed.
• The tetrahedron is completely still (is in static & rotational equilibrium).

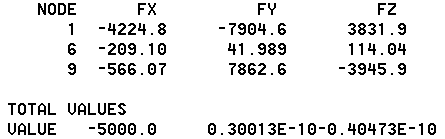
Calculate the forces on points $A$, $B$, and $C$ that are necessary in order to maintain said equilibriums.

Summary: Tetrahedron with three fixed vertices, and a force applied to fourth vertex. Find the normal forces (as vectors in three-dimensional space) that will act on the fixed vertices to cancel out this force.

I have tried setting both the net force and net torque to zero; however, this does not appear to be enough information to be able to solve the for the forces acting at $A$, $B$, and $C$ in terms of just the $A$, $B$, and $C$ position and the force vector $\vec{F}$.

Answer 1

I'm not convinced that there is a definite answer.

For simplicity, suppose the force exerted at point D is zero. Are the forces determined for the other three points?

Well, one solution is that they are all zero. With no forces, the tetrahedron will not move.

Another solution is equal/opposite forces applied to A and B, directly towards or directly away from one another. Likewise for any pair of vertices.

Then we can take any solution and simply double all the forces. If the tetrahedron is static under the initial forces, it will continue to be so when you double them.

And we can take any pair of solutions and add them. If $F_A+F_B+F_C=0$ and $f_A+f_B+f_C=0$ then $(F_A+f_A)+(F_B+f_B)+(F_C+f_C)=0$, and likewise for moments. The sum will still be a solution.

So unless there are constraints on the directions of forces you haven't mentioned, I don't think a single solution can be identified.

I am guessing you are imagining a tetrahedron resting on three points, with whatever forces they initially apply to hold it in place (do we include gravity?), and then you reach in, push or pull the fourth corner. Knowing the forces being applied before you touch it may give you enough extra constraints to say what happens when you do. But you will probably have to introduce more assumptions. Suppose, for instance, that the corner mounts involve springs, or elastic materials of different stiffnesses. The extra force each applies due to a small displacement may not be evenly divided, and may depend on the direction, too.

This is probably how I would approach the problem - imagine a tiny set of springs at each corner with a different stiffness in each direction, and consider the forces and torques generated at each by a small displacement. It sounds interesting! Good luck!

Answer 2

In the below picture the undeformed original frame with infinitely rigid ( Modulus of Elasticity E$ \to \infty $ ) welded metal bars of some assumed cross-section and lengths loaded at apex by 5 tons and fixed on ground... are shown. No deformation or stress occurs. Also no such material exists.

In order to solve for deformation and stress in general we apply six equations of static equilibrium.. three moments and three forces sum taken with respect to any convenient point or axis do vanish:

$$ \Sigma F_x=0, \Sigma F_y=0, \Sigma F_z=0~ ;$$ $$ \Sigma M_x=0, \Sigma M_y=0, \Sigma M_z=0~ ;$$

The same frame of steel of practical rigidity $ E\approx 20,000 ~ kg/mm^2, $ structure with same 5 tons is applied at apex , found to be deformed as shown with a maximum deformation 10.6 mm at apex.

A structural software finds forces/moments along directions of tetrahedral elements. Stress results are not shown.

Forces at 3 fixity points in global coordinate system are:

Answer 3

The solution really depends on the constraints of the tetrahedron. If there are 3 spherical hinges at the 3 vertices, each constraint is equivalent to a force, that in 3D space has 3 components.

The equilibrium equations for a rigid body for translation and rotation, can be recast as 6 scalar equations.

Thus, since you have 6 linear equations with 9 unknowns (3 components per 3 forces at the vertices), this linear system is likely to be under-determined, so that you can find $\infty^3$ solutions.

If you only have 3 beam elements joining the vertex $D$, with the other three vertices $A$, $B$, $C$ and four hinges at the vertices, the solution is determined:

• the reactions at the hinges are aligned with the axis of the beams

• the equilibrium of the vertex $D$ reads

  $\mathbf{F} + \mathbf{N}_A + \mathbf{N}_B + \mathbf{N}_C = \mathbf{0}$,

  being $\mathbf{N}_i = N_i \mathbf{\hat{t}}_i$. Thus the 3D vector equation has three unknowns, the magnitude of the reactions $N_i$, and thus is a determined system, except fot pathological conditions. The linear equations reads

$t^A_{x} N^A + t^B_{x} N^B + t^C_{x} N^C = - F_x$
$t^A_{y} N^A + t^B_{y} N^B + t^C_{y} N^C = - F_y$
$t^A_{z} N^A + t^B_{z} N^B + t^C_{z} N^C = - F_z$