Recently the user CBBAM asked about the inviscid limit in turbulence and the relation between Navier-Stokes equations and Euler equations when $\nu \to 0$. There I pointed out that Onsager proposed that in this limit the velocity field does not remain differentiable and energy is not conserved in weak solutions of the Euler equations such that $u(x+r)-u(x)\leq C r^{1/3}$.
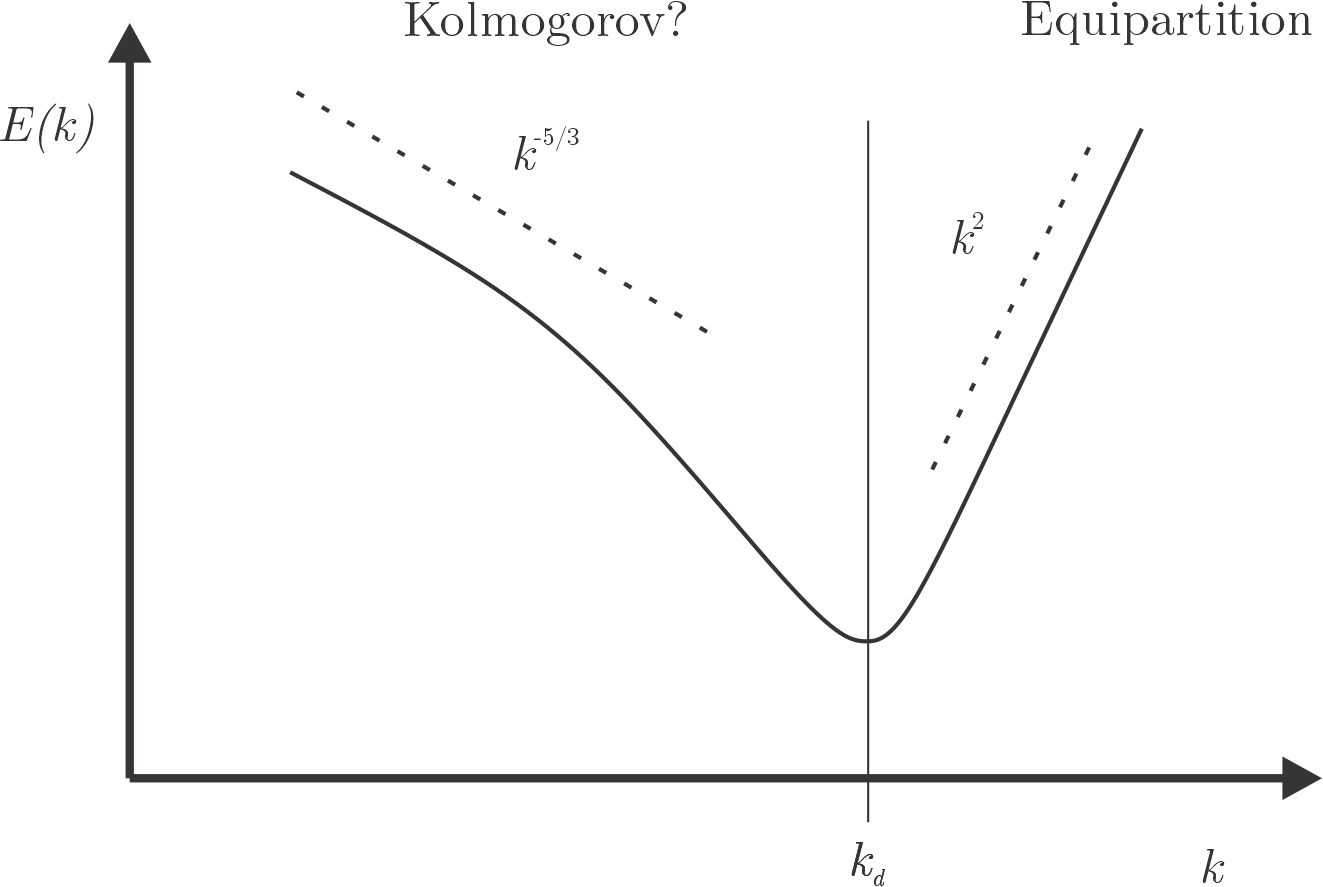
But I came to the realization that I don't really understand the relation between Euler equations and Navier-Stokes turbulence, nor the notion of weak solutions of Euler equations. It is known that in equilibrium there is an equipartition of energy (spectrum of the form $\sim k^2$). In a paper 1 simulations of truncated Euler equations show that this spectrum starts to develop in the small scales as shown in this figure,
They observe that, as time advances $k_d$ moves to the left (to the larger scales) and the equipartition zone gets larger, until an absolute equilibrium is reached. Now, as I understand this, the flow of energy to the equipartition (flow through the $k_d$ shell) could be interpreted as an effective dissipation, is this correct?
Another doubt that I have is, in the $\nu\to 0$ limit the inertial range extends infinitely, this would mean that fully developed turbulence corresponds to Euler solutions where $k_d$ is infinite, so that in no finite time the equipartition range would reach a finite value?
The interpretation I've read is that the energy cascade emerges in Navier-Stokes solutions because the system tries to reach the equipartition, but the viscosity stops de development at the dissipation range. Does this means that the introduction of finite viscosity is equivalent to a coarse graining of the Euler solutions? Could the inertial range dynamics be described by a coarse graining of the Euler solutions?
1 Cichowlas, Cyril, et al. "Effective dissipation and turbulence in spectrally truncated Euler flows." Physical review letters 95.26 (2005): 264502.