1
$\begingroup$

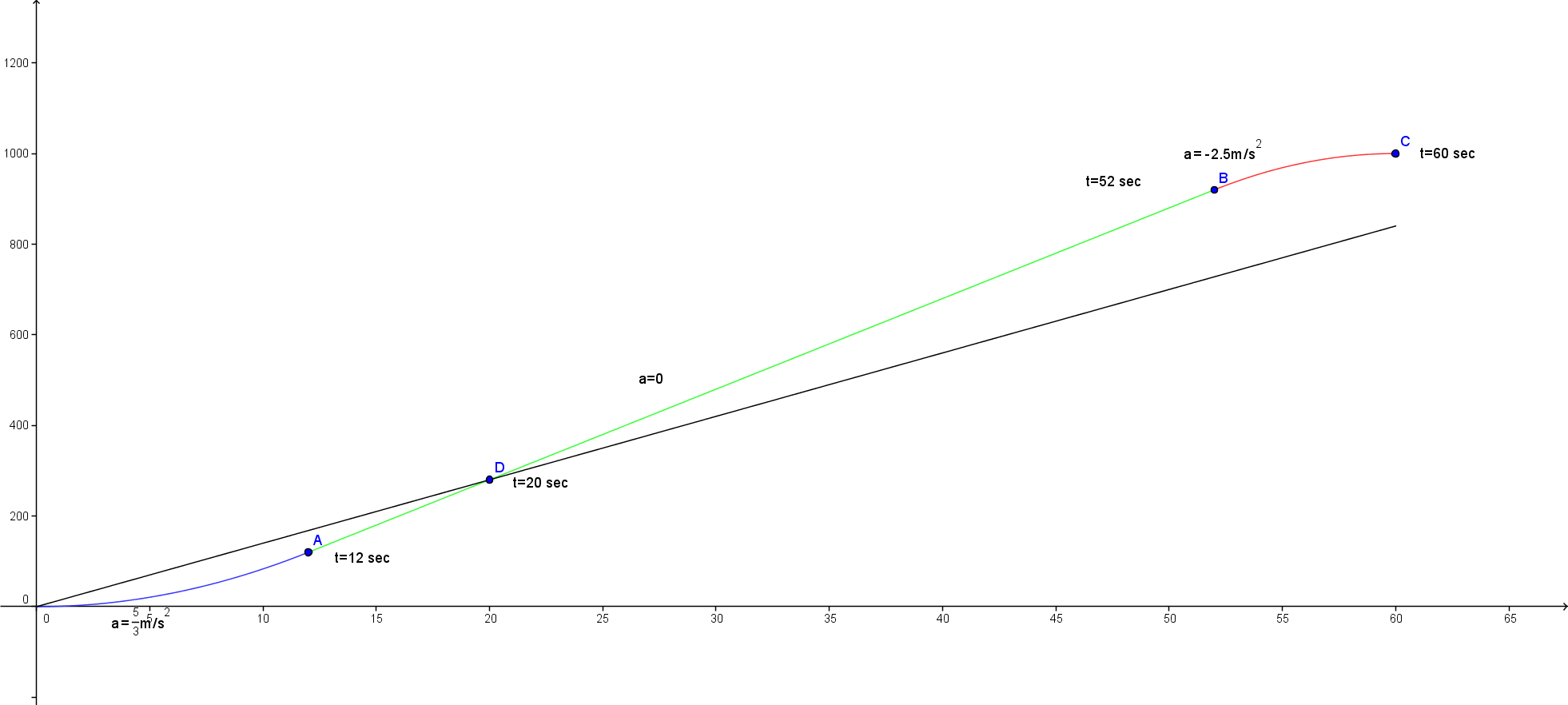
I have tried to solve this problem by adding the sum of the displacements during acceleration, constant velocity and deceleration, but it does not work out.

Question:

A car accelerates from rest to $20~\text{m/s}$ in $12$ seconds ($a =5/3~\text{ms}^{-2}$), it travels at $20~\text{m/s}$ for $40$ seconds, then retardation occurs from $20~\text{m/s}$ to rest in $8$ seconds ($a = -2.5~\text{ms}^{-2}$). As the car accelerates an RC car, moving parallel to the car, is moving at $14~\text{m/s}$. When will overtaking occur and what will the distance be? The RC car passes the car just as it starts to accelerate.

I can do this without a problem if acceleration is a constant. Is there a differential equation I can use to compute this as that is my better area or must I stick with the SUVAT equations?

Again, if I could be pointed in the right direction that way I can learn.

Improve this question
$\endgroup$
2
  • $\begingroup$ Sorry about that, it should be in 12 seconds $\endgroup$
    – user
    Commented Jul 28, 2013 at 16:49
  • $\begingroup$ It is 5/3 to be exact $\endgroup$
    – user
    Commented Jul 28, 2013 at 16:56

3 Answers 3

Reset to default
4
$\begingroup$

Since the acceleration changes discontinuously, there is no one way to solve it in one step.

One simplification is to switch to the frame of reference of the RC car. You can do this simply by making the initial velocity of the starting/stopping car $-14$ m/s. The question then reduces to: When is the displacement of the moving car zero again?

if you use SAVTU on the first acceleration, you can find out if $S=0$ in less than 12 seconds. If not, you can find out $S$ after 12 seconds, and then see if you get back to $S=0$ at constant velocity of $6$ m/s in less than 40 seconds, and so on...

Explicit solution added;

First, Setting $s=0$ (car catches RC during first acceleration), $a = \frac{5}{3}$, and $u = -14$, and solving for t, we get t = 16.8 sec

It would take 16.8 sec of constant acceleration for the car to catch the RC, which we don't have!

So, finding s at the end of the first acceleration, if $a=\frac{5}{3}$, $u = -14$, $t=12$, we get $$s=-14 \times 12+\frac{1}{2}\times\frac{5}{3}\times 12^2=-48$$The car is 48 m behind the RC at the end of the firat acceleration.

The car is catching the RC at $6 $ m/s, and will make up the 48 m in 8 seconds of constant velocity, which we do have.

Total time is 20 seconds. The RC will cover 280 m, and so will the accelerating car...

Improve this answer
$\endgroup$
3
  • $\begingroup$ Could you show me how to work out the question using this method as I understand the theory but a worked example would be very beneficial. $\endgroup$
    – user
    Commented Jul 28, 2013 at 18:27
  • $\begingroup$ + for "switch to the frame of reference of the RC car". $\endgroup$
    – Mike Dunlavey
    Commented Jul 28, 2013 at 19:48
  • $\begingroup$ @user2352274, this is a great method to solve such problems too! It helps when plotting graphs becomes pretty difficult. $\endgroup$
    – udiboy1209
    Commented Jul 29, 2013 at 10:01
3
$\begingroup$

Plotting the displacement time graph helps a lot in these kind of problems.

enter image description here

From this graph we can say the RC is ahead of the car initially. The car catches up and overtakes the RC at t=20 seconds.

Note: You do not need graph plotting tools to plot these graphs. Basically, you don't need to plot these graphs to accurately too.
Rough back-of-the-envelope calculations can easily determine the displacement of the car and RC at various time intervals. Then you can use the fact that an accelerated S-T graph would be quadratic, hence a parabola, again giving you approximate plots.

This is enough to determine that the car will overtake the RC during its uniform motion. And then it becomes pretty easy to use SUVAT!

Improve this answer
$\endgroup$
5
  • $\begingroup$ Can I use a VT graph instead as I am more familiar with those. $\endgroup$
    – user
    Commented Jul 28, 2013 at 18:26
  • 1
    $\begingroup$ VT graph won't tell you when the overtaking will occur. You will need an ST graph $\endgroup$
    – udiboy1209
    Commented Jul 28, 2013 at 18:28
  • $\begingroup$ Just so I am clear, the purpose of the st graph is to find whether overtaking occurs during acceleration, constant velocity or retardation. As it occurs during constant velocity I would simply let 14(t) = what? $\endgroup$
    – user
    Commented Jul 28, 2013 at 18:36
  • $\begingroup$ They both have to cover the same distance to overtake each other, right. So $14t=s_1+s_2$ where $s_1$ is the displacement of the car during initial accelerated motion and $s_2$ is displacement during constant velocity of $20m/s$. Gives you $14t = \frac 12 \frac 53 (12-0)^2+ 20(t-12)$ $\endgroup$
    – udiboy1209
    Commented Jul 28, 2013 at 18:42
  • 1
    $\begingroup$ Gotcha, now I get it, before I had no constant because I did used t^2 in place of 12-0. Thank you sir $\endgroup$
    – user
    Commented Jul 28, 2013 at 18:49
0
$\begingroup$

The car accelerates from 0 to 20 m/s in 12 s. It has an average speed of 10 m/s over that time, and so covers 120 m in that time. In that same time, the RC car goes 14 m/s * 12 s = 168 m. So the car didn't catch up while accelerating. At 12 s, the car is 168 m - 120 m = 48 m behind the RC car.

The car is now going a constant 20 m/s, whereas the R/C car is going 14 m/s. So it can catch up. The difference is 6 m/s. The car has 48 m to make up, so that takes 48 m / 6 m/s = 8 s after it finishes accelerating, or 20 s after it starts. This is long before the car begins to decelerate, which is 40 s after it's done accelerating.

Therefore the car catches up at 20 s, which is at 14 m/s * 20 s = 280 m.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.