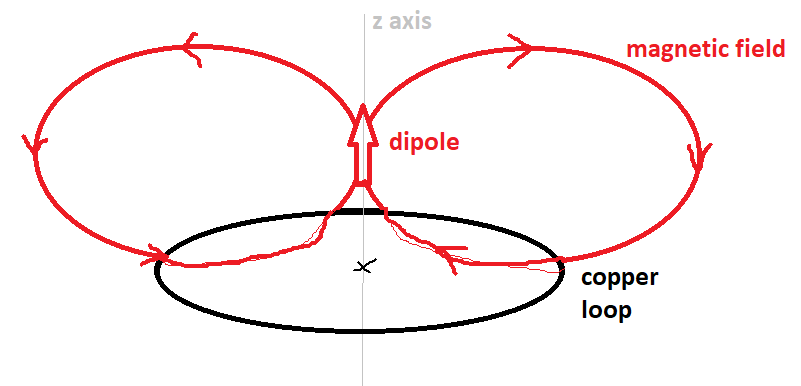
We have a magentic dipole parallel with z-axis (shown on the diagram below) falling through copper loop, because of changing magnetic flux there is induced current in cooper ring/loop and consequently we get magnetic force on ring/loop and on our dipole (because of Newton's thrid law).
Lets start calculating force on our dipole with $$F_{dipole} = - \int dF_{ring} = -\int I(\vec{dl} \times \vec{B})$$ we know that $\vec{dl} = R d\phi \hat{\phi}$ and $\vec{B} = B_z \hat{z} + B_{\rho}\hat{\rho}$, continuig with integration... $$= -\int_0^{2\pi} IRB_z \; d\phi \; \hat{\rho} +\int_0^{2\pi} IRB_{\rho} \; d\phi \; \hat{z} = -F_{\rho} \hat{\rho} + F_z \hat{z}$$
We get that there is a forcce $F_{\rho}$ that tries to compress the dipole in all directions, but my intuition tells me that force in radial direction should be zero ($F_{\rho} = 0$). Where did I go wrong? Plese explain, thank you very much in advance.