Reason why light beam hits detector

Question

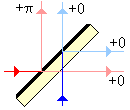
I was thinking about the basic structure of interferometers and I watched a video about it. I came across a simple illustration that looked something like this:

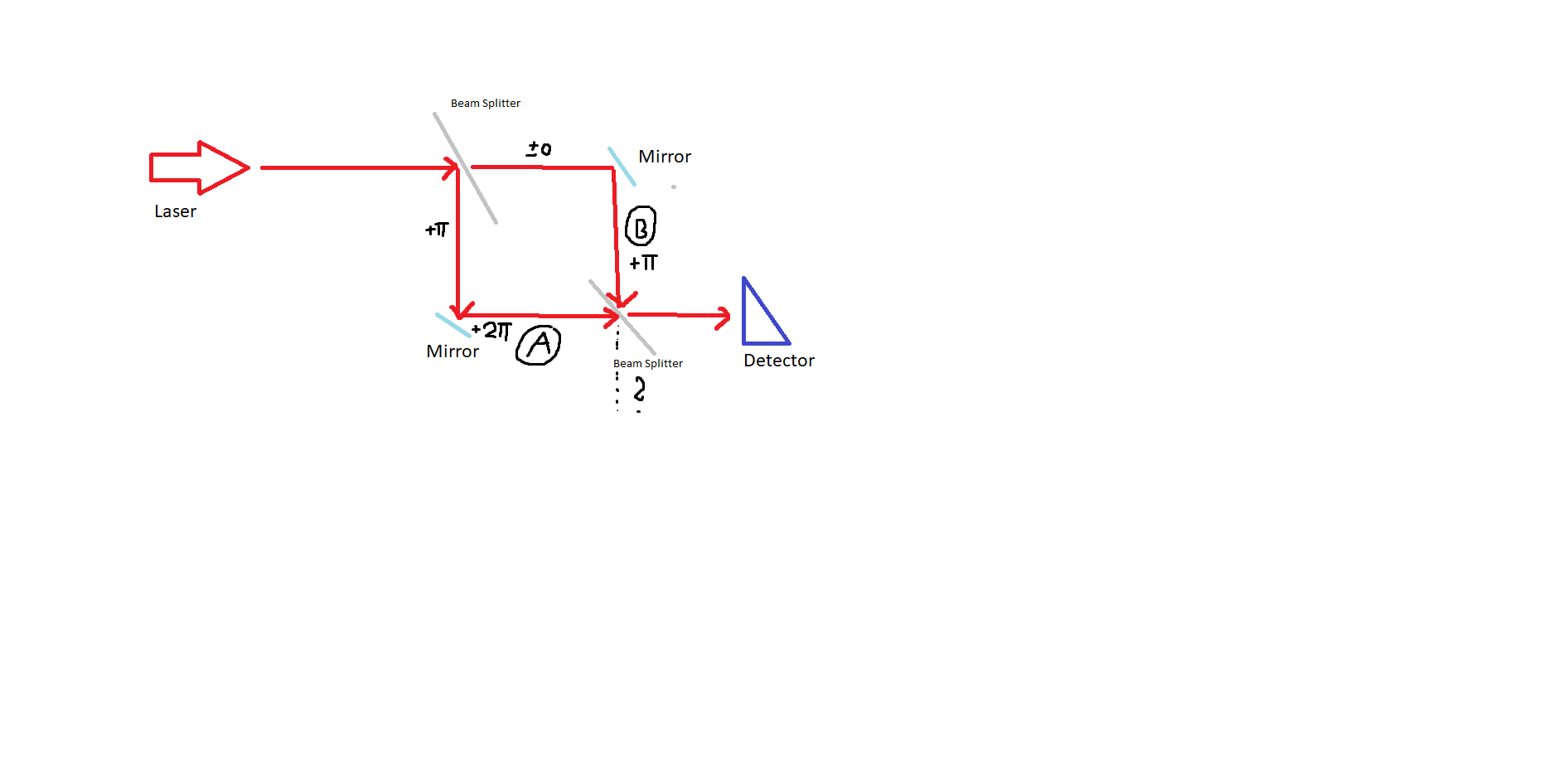A laser beam is split into two seperate beams with half intensity by a beam splitter, each with probability 1/2. The reflected beam should have a phase change of +π. The transmitted beam should have no phase change. Both beams hit a mirror at 90 degrees, and both get shifted by +π again, and hit another beam splitter. My question is: Why does the whole recombined beam hit the detector?

To my understanding, beam A) should hit the second splitter and be transmitted (so it stays at phase change +2π) and be reflected (resulting in phase change +3π), and beam B) should be transmited (stay at phase change +π) and be reflected (resulting in phase change +2π). So there should be two resulting beams; there should be no destructive interference.

Where is my reasoning wrong and why is there destructive interference athe the second beam splitter resulting in the detector registering the whole original beam?

Thanks in advance

Answer 1

There are two misunderstandings in your reasoning:

The interference is not introduced by the reflection phase shift on each mirror but by the difference of optical path length between the two beams. If the path of A and B have the same optical length, there is no interferences.

The superposition of two beams of light is always a beam of light. What is at stake in light beam superposition is light intensity. If the two beams interfere, the pointwise intensity of the sum of the two beams is not the sum of the pointwise intensities of each beam. The intensity is spatially redistributed; some places are bright other are dark.

Answer 2

What you are trying to do, understand a Mach-Zehnder interferometer by just looking at the phase shifts cannot be understood using 1D unspecific beam splitters. This educational paper: How does a Mach-Zehnder interferometer work? (Zetie et al, Teach. Phys. 2000) says it is a common misconception.

If you consider that the beam splitter is made thick glass with a dielectric coating, the beam reflecting inside the glass does not introduce a phase shift. As found in Wikipedia article for beam splitters with this image:

In the Mach-Zehnder article of Wikipedia, they use a picture that makes it more clear on which side is the mirror is in each beam splitter :

Now try to count the phase shifts by taking into account that if the beam reflects inside the glass it does not change its phase.

The upper path that goes into detector 2, gains only $2\pi$ phase shifts, while the lower path going into detector 2 only gains $1\pi$, providing a phase difference of $\pi$ resulting in destructive interference.

For detector 1, both beams gain the same phase shift = no phase shift, thus constructive interference.

Note that these are the kind of beam splitter used for this interferometer. Other systems may require beam splitters that are more symmetrical in their phase shift.