Doesn't particle diffraction prove that electron beams show wave-like properties, not a single electron?

Question

I'm reading the experiment for the diffraction of an electron on double-slits and this section of my textbook confuses me. the diffraction pattern isn't shown by a single electron its shown by multiple.

How does this experiment show that a single electron has wave-like properties?

And what does this quote from my textbook mean:

First, we need a source of particles, for instance, an electron gun. By controlling the energy of the electron, the wavelength is varied. Each electron has a random phase angle with respect to every other electron. Consequently, two electrons can never interfere with one another to produce a diffraction pattern. One electron gives rise to the diffraction pattern, but many electrons are needed to amplify the signal so that we can see the pattern. A more exact way to say this is that the intensities of the electron waves add together rather than the amplitudes.

Are there any experiments that show a single electron with a diffraction pattern through a double slit? Not a diffraction pattern made by electrons through a slit.

Answer 1

The thing about quantum mechanics is the fact that each quantum can only interact locally. Therefore, if we only send one electron through a double slit we can only see one little spot at the back where it interacts with the observation screen. From that single spot we cannot learn much. The location of the spot is governed by some probability distribution which is predicted by the theory, but one single spot cannot give us enough information to see the whole distribution.

So, to see the whole probability distribution, we need to build up statistics. In other words, we need to repeat the same experiment over and over again, every time sending one electron through the double slit. In this way, we get more and more spots. Eventually the distribution of these spots can be compared with the probability distribution that our theory predicts. This is how all (or at least most) quantum experiments work, because our quantum theory predictions always (or usually) are in the form of probability distributions.

Answer 2

The successive electrons in the experiment are assumed to be statistically independent; they need to be "unrelated" to each other. Physically, we try to do this by various methods, such as

• production from a hot (i.e. noisy) thermal system (consider that the classic "electron gun" is essentially an incandescent lightbulb plus extras)
• separation in time
• separation in space (mostly follows from time separation if the electrons are fast)

If the electrons are still related to/communicating with each other after we've done everything we can to separate them, then there's some force/phenomenon responsible for it which we should be able to detect. If we're not seeing anything then the electrons should be independent.

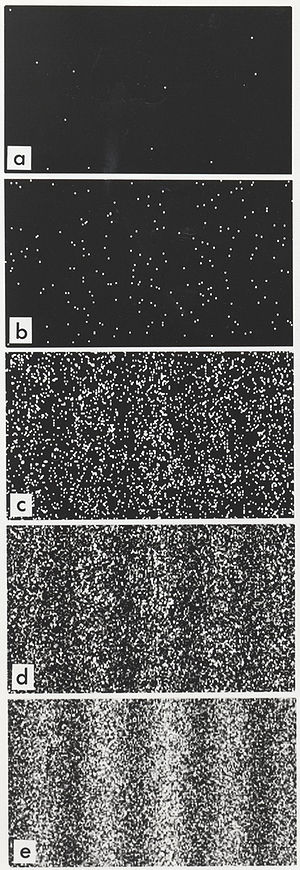
Once we have a supply of independent electrons, we can perform the double-slit experiment on them, taking care to keep them separate and to keep the apparatus itself from "remembering" anything between shots. Once we are done, we will be able to say "it was a property of our electron collection that it produced so-and-so distribution on our detector screen." Because our electrons were independent, we can go further and say "any one electron put through the experiment will have so-and-so probability of landing in any given position."

(The precise reasoning for the above is: for any one electron, there is some probability distribution for where it will land on the screen, even if we don't know it. If we have a beam of independent electrons, then the pattern it makes on the screen is completely determined by the single-electron distribution. If we measure the pattern, we can invert the relation to learn about the single-electron distribution. Without independence, the relation between beam pattern and single-electron distribution is broken.)

It is of course impossible to do the double-slit experiment with one electron and get a result of much confidence. Compare a similar macroscopic question:

You have a coin that will blow itself up after you flip it once. Can you tell whether the coin is fair (50/50) or not by flipping it?

The electron is consumed by the detector screen analogously to this coin. With just one trial, you cannot get a good picture of the probability distribution.

Answer 3

You have found a very common mistakes in low-end (e.g. YouTube) explanations of YDSE. The electron always acts like a wave. When you have which-way information, the pattern on the screen is two overlapping diffraction patterns:

$$ \psi_{inc}(\theta) = ||A_L(\theta)||^2 + ||A_R(\theta)||^2 $$

where the $A({\theta})$ are the amplitudes from each slit.

When you don't have which way information:

$$ \psi_{inc}(\theta) = (A_L(\theta) + A_R(\theta))^*(A_L(\theta) + A_R(\theta))$$

$$ \psi_{coh}(\theta) = ||A_L(\theta)||^2 + ||A_R(\theta)||^2 + [A_L(\theta)^*A_R(\theta) + A_R(\theta)^*A_L(\theta)]$$

where the term in square brackets represents interference.

Note that the wide diffraction terms seen in the incoherent case are identical in the coherent case.

It's always a wave formed by summing the amplitudes coherently over all allowed paths. In the case of single slit diffraction, that is an integral over the width of the slit, and that looks just the definition of a Fourier transform of the aperture profile.

Answer 4

Doesn't particle diffraction prove that electron beams show wave-like properties, not a single electron?

Here is an experiment one electron at a time, the total accumulation showing the diffraction. Its newest version is here.

This answer of mine is relevant

The individual electrons leave a point on the screen which seems random. The accumulation gives a probability distribution that has sinusoidal variations.

The wave like properties of the electron depend on the wave function of the solution of the quantum mechanical boundary conditions for a given problem. It is the probability of finding an electron at (x,y,x,t) that has wave like properties and shows the diffraction pattern.

You ask:

How does this experiment show that a single electron has wave-like properties?

It does not. It shows that an accumulation of same momentum electrons interacting with the same boundary condition shows a diffraction pattern, but if it were analyzed one electron at a time it would be like the experiment shown above.

Answer 5

You say...

If I understand correctly, only on electron 'decides' the diffraction pattern; which, is an assumption based on having ruled out the possibilities of interference from other electrons. And all the others just intensify the diffraction pattern the first electron 'chose'. The question then becomes how do the other electrons know to intensify it?

The electrons don't 'know' to intensify pattern and the first electron doesn't 'decide' anything.

What the experiment shows is that if you fire individual electrons through slits there is some factor at work that causes them to build up a diffraction pattern. The electrons cannot be interfering with each other, because they are never passing through the slits at the same time. That means that the factor that causes the diffraction pattern must be at work on the individual elections one at a time.

More specifically, you can perform experiments in which you vary the slit width, vary the distance between the screen with the slits and the detector screen, and vary the energy of the individual electrons.

What you find when you do that is a relationship between all three that suggests that the probability of a single electron hitting the rear screen at a given spot is consistent with the idea that associated with each electron is a function in the form of a travelling wave that interferes with itself upon passing between the slits, where the wavelength of the function is related to the momentum of the electron.

That does not mean that the electron 'is' a wave- it just means that the electron's behaviour can be predicted by calculations in which it is assumed that the electron has an associated wave function. The physical significance of the wave function is still a debated question in physics.