0
$\begingroup$

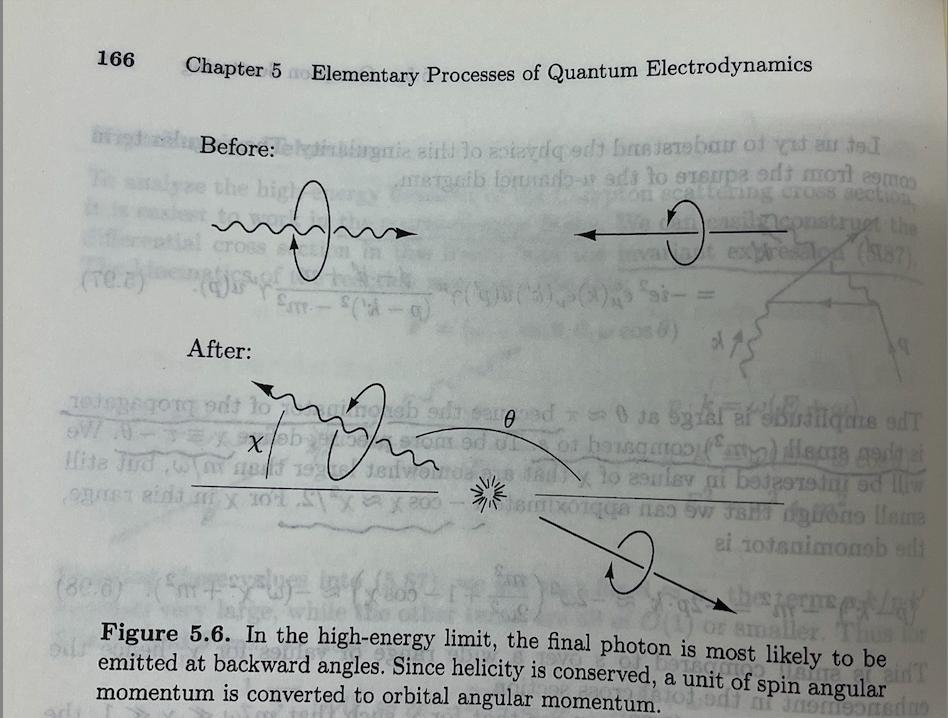
I have two points not clear in Peskin & Schroeder's QFT on page 166.

  1. On Figure 5.6, "Since helicity is conserved, a unit of spin angular momentum is converted to orbital angular momentum", I am really puzzled on this sentence. In my understanding, in this Center-of-Mass frame, the initial total spin is zero, also the finial total spin is zero. For the orbital angular momentum, I see that the "back-scattering" probability is large, so their need to have some orbital angular momentum in principle. But I am really puzzled on how this one unit of spin angular momentum converted to orbital angular momentum. By the wave, the book mentioned the final states is a "p-wave", also what is this "p-wave"?

enter image description here

  1. I also troubled with the sign in eq.(5.101), I thought their need to have a "minus" sign, the reason as follows: for the equation above (5.101), I thought it should be $$ \bar{\sigma}\cdot (p-k^{\prime})\simeq -\bar{\sigma}^{1}(p-k^{\prime})^{1}=\sigma^{1}\cdot (-\omega\chi) $$ so it has a "minus" sign difference with book, and we have used the approximation $$ p-k^{\prime}\simeq (0,-\omega \chi,0,0) $$

If you have any comments on above questions, I am really appreciate it.

Improve this question
$\endgroup$
4
  • $\begingroup$ I answered your first question. For your second question in the second inequality the minus sign is already coming from the fact that the spatial 1 component of the 4-vector $\bar{\sigma}$ is negative so that is why P and S already don't have a bar in the second line (unlike in your question). $\endgroup$
    – octonion
    Commented Jul 17, 2022 at 5:26
  • 1
    $\begingroup$ @octonion Thanks for your detailed explanation about the first question. While for the second question, we know the definition of $\bar{\sigma}=\{1,-\sigma^{1},-\sigma^{2},-\sigma^{3}\}$, so their have a minus sign, and another minus sign is from $g^{\mu \nu}$, so I thought it should be $\sigma^{1}\cdot (p-k^{\prime})^{1}$. Is their still something that I missing? $\endgroup$
    – Daren
    Commented Jul 17, 2022 at 9:03
  • $\begingroup$ Good point, perhaps they really do have a sign error in their expression. They end up squaring the amplitude to compare with the cross section so they might not have noticed. $\endgroup$
    – octonion
    Commented Jul 17, 2022 at 12:11
  • $\begingroup$ @octonion Thanks！Yes when they squared the amplitude, the differential cross section is invariant. $\endgroup$
    – Daren
    Commented Jul 17, 2022 at 13:51

1 Answer 1

Reset to default
1
$\begingroup$

The total spin about the z-axis in the initial state is +1/2 (photons have spin 1), and in the final state it is -1/2 (if $\chi=0$, to be exact), so there must be some additional +1 orbital angular momentum about the z-axis in the final state compared to whatever was there in the initial state.

The amplitude is calculated in terms of well defined initial 3-momentum pointing along the z-axis, and final momentum pointing in a direction specified by the angle $\chi$. But to really talk about orbital angular momentum we ought to expand in terms of spherical harmonics. Consider a table of spherical harmonics. Notice that all the spherical harmonics vanish at $\theta=0$ unless $m=0$, so even though there is not a well-defined initial total orbital angular momentum $l$, there is definitely initially zero z-component.

So we know the $z$ component in the final state should be $m=1$, and if you again take a look at that table you'll notice all of the $m=1$ spherical harmonics are proportional to $-\sin\theta\approx \chi$, which is consistent with Peskin and Schroeder's calculation. By the way ``p-wave" just means the $l=1$ orbital angular momentum state, and it is a slight abuse of terminology in my opinion since what is really well-defined is that $m=1$, not the $l$ value.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.