1
$\begingroup$

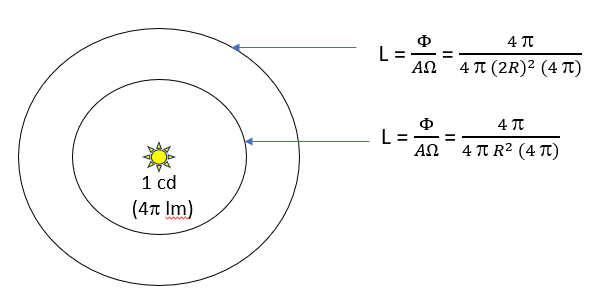
Recently, I learned that apparently both radiance and luminance are independent of the distance between the light emitting object and the observer.

The reasoning was that although the radiant/lumninant intensity decreases proportionally to the square of the distance (inverse square law), the solid angle subtended by the light emitting object as seen from the observer decreases by the same factor, thus, both factors cancel each other out so that radiance/luminance are always equal irrespective of distance.

There was, then, the example that if I have two equally light street posters with one being farther away from the other they would still look equally bright since the eye apparently measures luminance.

This made wonder: If radiance/luminance does not depend on distance to the observer, why aren't star in the night blindingly bright? There are many stars far brighter than our sun, shouldn't looking at those stars in the night sky be like looking into a laser?

Another logical consequence would also be that we should see stars brighter than our sun when at daytime since those stars would outshine our sun.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ I can assure you, that 2 equally lit lamps, at different distances, would not appear equally bright. $\endgroup$
    – jensen paull
    Commented Jun 23, 2022 at 9:51
  • $\begingroup$ Although I am not familiar with the specific terminology. It all boils down to EM, for a point source atleast. the average poynting vector falls like $\frac{1}{r^2}$The poynting vector integrated about some spherical surface tells the total power radiated. A spherical surface grows like r^2, thus the total power radiated at any distance is a constant! This is a consequence of conservation of energy. The energy is not lost, only spread out over a larger area. I would asume that is what your text is talking about. Since ofcourse a light apears less bright farther away as per $\frac{1}{r^2}$ $\endgroup$
    – jensen paull
    Commented Jun 23, 2022 at 9:58

5 Answers 5

Reset to default
2
$\begingroup$

This is a vocabulary problem. There are 3 related concepts - Radiant Flux, Radiant Intensity, and Radiance. Likewise there are 3 more for luminance.

Helpfully, there are a variety of names that each can be called.

The name here that sounds most like "brightness" is Radiant Intensity. The one that is actually most like it is Radiant Flux.

Improve this answer
$\endgroup$
1
$\begingroup$

I think the missing link is that the posters are extended objects. Ok, that's true for the stars as well, but the posters appear as extended objects. The further away, the smaller, but the photons per pixel (if you take a photo...) is constant, at least for pixels that are fully filled with the poster. Precondition is of course, that the posters are light sources having the same radiance (so not reflecting light from any source having different distances to the posters or so...). The stars on the other hand are point sources, they are not resolved. So the compensation of seeing more light emitting area per pixel does not work.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I think the approximation that stars are point sources doesn't hold here. For example I can look at a star through a telescope and see it as ball with finite solid angle, while still not getting blinded by it. $\endgroup$
    – tempdev nova
    Commented Jun 23, 2022 at 16:45
  • 2
    $\begingroup$ Resolving stars is nothing you can achieve with your amateur telescope. They achieved this with the large telescopes in Chile, for example, putting them together and do interferometry, but that's a lot of effort. I found a list here with resolved stars en.m.wikipedia.org/wiki/List_of_stars_with_resolved_images. What you see with a usual telescope is most likely saturated pixels or so, but not resolved stars. The effect is that you multiply the radiance with a larger area (the lens) compared to your eye, therefore getting much more photons, but that's not what I meant... $\endgroup$
    – Charles Tucker 3
    Commented Jun 23, 2022 at 17:31
0
$\begingroup$

When you buy a flat screen tv. It has a certain luminance rating. It doesn’t matter how far you are from the tv. The rating of the tv is the same.

When you have an optical system it is useful to have a measure of the light energy as a function of the wavelength and since the light leaves the source spreading out geometrically like sphere if it is a point source, or like a cone if it is from an aperture it is useful to use a solid angle. When you make that kind of measurement it is the spectral radiance. If you factor in the response of the human eye then it is luminance. The units confuse everyone and to make good measurements is actually kind of tricky even if you are careful.

Both luminance and radiance are meant to be geometrically invariant. This means that if you measure the light source near its origin and take the geometry into account you should get the same value if you made the same measurement with your detector farther away. There will be less light on the detector, but you include the increased distance in you calculation.

If you measured the radiance or luminance of a beam of light, and then put a lens in the beam. And measured the system at the spot where the the light was focused you would have more light on the detector but you would have to take the geometry (solid angles etc ) into account and you should get the same value for the radiance or luminance of the source.

So the star has a certain amount of energy leaving it. How many photons reaching your eye would depend on how far away and the amount that energy. To figure out the radiance or luminance (people also used to use the term Brightness) and make the measurement on earth you would need to know how far away you were.

If you know the radiance and luminance of a source and you want to know the amount of light falling on a surface you would start a calculation with that and then have to include the distance and area of the surface to find how much energy is hitting the surface.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Thats pretty much what I stated. So my question is why then when we look at stars in the night sky don't they appear extremely bright? There are many stars far brighter than our sun yet we get no eye damage when looking at them, but we do get eye damage when directly looking at the sun. $\endgroup$
    – tempdev nova
    Commented Jun 23, 2022 at 11:13
  • $\begingroup$ They are much farther away than the sun. When you buy a lightbulb it has a rating like 60 watts ( for the electrical power) and it also has a luminance for how bright it looks for the eye. You can buy a 100 W bulb and it will be brighter. If you put the w 100 watt (higher luminance ) bulb farther away it is still a 100 watt bulb and it’s luminance hasn’t changed, but it will look dimmer than the 60 watt bulb since not as much light will reach your eye. The stars are still brighter, but not as many photons reach the eye. $\endgroup$
    – UVphoton
    Commented Jun 23, 2022 at 11:22
  • 1
    $\begingroup$ So a farther away light source does appear dimmer than a closer one? $\endgroup$
    – tempdev nova
    Commented Jun 23, 2022 at 11:25
  • $\begingroup$ Yes, the poster example as it is written above is wrong. First the posters are reflecting light not emitting light. The posters are not a light source. If you had a light shining on the two posters and were looking at them, the one farther away would have fewer photons bouncing back to your eye and would look dimmer because of the geometry. The luminance of the light source would be the same. If the posters are identical the percent of photons that reached them would be the same, but ithe poster would look dimmer because fewer photons reach the eye. $\endgroup$
    – UVphoton
    Commented Jun 23, 2022 at 11:37
  • $\begingroup$ However the poster would also appear smaller to the eye due to perspective, meaning that less light reaches a smaller area. To my knowledge the eye measures light/area so both factor should cancel each other out? $\endgroup$
    – tempdev nova
    Commented Jun 23, 2022 at 12:22
0
$\begingroup$

Obviously that the farther you are from the light source, the lower the brightness you see.

Exampe 1 cd source.

From Wiki, Luminance is invariant "in geometric optics"，It means that when your light go through an optical element , before and after this optical element, the luminance doesn't change by it. but in my case and yours, the luminance does change from the distance. The rate of brightness decays depends on the solid angle.

Improve this answer
$\endgroup$
0
$\begingroup$

A uniformly bright surface will appear equally bright, no matter how far away it is. That is because this brightness (radiance) is a flux per solid angle and both of these decrease as the square of the distance in the same way.

This only remains true however if you can spatially resolve the surface. When we look at a star, even with a telescope, it remains unresolved. That means the solid angle subtended by the star is the same no matter how far away it is. This is a simple consequence of the diffractive nature of the optics and imperfections in the imaging system. As a result, the "brightness" of a star - which is actually its integrated flux over the unresolved image formed by the imaging system, decreases as the square of the distance.

If we could take resolved pictures of stars, then their surface brightness (e.g. photons per resolved pixel on the detector) would indeed be a constant for a given star (ignoring issues like extinction).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.