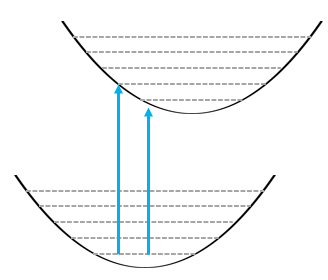
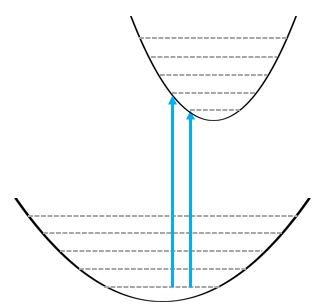
In molecular quantum mechanics, it is very common to model a diatomic molecule as a two-level harmonic oscillator with vibrational levels lying within the electronic states:
In most of the textbooks out there, it is assumed that the curvature of the ground and excited states have the same curvature, (i.e. described by the same harmonic oscillator). The Born-Oppenheimer approximation allows us to write the ground state as $\left|g\right>\otimes\left|n\right>=\left|g,n\right>$ and similarly the electronic state as $\left|e\right>\otimes\left|n\right>=\left|e,n\right>$. From the expressions written down here, we see that the Hilbert space of a molecule is $\mathcal{H}_{molecule}=\mathcal{H}_{electronic}\otimes\mathcal{H}_{vibration}$.
However, we know in general that the nuclear potentials in the ground and excited state of a diatomic molecule do not have the same curvature (the excited state's potential is usually narrower). So how would the Hilbert space and the states look like in this general picture?
I expect it to be something like $\left|g\right>\otimes\left|n\right>=\left|g,n\right>$ and $\left|e\right>\otimes\left|n'\right>=\left|e,n'\right>$ with different vibrational quantum number $n$ and $n'$. But then this would mean that the Hilbert space is now $\mathcal{H}_{molecule}=\mathcal{H}_{ground}\otimes\mathcal{H}_{vibration}+\mathcal{H}_{excited}\otimes\mathcal{H'}_{vibration}$.
Is this correct? Or should it be something like $\mathcal{H}_{molecule}=\mathcal{H}_{electronic}\otimes\mathcal{H}_{vibration}\otimes\mathcal{H'}_{vibration}$?
On top of this, if the molecule now absorbs a photon and resulting in a vibronic transition (i.e. $\left|g,n\right>\rightarrow\left|e,n'\right>$), then it seems like the molecule jumps from one Hilbert space ($\mathcal{H}_{vibration}$) to another Hilbert space ($\mathcal{H'}_{vibration}$).
How would the Hilbert space look like for this general case? I couldn't find a good discussion on this topic so any insights will be greatly appreciated.