What happens when light gets reflected and refracted at the same point?

Question

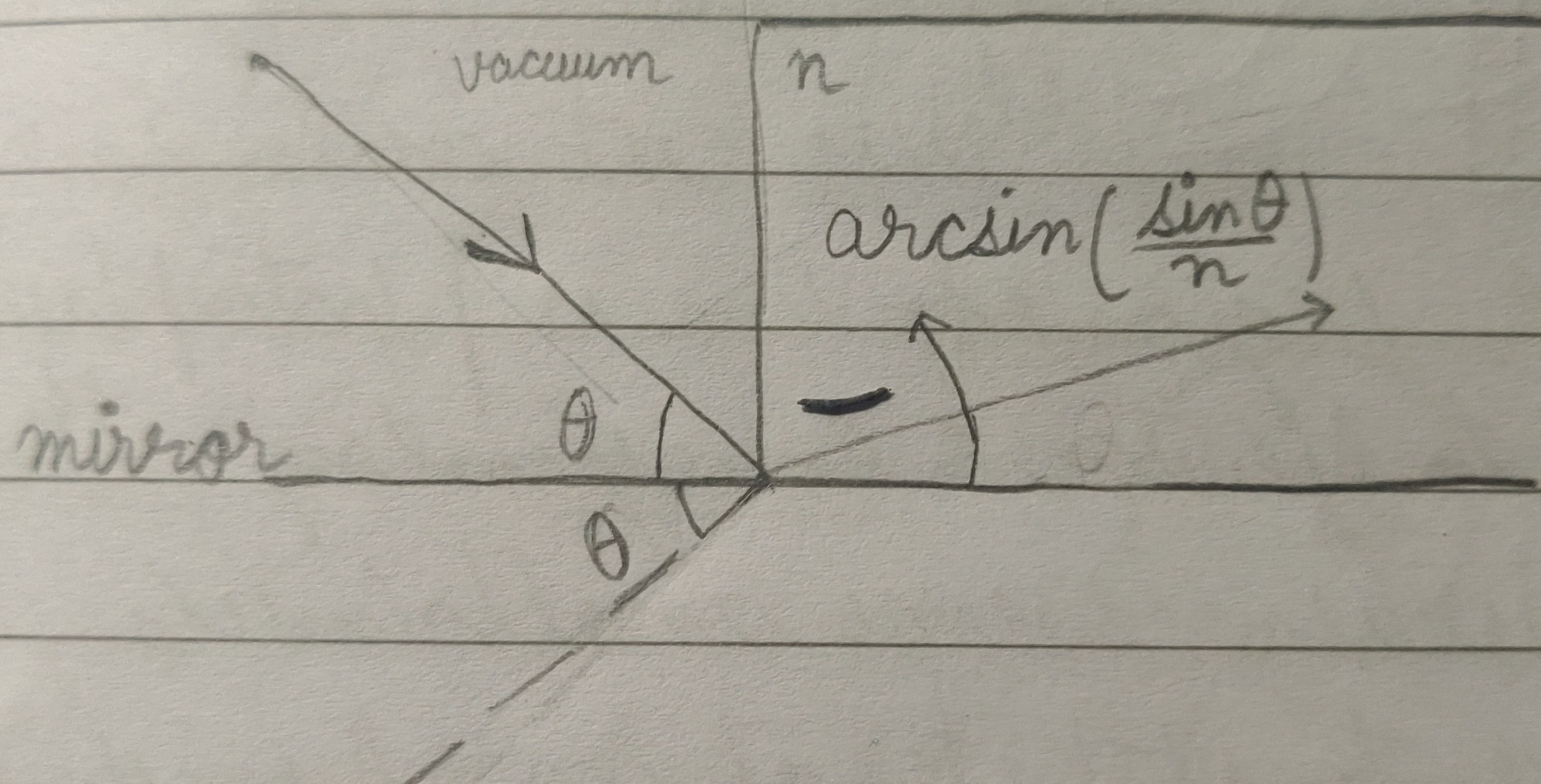
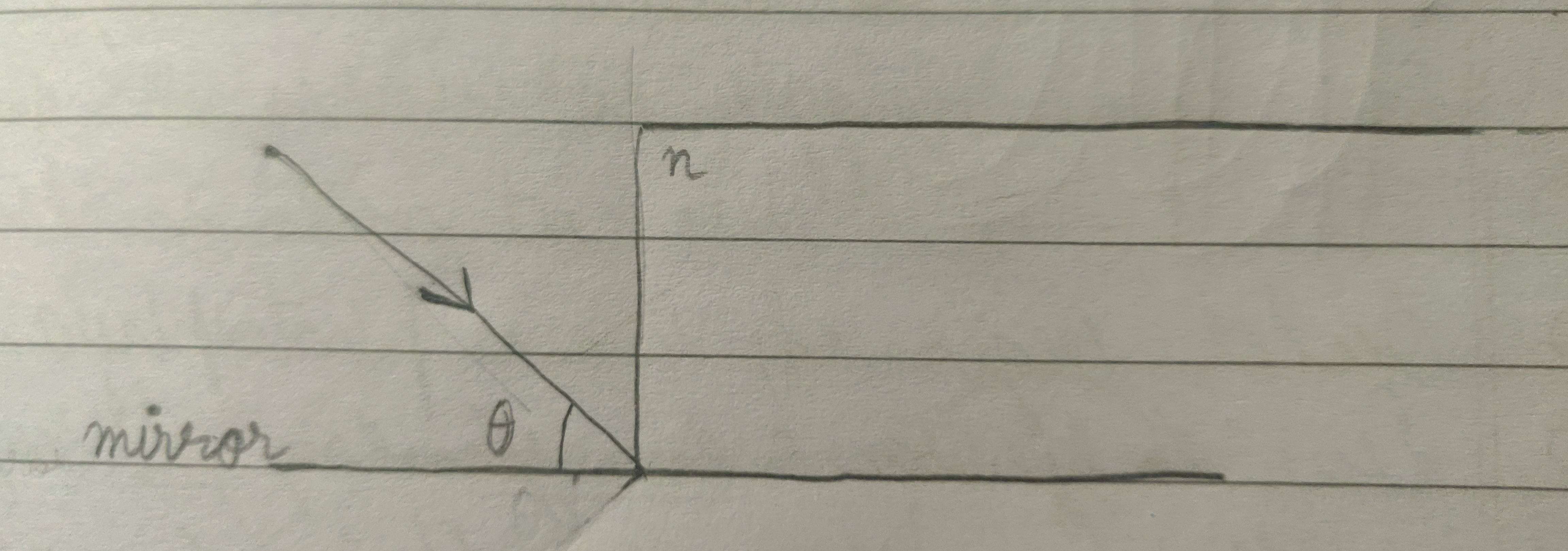
Suppose that medium of refractive index n is placed on a mirror (as seen from the image), and the rest of the place is vacuum. A light ray is incident on the common point of vacuum, glass and mirror. What would be the path of light after reflection.

What seems sensible by symmetry to me is that refraction would take place as if light is coming from 'behind' the mirror. Thus, the angle between the mirror and refracted ray would be:\begin{align}\arcsin{(\frac{\sin\theta}{n})}\end{align} But I cannot justify this, nor am I certain about this.

Answer 1

A perfectly narrow ray perfectly intersecting a point such as the one you’ve drawn is unphysical. Reflection and refraction are reliant on phase matching at a boundary, in which is implicit a length along the boundary over which the phase is to be matched.

That said, your intuition is correct. You can see this by computing what the rays a little to the left and right of the intersection point do (after all, real EM waves have finite width). Whether the wave hits the mirror first then refracts, or the other way around, the result is the same.

Answer 2

Your assumption is perfectly right here. When light falls on any surface a part of it is reflected, a part of it is refracted and the rest gets absorbed. If you try to visualize the picture in your mind (try water and glass for easier imagination) it should probably be clearer to you.

Light is not a line meeting a point, what it really is. - is a form of energy that propagates through the reflector and refractor surface in a wave-like manner. so when it's incident on the connecting point between the reflecting surface and the reflector surface a part of the energy will interact with the reflector resulting in a reflected ray and another part of it will interact with the refractor resulting in a refracted ray.