Checked around a buch and could not find any help. But I needed help with:
Understanding that if I get the Inverse FT of K-space data, what is the scaling on the X-space (object space) resultant image/data i.e. for every tick on the axis, how do I know the spatial length?
More detailed explanation in the below image.
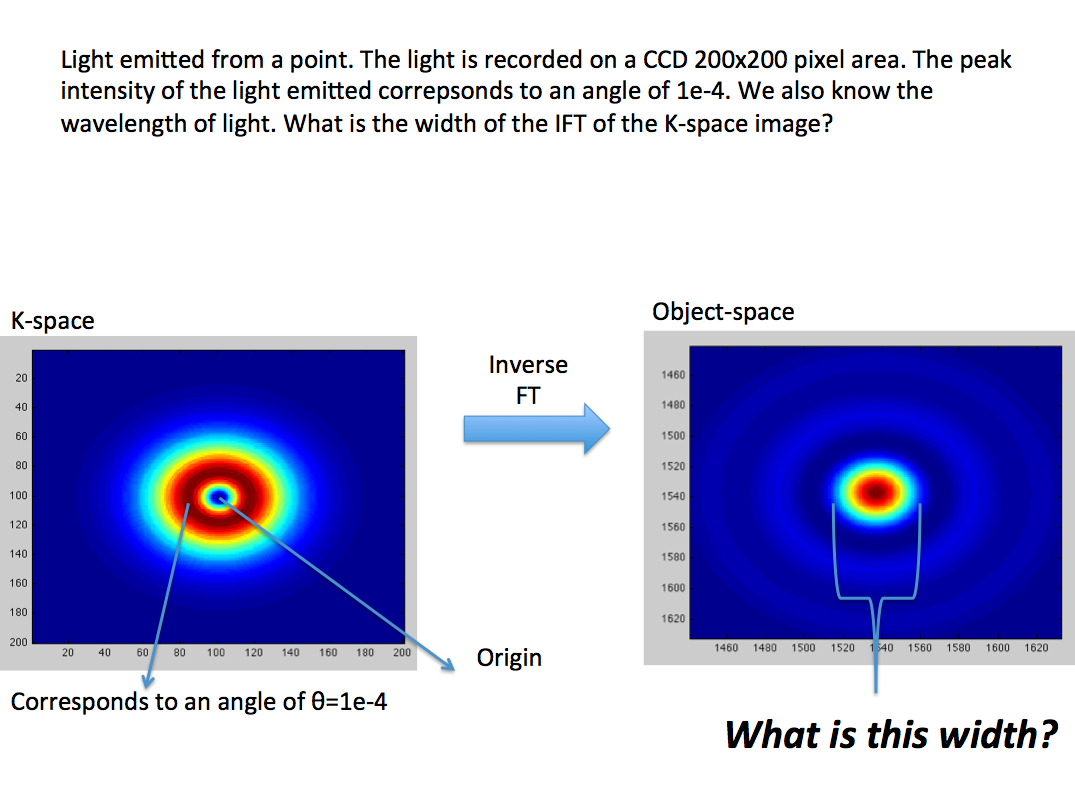
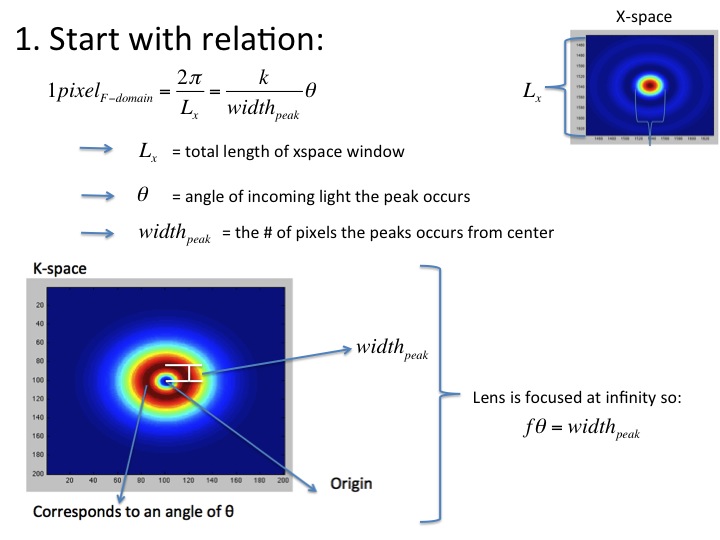
The units of your X-space are the inverse of the units of your K-space. So if your K-space is in $\mathrm{m}^{-1}$, then your X-space will be in $\mathrm{m}$.
To make the full circuit $f(x) \rightarrow F(k) \rightarrow f(x)$ requires an overall normalization factor of $1/2\pi$ to ensure that you get the function you started with. As Chris White points out in his comment, there are a few different conventions on where exactly to put this normalization factor. Some put it entirely on one of the transformations. Some conventions split it between the two transforms, and put $1/\sqrt{2\pi}$ on each integral; this has the advantage of making the Fourier transform and the inverse Fourier transform perfectly symmetrical with respect to $x$ and $k$.
In addition, some conventions for wavenumber define it as cycles per unit distance (so that $xk = 1$), while some define wavenumber as radians per unit distance (so that $xk = 2\pi$).
Ultimately, you might need to multiply the axes in your X space by $1, \sqrt{2\pi},$ or $2\pi$, depending on the set of conventions your software is using, and the convention you have used to express your $k$ values. You should already know the latter. For the former, you will have to check the documentation for the Fourier transform in your software.
Ok, so firstly thanks so much for all of your help...
Secondly I have wrote down the solution (ATTACHED PDF) that one of the guys in my group gave me. But to be honest I don't understand the very first relation (in step one).
I specifically don't understand how the width of the peak in pixels fits in? Any guidance?
