Can't understand this equation

Question

We are in phase space of $6N$ dimensions. Each point $ \mathbf r$ in this space has $6N$ coordinates.

Pathria writes

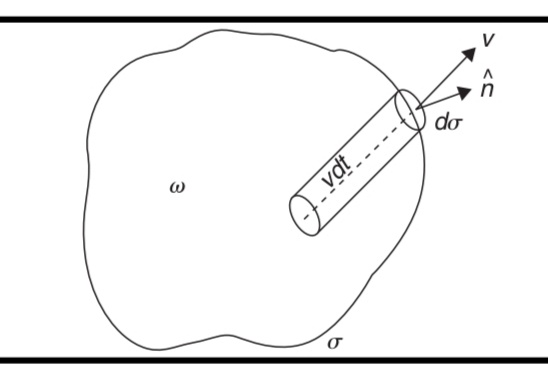
Consider an arbitrary "volume" $\omega$ in the relevant region of the phase space and let the "surface" enclosing this volume be denoted by $\sigma$; then the net rate at which the representative points "flow" out of $\omega$ (across the bounding surface $\sigma$ ) is given by $$ \int \rho \boldsymbol{v} \cdot \hat{\boldsymbol{n}} d \sigma $$

Where $\boldsymbol{v}$ is a generalized velocity and $\rho$ is number density function

I can understand why the equation is true if the velocity is a spatial 3D vector, and the surface is a regular 3D one, however in higher dimensions I'm not sure what the equation means and why it holds. We can't even visualize a surface so what does it mean that representative points flow out of a surface?

Can anyone please explain the equation above.

Thank you

Answer 1

$\rho\vec{v}$ is a (probability) flux density, $\hat{\vec{n}}$ the unit normal to the surface, their scalar product is the outflux per unit surface, integrate and you have the total outflux. So far, so good?

Up until here we have not talked about spatial dimension, because it works in any. And if you say you only understand it in three dimensions, maybe try two and one first to understand the generalisation of the principle.

Answer 2

This is leading up to Liouville's Theorem. It's just an application of the n-dimensional divergence theorem.

If your question is regarding the validity of the expression then PhysSE isn't the right place for this.

Additionally, you don't need to use the divergence theorem to reason through Liouville's Theorem. If our probability density, $\rho$, is generally a function of time as well as the phase space coordinates then,

$$\rho \equiv \rho(t,q_1,p_1,...,q_N,p_N)$$

$$\frac{d\rho}{dt}=\frac{\partial \rho}{\partial t}+\frac{\partial \rho}{\partial q_1}\frac{\partial q_1}{\partial t}+\frac{\partial \rho}{\partial p_1}\frac{\partial p_1}{\partial t} + ... \tag{1}$$

In Gibbs' 1902 book he reasoned that this probability density acts exactly as an incompressible fluid does, or in other words, the rate of change of probability within the phase space will exactly equal the probability flowing out of it:

$$\frac{d \rho}{dt}=0 \tag{2}$$

This is always true, whether or not we are at equilibrium. If our ensemble is complete, then the probability density will integrate to 1 over the phase space:

$$\int ... \int \rho d^{3N}q d^{3N}p=1 \tag{3}$$

Clearly the time derivative of $(3)$ is zero, and so the partial time derivative of $\rho$ must be zero ($\rho$ is a function of time and the phase space so we must change the total derivative to partial when bringing it inside the integral):

$$\frac{\partial \rho}{\partial t} =0$$

Reformulating with Hamiltonian Mechanics, we get, using the Poisson Bracket:

$$\{\rho, H\} = 0$$