What is the centripetal force when a bar rotates about its center of mass?

Question

Scenario 1:

The centripetal force acting on the ball towards the center of rotation is $\frac{mv^2}{r}$, where $m =$ mass of the ball, $v =$ magnitude of the instantaneous linear velocity of the ball, $r=$ distance between the center of mass of the ball and the center of rotation. The source of the centripetal force in this scenario is the man's finger holding onto one end of the string while the other end is attached to the ball.

Scenario 2:

Here, $O$ is the center of mass of a rigid and uniform bar. None of the points on the bar are affixed: it is an unconstrained bar. Here, $|\underline{u}|=|\underline{v}|$. Due to the couple, the bar will rotate about its center of mass. If there's rotation, there must be a centripetal force. What is the centripetal force in this scenario and how can I calculate it?

Edit:

In scenario 2, $\underline{u}$ and $\underline{v}$ are impulsive forces and they constitute a couple. Needless to say, they are applied for an instant.

Answer 1

A way to model the forces in the bar is staying in its non inertial frame. For this frame, the bar is in equilibrium.

In a small slice with length $\Delta x$ at a distance $x$ from the COM, the balance of forces is $F_x - F_{x+\Delta x} + \rho \Delta x \omega^2 x = 0$, where the last term is the fictitious centrifugal force acting in the slice, and the $F$'s are the centripetal forces at each end of the slice.

Dividing by $\Delta x$ and taking the limit when it goes to zero, and knowing that $\omega \frac{l}{2} = v$, where $v$ is the modulus of the velocity of the ends of the bar:

$$\frac{\partial F}{\partial x} = \rho \frac{4v^2}{l^2} x$$

At the ends of the bar ($x = \frac{l}{2}$), there is no forces, because there is no mass to pull to the center. Integrating, we get the expression for the centripetal force:

$$F(x) = \frac{1}{2}\rho v^2(4\frac{x^2}{l^2} - 1)$$

Answer 2

The following was added due to the OP placing a bounty on this question with accompanying discussion.

See the figure below.

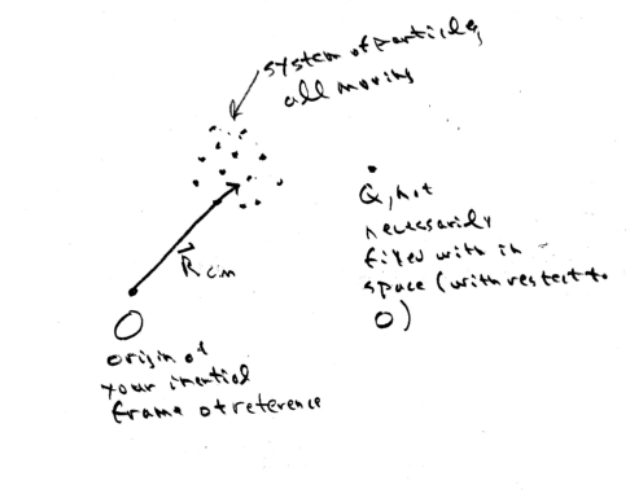
Let $O$ denote the origin of the inertial system you are using to evaluate motion. For a "system of particles" (not necessarily a rigid body), the translational motion of the center of mass is always specified as $\vec F_{ext} = M\vec a_{CM}$ where $\vec F_{ext}$ is the total external force, $M$ is the total mass (sum of mass of all particles) and $\vec a_{CM} = \ddot{\vec R}$ is the acceleration of the center of mass, where $\vec R$ is the position of the center of mass relative to $O$. Torque and angular momentum depend on the point about which they are evaluated, call it $Q$, a point not necessarily fixed in space relative to the fixed origin $O$. If you choose the center of mass as the point $Q$, it is always true that $\vec \tau_{CM} = {d \vec L_{CM} \over dt}$ even if the center of mass is accelerating, where $\vec \tau_{CM}$ is the net external torque for all the particles and $\vec L_{CM}$ is the total angular momentum of all the particles, both evaluated with respect to the center of mass. You can choose any point $Q$ besides the center of mass, but the simple relationship just provided between torque and angular momentum is not true in general.

The textbook Mechanics by Symon discusses all this is detail, and provides the relationship between torque and angular momentum about a general point, not necessarily the center of mass. A rigid bar is a special case of a system of particles for which the distance between any two points in the bar does not change.

For problems where the rigid body is unconstrained in space the point $Q$ is typically taken as the center of mass; (for problems where the rigid body is constrained to rotate about a fixed point, that point is typically taken as $Q$). [Symon, Mechanics]

For your problem, you are evaluating the motion of a uniform rigid bar. You also assume no net force on the bar so evidently you are not considering the force of gravity. For this case the center of mass does not move (it is a fixed point in your inertial coordinate system) so the evaluation of the rotational motion is most easily visualized taking $Q$ as the center of mass.

If the force of gravity is considered in your problem, the translational motion (motion not considering rotation) is described as the motion of center of mass using $M\vec g = M\vec a_{CM}$. In this case the body is not constrained, and it is typical to take $Q$ as the accelerating center of mass for evaluation of the rotational motion using the simple relationship provided earlier. The motion is easily described as translational motion of the center of mass, with rotational motion "about" (with respect to) the center of mass.

So, for your problem, my evaluation is for rotation with respect to the center of mass. Hope this helps!

---

The following discussion takes $v$ as the speed of an end of the bar, since the OP was contrasting the bar motion to that of a ball moving in a circle with speed $v$. But in response to comments/questions by @Bob D and myself, in a recent update to the OP, it is stated that $\vec v$ and $\vec u$ are forces that form a couple; and the forces are supplied only for an instant (after which the angular velocity about the center of mass remains constant since there is no torque about the center of mass once the forces are removed). The discussion below has not been updated, so it assumes $v$ is the speed of an end of the bar after the net force is zero. Also, the distinction between the centripetal and centrifugal forces has, hopefully, been clarified.

Original answer follows

A little more discussion may help understand the result developed earlier by @Claudio Saspinski. Rotation with respect to the center of mass is evaluated. @Claudio Saspinski uses a non-inertial reference frame rotating with the bar, and in this frame the fictitious centrifugal force at a point in the bar acting outward along the bar from the center of mass is equal in magnitude and opposite to the centripetal force acting inward toward the center of mass along the bar to keep the bar stationary (not rotating) in this frame. For an inertial frame (non rotating), there is no centrifugal force, and the only force is the centripetal force acting inward. The centripetal force is the name given to the actual force that causes inward acceleration, here the tension force in the bar. That is, the tension is the centripetal force.

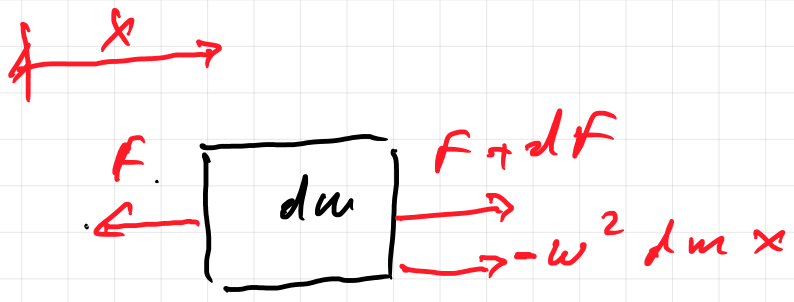
The figure below shows the forces in both the inertial and non-inertial frames, and provides a force balance in the non-inertial frame on a mass element $\Delta m$ where $l$ is the length of the rod and $\rho$ is the linear density (mass per unit length) of the bar. Note that $\Delta m$ is stretched outwards at both ends due to the tension on the bar.

This result obtained by @Claudio Saspinski can be understood as follows.
$$dF(x) = \rho \frac{4v^2}{l^2} x dx$$ is the centrifugal force on mass $dm = \rho dx$ located at position $x$, per the earlier answer. Therefore, the centrifugal force on the total mass within $[-{l \over 2}, x]$ is $$(1) F(x) = \int_{-{l \over 2}}^{x} \rho {4v^2 \over l^2} x dx = \frac{1}{2}\rho v^2(4\frac{x^2}{l^2} - 1)$$ Note that at $x = {-l \over 2}$, $F(x) = 0$ since $[-{l \over 2}, -{l \over 2}]$ contains no mass, and at $x = {l \over 2}$, $F(x) = 0$ since for the mass within $[-{l \over 2}, 0]$ the centrifugal force is in the opposite direction but equal in magnitude to the centrifugal force for the mass within $[ 0, {l \over 2}]$. Note that for ${-l \over 2} < x < {l \over 2}$ the centrifugal force $F(x)$ is in the $-x$ direction, since most of the mass within $[{-l \over 2}, x]$ is to the left of the center of mass. $F(0)$ is the maximum value of $F(x)$, and $F(0)$ is in the $-x$ direction. See the figure below.

The centripetal force is equal in magnitude and opposite in direction to the centrifugal force so $-F(x)$ is the centripetal force (the tension) on the total mass within $[-{l \over 2}, x]$. Note that the centripetal force $-F({l \over 2}) = 0$. That is, there is no net force on the total bar in the inertial frame so there is no motion of the center of mass of the bar.

The centrifugal (and centripetal) force per unit mass is dependent of the location of the unit mass, since the centrifugal force $m\omega ^2 x$ on a mass $m$ depends on the location of the mass $x$. This is different from the force of gravity. For the horizontal bar at rest on a flat surface, the force of gravity on a mass $dm = \rho dx$ equals $\rho gdx$ and is independent of $x$. The force of gravity on a section of the bar within $[-{l \over 2}, x]$ is $\rho g (x + {l \over 2})$, where $\rho (x + {l \over 2})$ is the mass within $[-{l \over 2}, x]$.

Per an earlier request from the OP, the following has been added. Centrifugal force (fictitious force) appears in the non-inertial (accelerating) reference frame attached to the moving bar; not due to any force you see as a fixed observer. It acts on every element of the bar and maintains the bar at rest in the reference frame attached to the bar. To an observer at rest watching the bar rotate, there is no centrifugal force and the body moves due to a centripetal force supplied by tension within the body (electromagnetic forces of chemical bonds); just like the ball on a string in rotation has centripetal force supplied by the tension on the string.

Answer 3

Actually this question is wrongly framed.

For different point in space, the centripetal force computed may be different. The centripetal force is not the 'cause' by rather an alternative expression for net force similar to how we can always write sum of net force = ma_x in one dimensional motion.

The idea is that depending on what point of space you take, the circle which the particles on the rod is spinning about is different.

The simplification one gets from taking the point rotation is considered as the middle of rod itself is that the direction of normal and direction of centripetal force agrees. So, they can directly equate components and solve for tension.

---

One more point, actually you can define centripetal acceleration for particles moving on non circular curves, so the idea that you suggest in the post that "circle curve.. so something centripetal" is not correct.

The idea of extending the idea of centripetal acceleration to general curves is to consider the circle best fitting to the at the point particle is. Now this circle's radius is the radius in centripetal formula, and the velocity is of course the velocity of particle tangent to curve.

This idea is known in Differential Geometry as the osculating circle.

Answer 4

Might as well throw my hat into the ring. Centripetal force is the force, minus any components parallel to velocity, which needs to be exerted on an object so that it moves along some path. It only cares about inertia though, not gravitational force, so it’s best thought of with acceleration. $${\vec{F}}_{Centripetal}=m\left(\ddot{\vec{r}}-\dot{\vec{r}}\left(\frac{\ddot{\vec{r}}\cdot\dot{\vec{r}}}{\left|\dot{\vec{r}}\right|^2}\right)\right)$$ As an example, a mass is tied to the edge of a wheel rolling on a road with constant angular velocity. From the perspective of someone moving at the same velocity as the wheel's center, the mass moves in a circle and the centripetal force has a constant magnitude. From the perspective of someone standing still on the road, the mass moves in a cycloid shape and the centripetal force’s magnitude is 0 when the mass is near the ground and maximum when at the top of the wheel. So centripetal force is coordinate system/frame dependent. Lets now look at the acceleration an object perceives in a rotating reference frame. $${\vec{a}}_r={\vec{a}}_i+{\vec{a}}_{Coriolis}+{\vec{a}}_{Centrifugal}+{\vec{a}}_{Euler}={\vec{a}}_i-2\vec{\omega}\times{\vec{v}}_r-\vec{\omega}\times\left(\vec{\omega}\times\vec{r}\right)-\left(\frac{d\vec{\omega}}{dt}\right)\times\vec{r}$$ where ${\vec{a}}_i$ is the inertial acceleration, $\vec{\omega}$ is the angular velocity vector, $\vec{r}$ is the position coordinate with respect to the rotating reference frame, and ${\vec{v}}_r$ is the velocity with respect to the rotating reference frame. Since your bar is rigid, ${\vec{v}}_r=0$. I’m also going to assume the angular velocity vector is perpendicular to the bar, the bar lies entirely along the x-axis in the rotating reference frame, and the angular velocity is only changing in magnitude along the same axis. $${\vec{a}}_r={\vec{a}}_i-2\vec{\omega}\times{\vec{v}}_r-\vec{\omega}\times\left(\vec{\omega}\times\vec{r}\right)-\left(\frac{d\vec{\omega}}{dt}\right)\times\vec{r}={\vec{a}}_i-\omega^2x\hat{z}\times\left(\hat{z}\times\hat{x}\right)-\dot{\omega}x\hat{z}\times\hat{x}={\vec{a}}_i+\omega^2x\hat{x}-\dot{\omega}x\hat{y}$$ We will now calculate the moment of inertia I and use it, along with torque τ to find $\dot{\omega}$. $$\begin{matrix}I=\int_{-\frac{L}{2}}^{\frac{L}{2}}{\frac{Mx^2}{L}dx}=\frac{2ML^2}{12}&\dot{\omega}=\frac{\tau}{I}\\\end{matrix}$$ The centrifugal force density(force per length is this 1D case) is $${\vec{f}}_{Centrifugal}=\frac{M\omega^2x\hat{x}}{L}$$ Meanwhile, the centripetal acceleration in the bar from the perspective of an observer with the same velocity as the bar is $${\vec{a}}_{Centripetal}=-\omega^2r\hat{r}$$ The tension is $$T=\int_{-\frac{L}{2}}^{x}{-{\vec{f}}_{Centrifugal}\cdot\hat{x}dx}=-\frac{M\omega^2}{L}\int_{-\frac{L}{2}}^{x}{xdx}=\frac{M\omega^2}{8L}\left(L^2-4x^2\right)$$ I hope this was informative enough.

Answer 5

I'll throw my hat into the ring too (and hope I don't live to regret it).

Let the bar have length $l$ and mass $m$ and negligible thickness. Given that the couple is applied momentarily (per the edit to the question), we will assume that the bar acquires an angular velocity of $\omega$ as a result of the momentary application of the force couple.

Consider a differential mass $dm$ located on the right side of the bar a distance $r$ from O. Since the bar is uniform, we have

$$dm=\frac{m}{l}dr$$

The differential centripetal force due to $dm$ is then

$$dF_{C}=\frac{(dm)v^2}{r}$$

Substituting angular velocity $\omega$ for linear velocity $v$ where $v=r\omega$

$$dF_{C}=\frac{(dm)r^{2}\omega^{2}}r=(dm)r\omega^{2}$$

Substituting for $dm$

$$dF_{C}=\frac{m}{l}\omega^{2}rdr$$

The centripetal force on the right side of the bar is then

$$F_{C}=\frac{m}{l}\omega^{2}\int_0^{l/2}rdr=\frac{m\omega^{2}l}{8}$$

where $F_{C}$ acts to the left on point O.

The centripetal force on the left side of the bar is equal in magnitude to that on the right, but acts towards the right on point O, for a net force of zero at point O. Considering the entire bar as a system, the centripetal forces are internal to the system, so the net external force on the system remains zero. The source of the centripetal force is the tension in the bar.

Hope this helps.

Answer 6

The "centripetal force" on a small mass element is

$$F+dF-F-dm\,\omega^2\,x=0\quad\Rightarrow\\dF=dm\,\omega^2 \,x\\ \text{with}\quad dm=A(x)\,\rho\,dx=A\,\rho\,dx $$

you obtain

$$F=A\,\rho\,\omega^2\int x\,dx=\frac 12 A\,\rho\,\omega^2\,x^2+c\\ \text{with}\quad F(L/2)=0\quad\Rightarrow c=-\frac 18\,A\rho\,{\omega}^{2}L^2$$

thus $$F(x)=\frac 12\,A\,\rho\,\omega^2\left(x^2-\frac{L^2}{4}\right) \quad, 0\le x\le \frac L2$$

you can obtain the angular velocity $~\omega~$ from the EOM

$$I_{\text{CM}}\,\dot\omega=\tau\quad,I_{\text{CM}}=\frac{m\,L^2}{12}$$