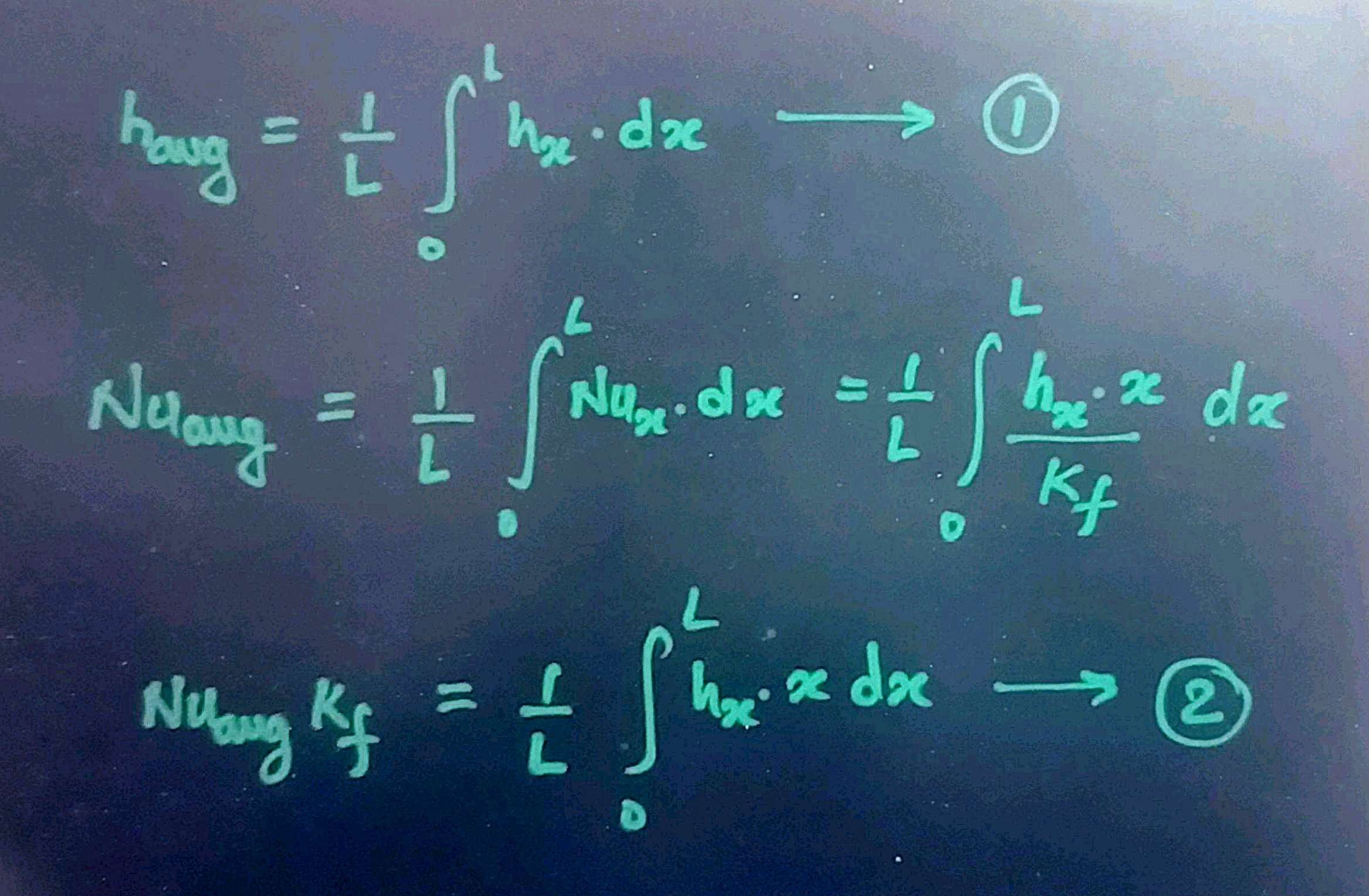Average Nusselt number and heat transfer coefficient

Question

For the flow over a flat plate of length L, how the average Nusselt Number over a length L is equal to

$$Nu_{avg} = \frac{h_{avg}L}{k_f}$$

where $h_{avg}$ is the average heat transfer coefficient over the length L.

What I'm essentially interested in knowing is how Average heat transfer coefficient gives average Nu, where the average is done over a length L.

---

My working:

The local Nusselt number at any x is given as,

$$Nu_{x} = \frac{h_{x}x}{k_f}$$

The average heat transfer coefficient over the length L will be,

$$h_{avg} = \frac{h_1 + h_2 + h_3 + .....+ h_n}{n} | ---------(1)$$

The average Nusselt Number over the length L,

$$Nu_{avg} = \frac{Nu_1 + Nu_2+ Nu_3 +....+ Nu_n}{n} $$

$$Nu_{avg} = \frac{\frac{h_1 x_1}{k_f} + \frac{h_2 x_2}{k_f}+\frac{h_2 x_2}{k_f}+....+ \frac{h_n x_n}{k_f}}{n} $$

$$Nu_{avg} k_f = \frac{{h_1 x_1} + {h_2 x_2}+h_2 x_2+....+ h_n x_n}{n} |---------(2)$$

How to use (2) and (1) to come up with relation

$$Nu_{avg} = \frac{h_{avg}L}{k_f}$$ ?

Another way is to make use of integration

but again how do I combine 1 and 2 to get

$$Nu_{avg} = \frac{h_{avg}L}{k_f}$$