Why do force vectors behave like displacement vectors?
$\begingroup$
$\endgroup$
10
-
5$\begingroup$ If you apply both a vertical force and a horizontal to a stationary object what direction would you expect it to move? $\endgroup$– By SymmetryCommented Mar 8, 2022 at 12:30
-
1$\begingroup$ Which physical facts about forces are we allowed to assume? $\endgroup$– J.G.Commented Mar 8, 2022 at 12:34
-
1$\begingroup$ why not? ....... $\endgroup$– Andrew SteaneCommented Mar 8, 2022 at 12:41
-
$\begingroup$ @BySymmetry Diagonally but why? $\endgroup$– user324713Commented Mar 8, 2022 at 13:00
-
4$\begingroup$ Vector addition is counterintuitive because you are not used to it. After using it for a while, it becomes very natural. $\endgroup$– mmesser314Commented Mar 8, 2022 at 13:18
|
Show 5 more comments
7 Answers
$\begingroup$
$\endgroup$
7
Why does a force in vertical and horizontal direction cause a diagonal force?
I read your question as "Why can force be described as a vector?"
The three Newton's laws of motion cannot be derived from a more fundamental set of (natural) laws. They are set of laws first formalized by Isaac Newton* which he deduced from a number of observations (experimental results) available to him at the time, many of which done by other scholars (Galileo, Kepler, Brahe etc.). Many scientists after Newton confirmed validity of the laws of motion by doing even more experiments.
The answer to why can force be described as a vector is simply because experiments show that behavior. We have never observed a behavior which would prove otherwise. Maybe we will in the future, but chances for such a thing are infinitesimally small.
Direct answer to "why can forces be added" is because forces can be described as vectors. Mathematics tells us that vectors have magnitude and direction, and can be added or subtracted. Therefore, you can always write a force as a sum of two (or more) other forces. In physics we often use horizontal and vertical pair of (orthogonal) axes for convenience, and each force can be written as a linear combination (sum) of horizontal and vertical vectors.
*Isaac Newton was not the first one to discuss what is now known as "Newton's laws of motion". According to some sources (see Wiki article), Galileo Galilei stated the law of inertia before Newton and possibly others have stated it even before Galileo Galilei. However, Newton was the first one to formalize all three laws in his masterpiece "Philosophiae Naturalis Principia Mathematica" from 1687.
answered Mar 8, 2022 at 13:01
-
$\begingroup$
Why can force be described as a vector?actually, I don't think it was his question. AFAIK, He has confusion on vector rather than force. He asks "why addition of two vector shows diagonally where one is along the 'x' axis(means y=0) and another one is along 'y' axis(x=0)?" $\endgroup$ Commented Mar 8, 2022 at 13:41 -
$\begingroup$ @BillyIstiak The OP's question is not very well specified (explained), but you could easily be right. I assumed that people usually do not have problems with grasping vector addition, but are puzzled about forces on a more fundamental level. $\endgroup$ Commented Mar 8, 2022 at 13:45
-
1$\begingroup$ @BillyIstiak, Maybe the OP did not know that they were asking why force can be described as a vector, but that effectively is what they were asking. Forces have direction and magnitude, and if it makes sense to decompose an arbitrary direction and magnitude into linearly independent "horizontal" and "vertical" magnitudes, then that's pretty much what vectors are all about. $\endgroup$ Commented Mar 8, 2022 at 15:49
-
1$\begingroup$ The law of inertia, Newton's first law, wasn't first stated by Galileo. It was first stated by Avicenna in the 11C building on previous work by Philoponus in the 6th C. And then to Buridan and Oresme and even one of Galileo's compatriots, Benedetti. Galileo, being learn'd, was well aware of the work of his predecessors. $\endgroup$ Commented Mar 8, 2022 at 17:50
-
$\begingroup$ @MoziburUllah Thanks, my intention was not to explicitly state who was first. I edited my answer to make this clearer. $\endgroup$ Commented Mar 8, 2022 at 17:52
$\begingroup$
$\endgroup$
2
Imagine I apply a force horizontally to an object and then stop, the object will be moving in that direction.
Then after that, I apply a separate force vertically this will cause an acceleration in the vertical meaning the object will be the superposition of the velocities.
Aka a diagonal trajectory
Now imagine I just apply the forces at the same time, the super position of the 2 forces will point in the direction of the velocity of the 2 forces applies seperately. Its pretty intuitive imo
answered Mar 8, 2022 at 12:47
-
$\begingroup$ Intuition is based on our previous experiences. Only the experimental evidence may tell us if forces behave like vectors or differently. Also the additivity you are using in your argument is based on experimental facts and it is far from intuitive. $\endgroup$ Commented Mar 8, 2022 at 14:50
-
1$\begingroup$ @GiorgioP: This is more or less how I imagine force to act and it is pretty intuitive from our daily experience which informs our intuition. I'd also point out that the kinematic/dynamic law was recognised long ago in the peripetic school in the work Mechanica by pseudo-Aristotle. $\endgroup$ Commented Mar 8, 2022 at 17:42
$\begingroup$
$\endgroup$
I think the other answers don't talk about why it is counterintuitive, so this one will.
First, we must see what we found intuitive, before we were taught about Newton's laws and vectors in school.
An common situation is to push a ball forward. After it has traveled a bit, we may kick it to the left, or even grab it and throw it to the left. What we don't notice is, our foot/hand not only pushes the ball left, it is also pushing it backwards. We observe that the ball stops moving forward, and stars moving leftwards.
So from that we create our intuition: that when you inflict a force on an object, it will stop moving in whatever direction it had, and will start moving only in the direction you forced it.
But this is incorrect, because we don't realize that our hand or our foot are actually pushing the object diagonally, positively in the new direction and negatively in the old direction.
When we learn about vectors and Newton's laws, they seem counterintuitive because they challenge our naive notion that forces first stop an object, and then push it in a new direction. In reality, forces simply give the object an extra vector component, which is added and then results in a new net direction.
$\begingroup$
$\endgroup$
I found this idea a bit tricky myself and had it explained to me like this:
If the forces are opposed, they (somewhat) cancel out.
If the forces are aligned, they add together.
When the forces are at some angle, some component of the forces are aligned and some are opposed.
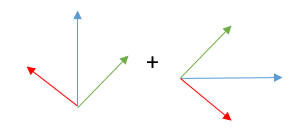
We add the aligned components and cancel the opposed.
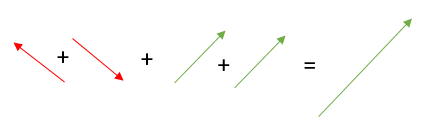
The result is the same as if we just performed vector addition.
$\begingroup$
$\endgroup$
Forces are measured by devices as load cells, that work by measuring small displacements. That displacements can be analized using geometry, as projecting them on orthogonal axis.
The relation $F = k\Delta x$ is the bridge that links forces to geometry. Vectors (at least in this introductory level) are geometric entities.
If for example, a ball is in a corner of a (instrumented and frictionless) cubic box, and pressed with a given angular orientation against this corner, the $\Delta x, \Delta y, \Delta z$ measured by the box load cells must compose a combined displacement to equalize the readings of the device ($\Delta r$) that is pressing the object. And the geometric rule in this case is the Pythagorean theorem.
answered Mar 9, 2022 at 0:08
$\begingroup$
$\endgroup$
You may solve the Newton equation with both forces and you will obtain a diagonal displacement expressed via the force vectors with the same coefficients. This is equivalent to using the sum of forces from the very beginning.
$\begingroup$
$\endgroup$
By Newton's second law, force is the cause of acceleration, which in turn leads to displacement in due course of time. Now since displacement is the very definition of how vectors behave (in some sense), therefore force must also have a vectorial nature.
