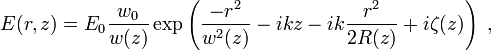1- The structure emitting the energy is doing so at a rate that repeats every 780nm. It's just a fundamental aspect of how the radiation is emitted. And yes it does have to do with the substance doing the emission. Different substances can be used to make different wavelengths or frequencies or colors (if you can see them).
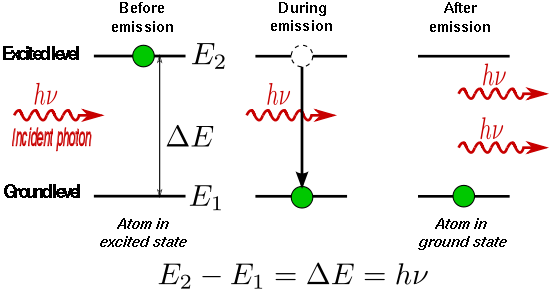
In a laser (not a free electron type) the structure or atoms emitting photons do so at specific frequencies (or wavelengths -- same thing) depending on the orbital positions of the electrons. Where these electrons are and which levels they change to determine the frequency at which the photon is emitted. Stimulated emission is what happens in a laser to generate light and the process looks like:
The frequency is a physically determined by the atoms and the type of emission they undergo. Don't get caught up in the idea that wavelength is a distance, it really is better thought of as how far a wave goes before it repeats its phase. Once you see how frequency and wavelength are one in the same thing it might help you.
2- Lasers form a beam of electromagnetic radiation whose transverse electric field and intensity (irradiance) distributions are well approximated by Gaussian functions. Gaussian is a reference to how the energy spreads both in terms of the E field and the power:
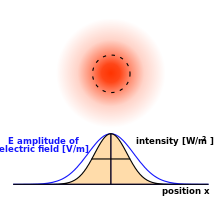
Edit: You can think of them as a sine wave but their properties vary in space like a Gaussian function.
3- Gaussian beams have a number of different propagation models but there are ways to transform them into a plane wave. Perhaps someone on here knows. I don't. I think you've picked up a simplification of the the model. The propagation speed is the speed of light c, but slower if not in free space.
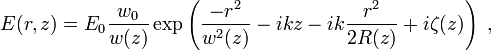
You'll see aspects of frequency, phase, R(z) which is the radius of curvature of the wave front (if not a plane).
r is the radial distance from the center axis of the beam,
z is the axial distance from the beam's narrowest point (the "waist"),
i is the imaginary unit (for which i^2 = -1),
k = 2 \pi/\lambda is the wave number (in radians per meter),
E_0 = |E(0,0)|,
w(z) is the radius at which the field amplitude and intensity drop to 1/e and 1/e2 of their axial values, respectively,
w_0 = w(0) is the waist size,
R(z) is the radius of curvature of the beam's wavefronts, and
\zeta(z) is the Gouy phase shift, an extra contribution to the phase that is seen in Gaussian beams.
Edit: A plane wave is a very specific case of a wave where the wave front appears to not have any directional orientation. It's flat, as in a plane. This is a very special case for lasers as they are often spherical wave fronts defined by R(z) in the above equation. I am not sure that you could shape a laser into a plane wave to be honest. So I also couldn't provide an equation of what that would look like.